20
$\begingroup$

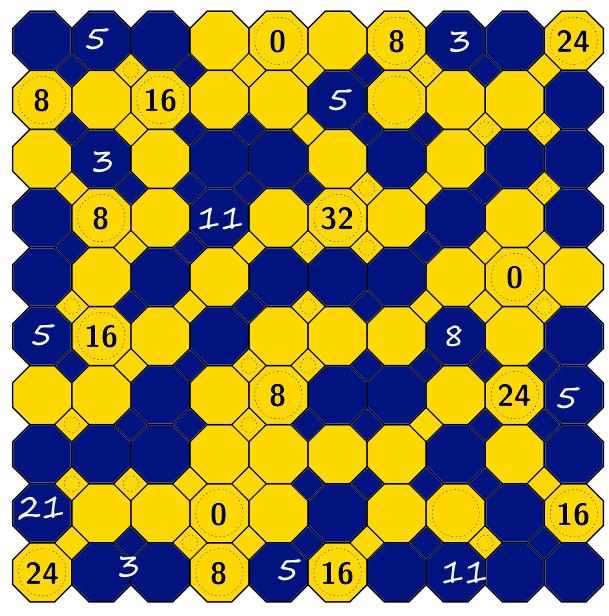
This is a Kurotto puzzle.

Rules of Kurotto:

  • Shade some cells to form connected groups of shaded cells.
  • Circled cells are unshaded. If they have a number in them, that number tells you the sum of the sizes of adjacent groups.

An example Kurotto puzzle (on a square grid), and its unique solution:

enter image description hereenter image description here

(In this puzzle, both squares and octagons can be shaded, and each square or octagon counts as a single cell for the number clues.)

enter image description here

Improve this question
$\endgroup$
3
  • $\begingroup$ Can you tell me why there are two occurrences of a circled cell labelled with a zero being adjacent to an unlabelled circled cell? I would have thought it redundant. If not, I don't fully understand the rules. $\endgroup$
    – theonetruepath
    Commented Oct 23, 2019 at 5:30
  • $\begingroup$ @theonetruepath Yes, those are redundant - they're there for clue symmetry purposes rather than being required to solve the puzzle. $\endgroup$
    – Deusovi ♦
    Commented Oct 23, 2019 at 5:43
  • $\begingroup$ Beautiful puzzle, Deusovi! Incredible! :D $\endgroup$
    – Mr Pie
    Commented Nov 21, 2019 at 6:55

1 Answer 1

Reset to default
12
$\begingroup$

That was fun. Here's the solution:

enter image description here

The key step that revealed how a lot of things had to be was

finding that the 21-group from bottom left had to extend all the way up to the 24-cell at top right.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Great job! Btw how to come up with the deduction of 21-group? $\endgroup$
    – athin
    Commented Oct 23, 2019 at 1:12
  • 2
    $\begingroup$ @athin There is not enough space for the 24 in the top right to not be connected to the 21-group from the bottom left. $\endgroup$
    – w l
    Commented Oct 23, 2019 at 14:32

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.