Some of Cattell IQ Tests really stuck me. So I hope you can help me.
In these two tests, which one is true according to the logic?
And in this one, we find two odd pictures, probably some kind of logic is behind them.
And these tests are like the first one.
In each step, the total distance from the center (sum of distance of each dot) increases by one. Both A and F have the correct total distance. There's also a pattern in the number of dots (5, 6, 5, 6). So the answer is A.
This is an analogy (1 is to 2 as 2 is to 4). The only thing the first two cells have in common is that they are made out of four lines, so since the third has seven lines, the answer is A or E. The second cell contains only circles (geometrical shapes), so the answer we're looking for is E.
B and E, since in all the other ones, it's the same shape across the diagonal (ignoring size, rotation, chirality, fill, line style)
On the two diagonals we can check (top to bottom, left to right, wrapping around), we have the same symbol, but rotated 90 degrees each step. On the two columns we can check, we have the same line style on the entire column.
At first, both A and F seem to work (A is identical to the first cell, F is more coarsely dashed). But if we look carefully, the line in row 1, column 3, has one dash less than the one in row 2, column 1, meaning the two columns have different styles. So we want F, the more coarsely dashed one.
The pattern seems to be in the "black -> white -> black" angle. Top to bottom, the angle increases by a constant amount. The answer is A, the only wider angle.
On each diagonal (top to bottom, right to left), we have the same style (outline, dotted, filled). On each row, left-to-right, you can imagine we're unfolding the shape step by step. So in the hidden bottom-left cell, we had a quarter-circle, which was unfolded into a half-circle, and which we'll unfold again to form a full circle. So either A, C, or E.
Now we have to choose which color to fill it with. The two colors in the first two row are different (dark gray and black), so the most logical choice is a third, different color (light gray). And on top of that, C and E are exactly the same, so if there's only one correct answer, it can't be one of those. So the answer is A.
Reading left-to-right, top-to-bottom, the shapes are rotating clockwise along the edge of the square, one step at the time. C, D, and E all have the shapes in the correct place. Let's see where the long line should be. Keeping track of its position each step from when we last saw it in the third cell, it will end up in the bottom-right corner, so the answer is D.
On each row, we have a shape in the middle cell (circle, triangle). To the left of it, the middle shape with something attached on its left side (square, cross). To the right of it, we have the middle shape, with the attached shape disassembled and placed to its right (so four lines in the first row since a square has four sides).
At first, answers A and C (which are identical) would seem like a good choice, but there are three problems. Firstly, all the other problems had only one correct solution. Secondly, the shapes are aligned to the top of the cell, not the bottom. Thirdly, the lines are curved (not that big of a problem, you could say the attached shape in the first cell was a lens, but it's still something different so a downside). A better choice would be F. The only problem with it is that the square is the white rather than grey, but maybe the missing shape in the bottom-left cell was black, and then there would be a pattern of the color becoming lighter each step.
Some of them seem pretty hard, i will try to add more solutions as i go along, here's what i got so far:
(disclaimer i can't guarantee correctness)
Will edit to add the others
Answers I got so far. Will add more later
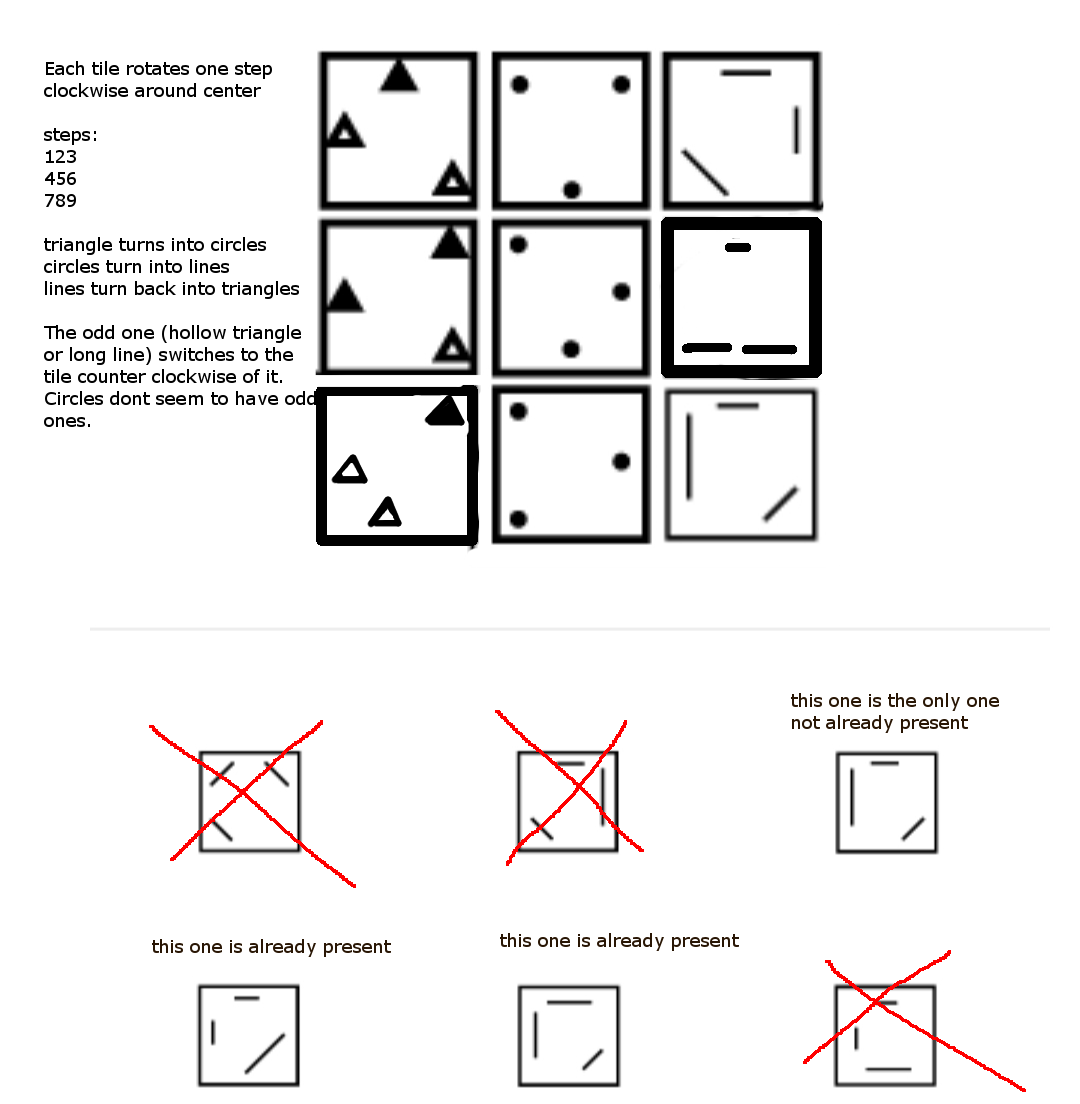
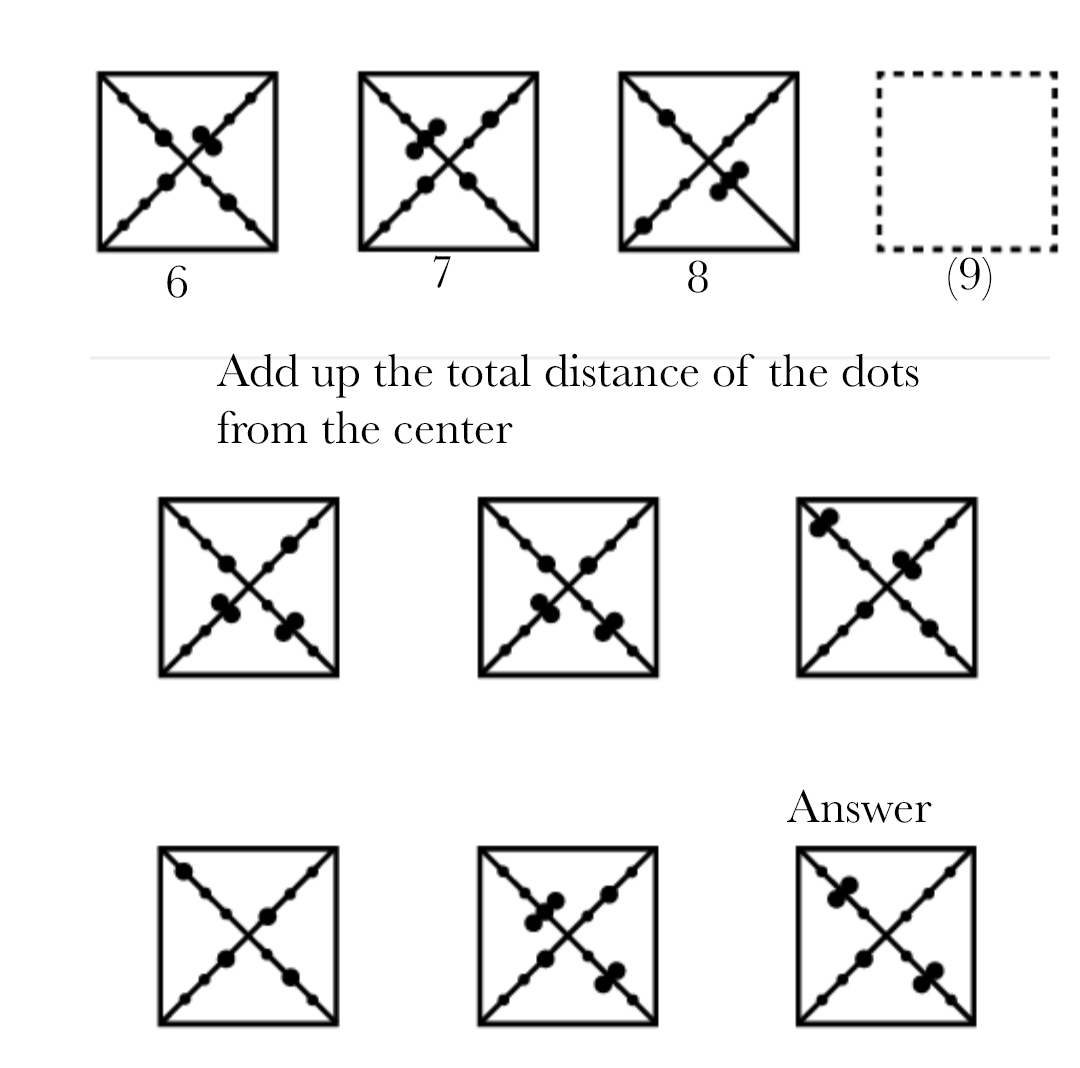
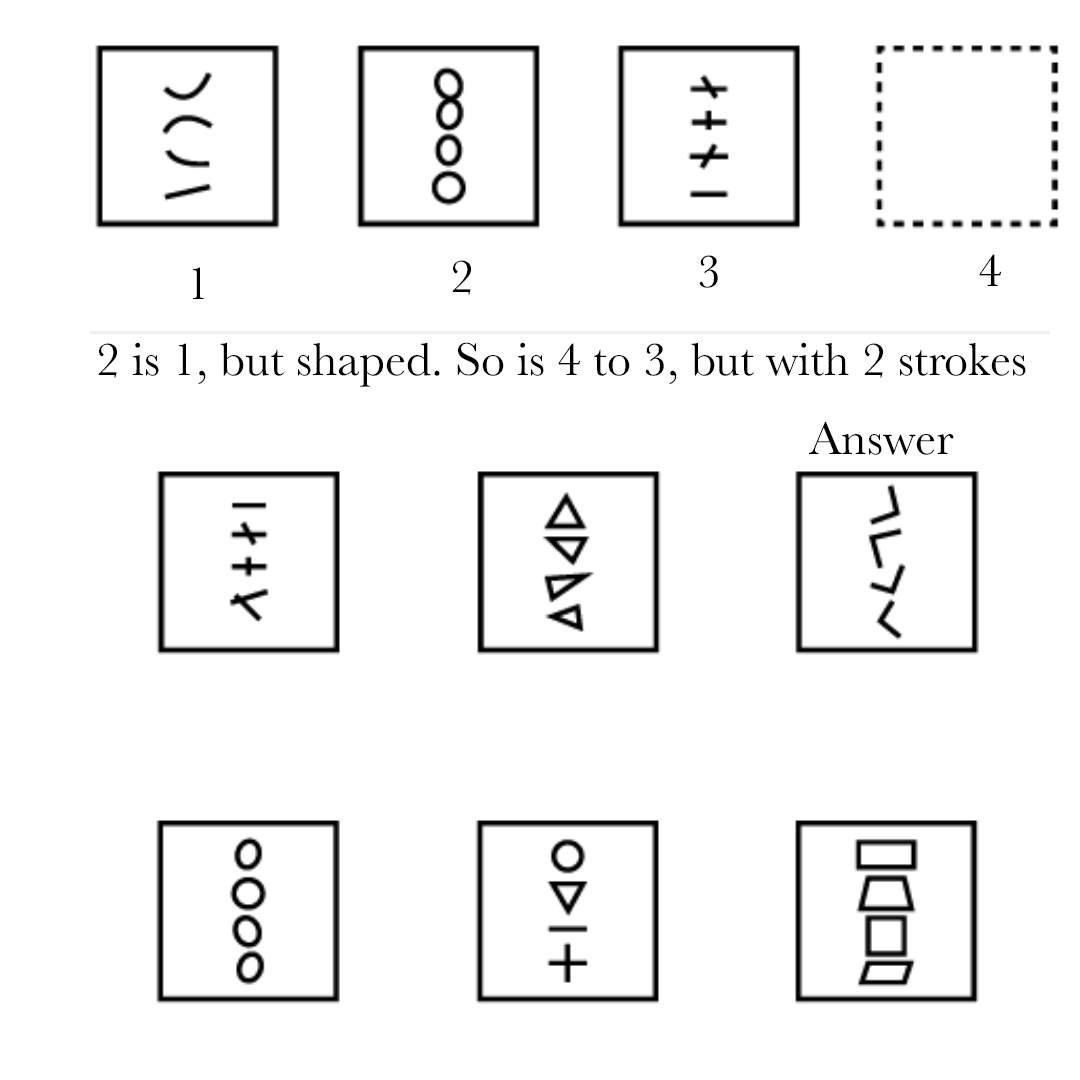
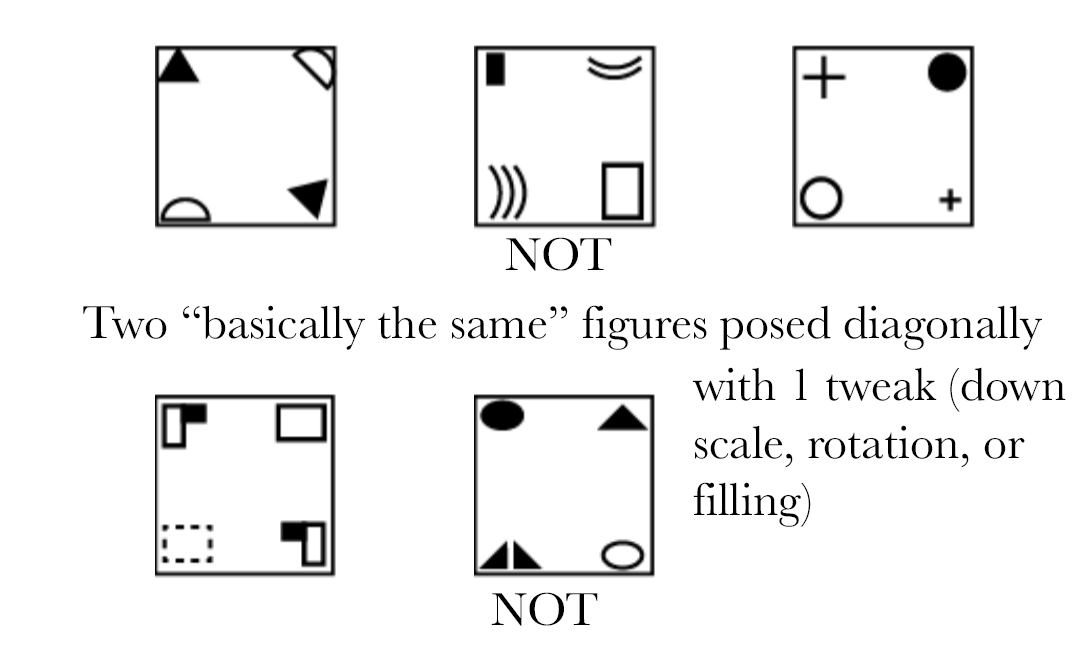
I hope I'm not too late, but I think I may be able to shed some light on all of the questions. SPOILERS AHEAD!
Question 1:
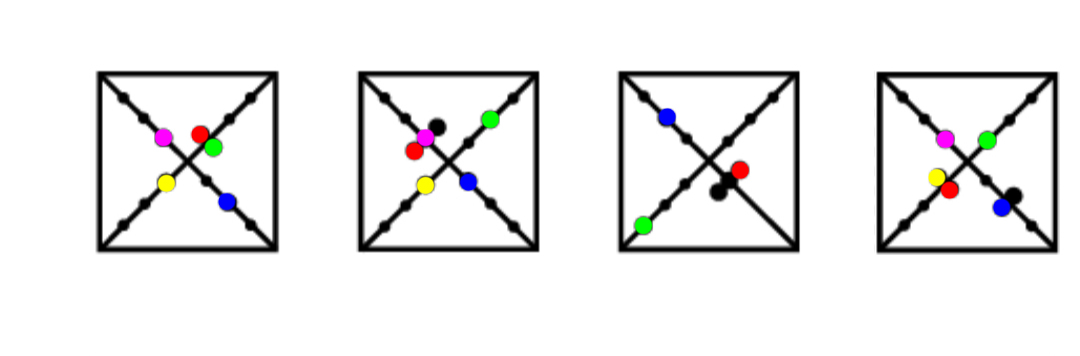
I've colored the beads to make them easier to track. The green bead and blue bead move linearly, first 1 space, then 2 spaces, and then 3. The red bead rotates counter-clockwise 1 position, then 2, and then 3. The purple bead and yellow bead stay put.
Each of the four lines can have, at most, only one position occupied at any given time. If more than 1 position would be occupied at a time, the beads closest to the center disappear.
Finally, each square must alternate between having 5 beads, then 6, then 5, and then 6. If there aren't enough beads already to make that possible, then extra beads, which I've left black, appear somewhere.
Question 2:
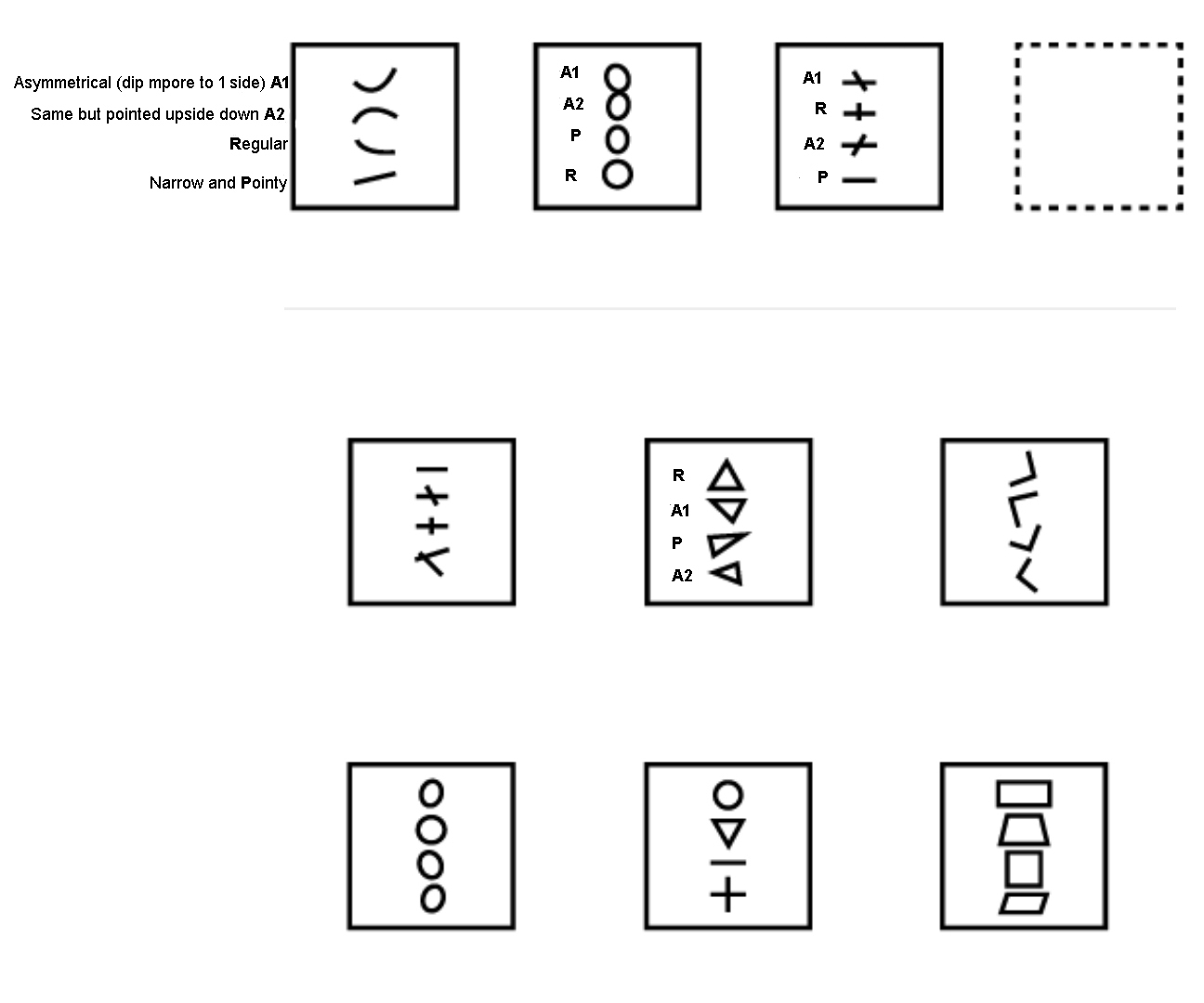
For this one, you've got an asymmetrical piece oriented one way (A1); another asymmetrical piece oriented another way (A2); a narrow, pointy piece (P); and a regular piece (R).
For the first square, you've got an A1 piece with a "dip" nearer one end than the other and pointed upward, an A2 that's similarly asymmetrical but pointed downward, an R piece with its "dip" in the middle, and then a pointy straight line (P).
You have a similar arrangement in the next square. The two ellipses A1 and A2 are technically symmetrical, but they're nevertheless distinguished from each other by pointing in opposite directions whereas the P ellipsis is pointing upward.
In the next square, you got lines with added slants pointing in opposite directions just like the ellipses from earlier.
If we look at the 6 choices, we can eliminate A because the last line with an added slant doesn't mirror the orientation of the other asymmetrical line with a slant. We can eliminate C its shapes are all the same, just oriented differently. We can eliminate D because two of the ellipses are pointing in the same direction. We can eliminate E because they're all completely different shapes. We can also eliminate F because the trapezoid would need to be a parallelogram slanted in the opposite direction of the one at the bottom.
B is the only one that satisfies all conditions. We've got a regular triangle at the top; a long, pointy triangle in the third position; and, finally, two asymmetrical triangles pointing in different directions. One has its longest edge pointing upward, and the other one has its longest edge pointing downward.
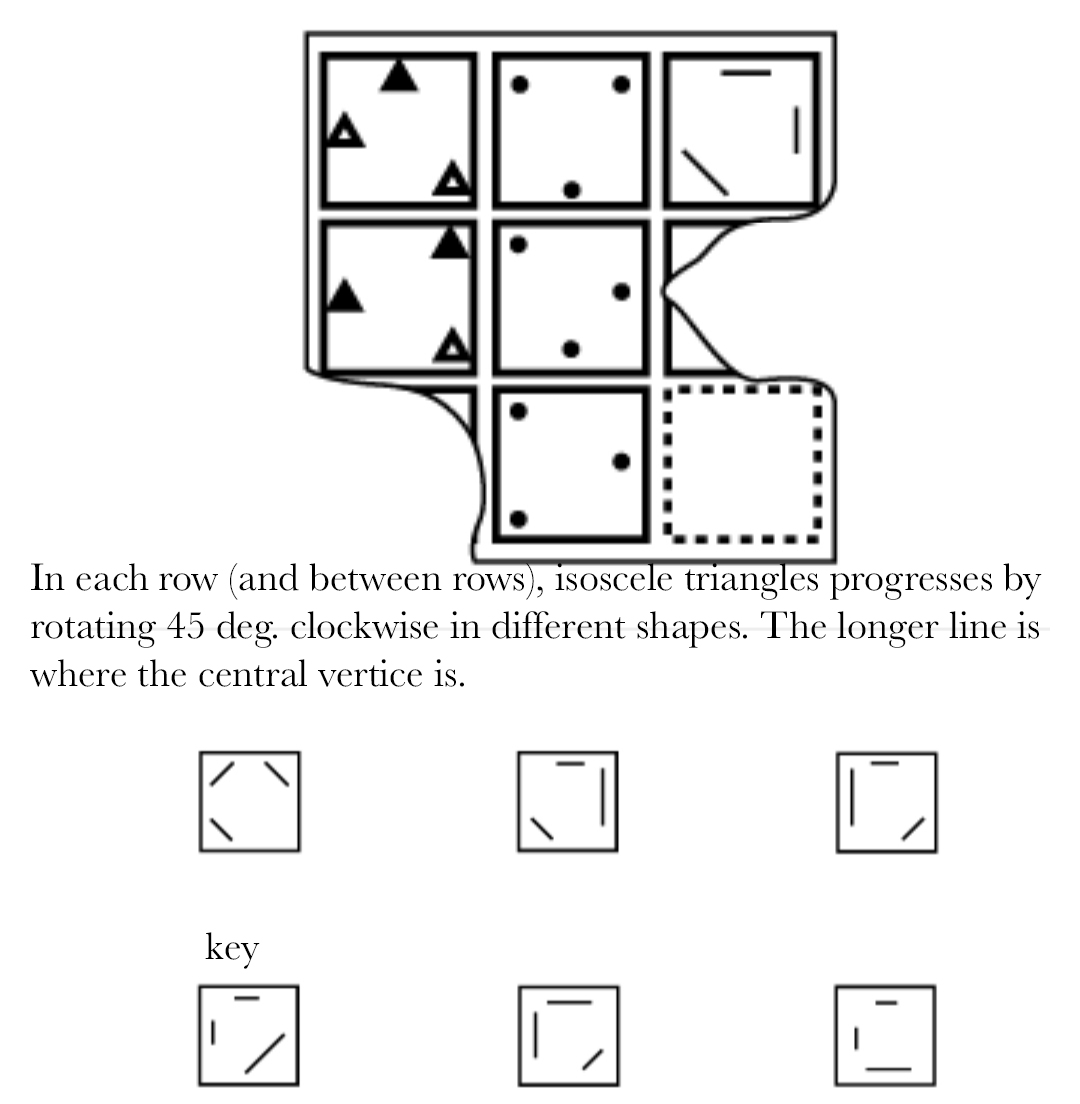
Question 3:
The first square is not like the others because the two different shapes in the upper and lower left change the same by being tilted 45 degrees clockwise whereas all the other shapes change a different way. The second square is out too because the two different shapes on the left undergo two changes instead of one. The top shape changes size AND color. The bottom shape gets rotated AND loses a curve.
Question 4:
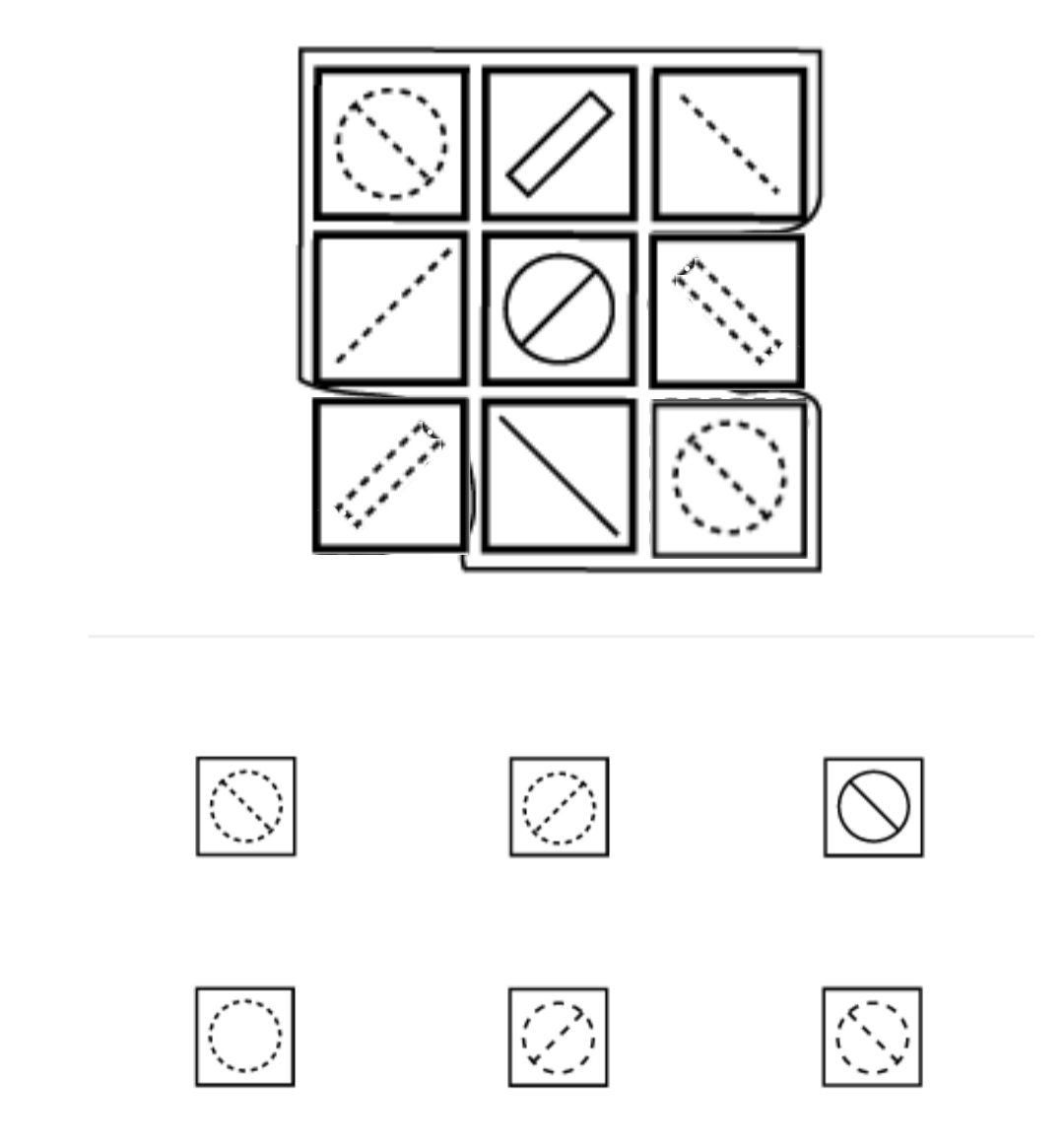
The three different shapes move diagonally. The they alternate between pointing leftward or rightward. There are two dotted version of each shape and a solid one. The "coarser" version is nowhere to be found for the straight line, so A seems more likely than F even though the two lines don't have the exact same number dashes since the one with 8 dashes should really have 6 if it were supposed to be the coarser type.
Question 5:
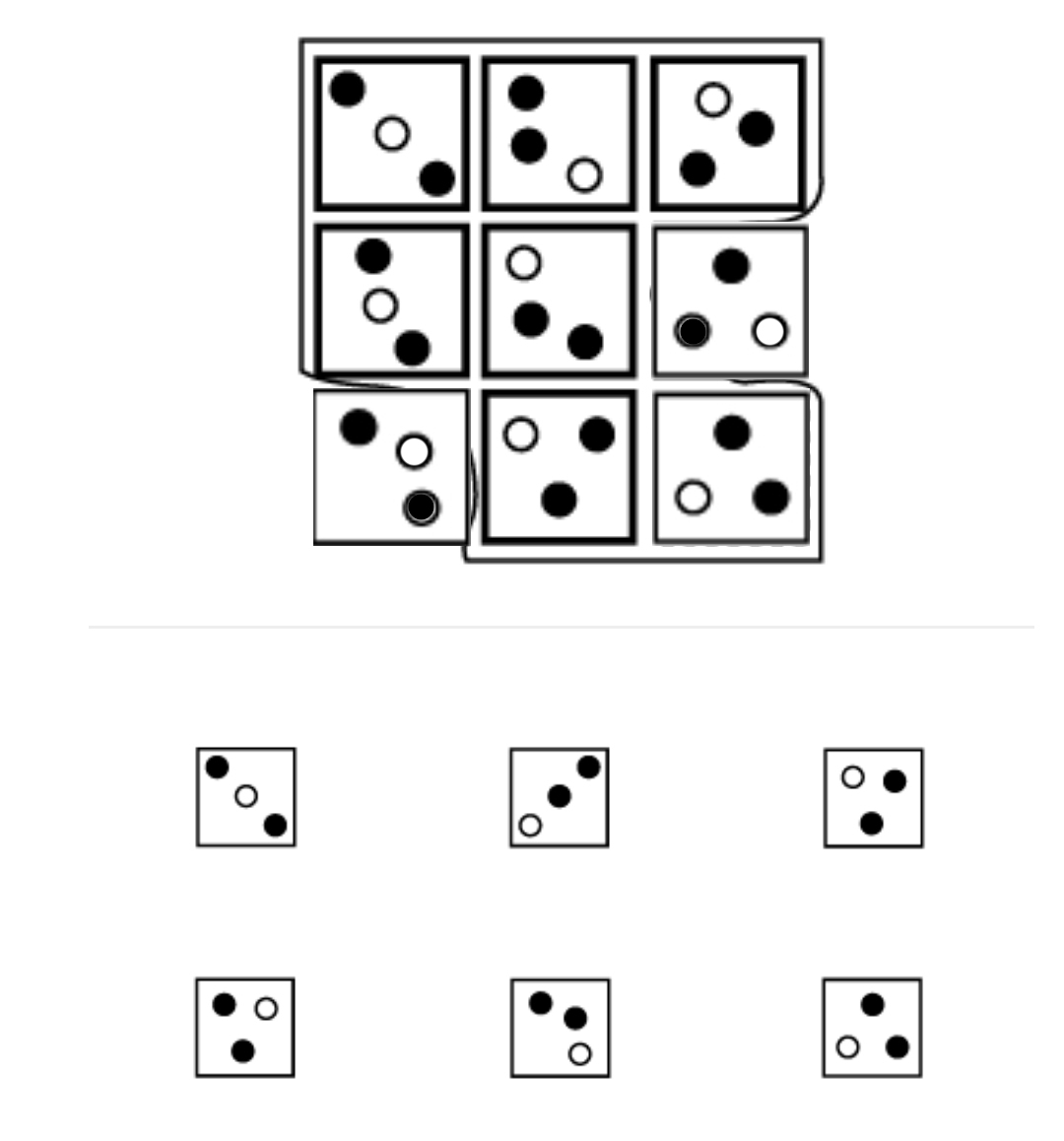
This one was the second hardest. You can think of the 3 circles as a clasp that gets closer to clasping shut in each row. They also rotate as little as possible to get to their final destination (the lower left corner). The color white cycles through them. The cycling is first counter-clockwise and then clockwise.
Knowing this, it's fairly straightforward to fill in the missing square in the middle right, but things get a little tricky one the bottom row because we can't see the square on the lower left. The clasp in the middle left square isn't pointing toward the lower left corner as the successive clasps in the earlier rows are, so, at first, we can't tell if the final clasp should be pointing in the lower left like the others or if it should point in some other place. We also can't see where the white circle is in the first square in the lowest row. But we do know that the final clasp must be triangular since the previous clasp can't be clasped shut any further, so the answer must be triangular too. That leaves us with C, D, and E.
However, since the direction of the cycling changed in the first row, it stands to reason it must alternate back to being counter-clockwise. That eliminates C because the white circle is in the same position. That also eliminates D because that would require the color white cycling clockwise. That only leaves us with E. I've interpreted the lower left to be pointing to the upper right right corner, but it can also be point to the upper left corner provided that the circle closest to the upper right corner is white instead of the middle one. Nevertheless, I think what I put in the picture is more likely as the two out circles start closer to the upper left and lower right corners just like in the previous rows.
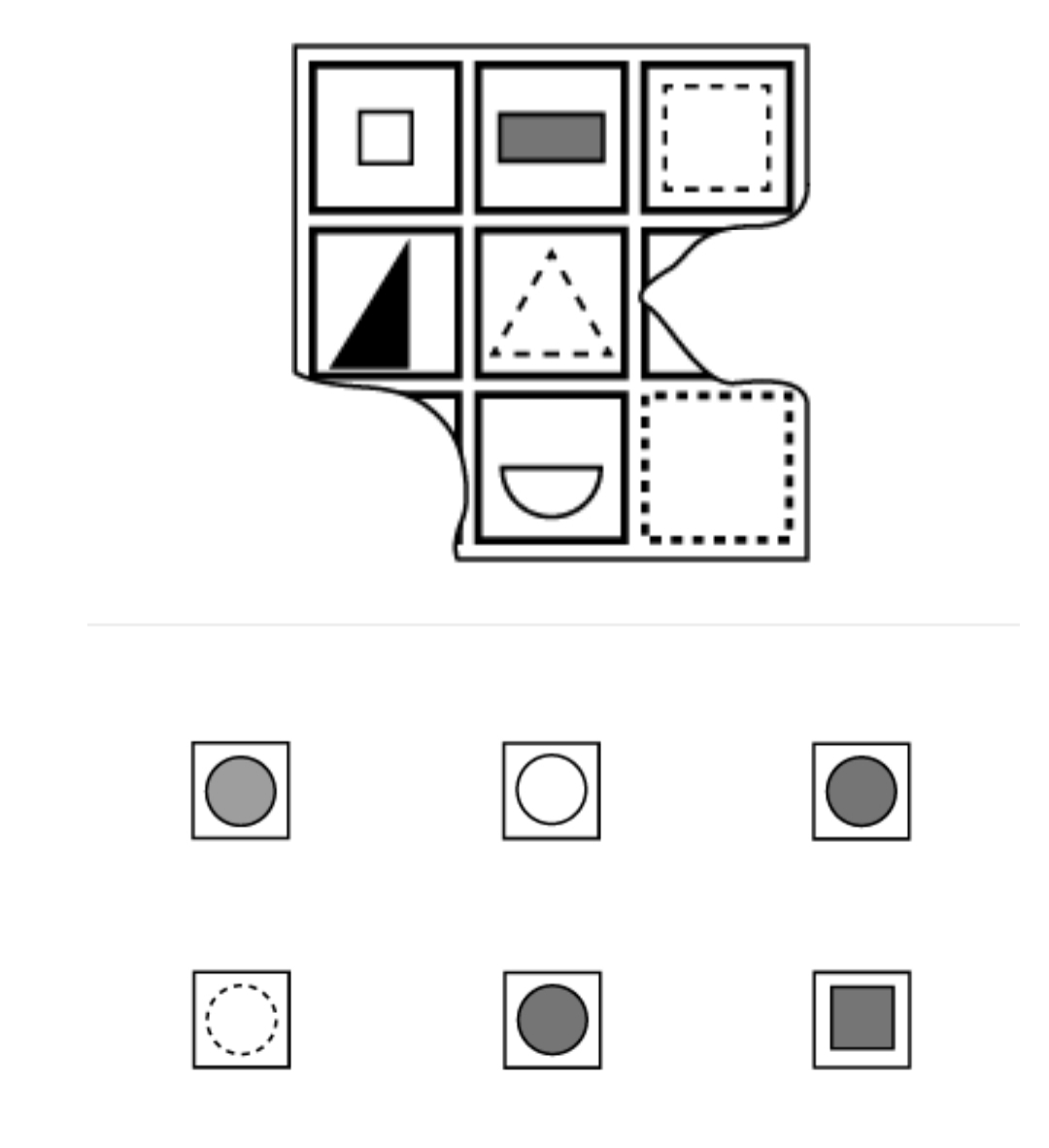
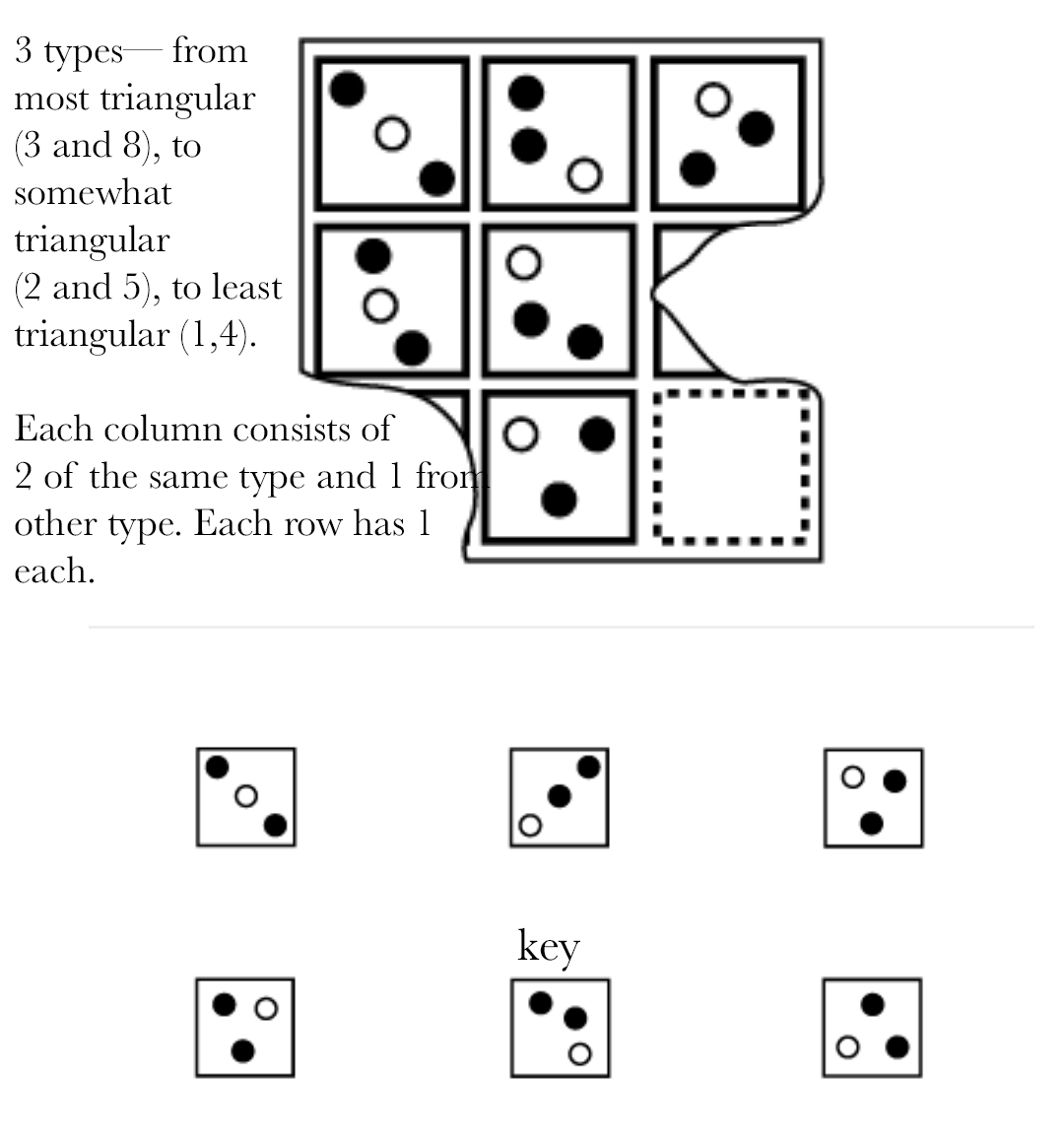
Question 6:
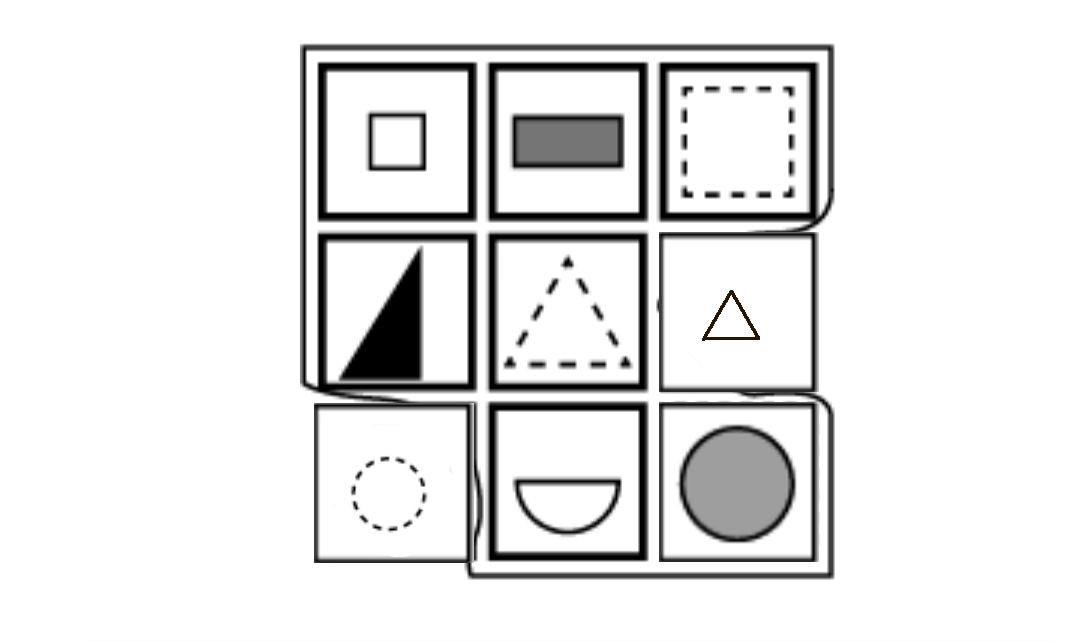Each row has its own basic shape that comes in 3 different variations: a small one, a half one, and a regular one (different variations). Furthermore, one of them is white with a solid border, a white one with a dotted border, and one filled in with a solid color (different "colors"). We can also see that colors don't have a one-to-one correspondence with the variations as evidenced by the small, white square and the white semi-circle. Furthermore, the dotted ones go diagonally as do the 2 other "differently colored" ones.
The only two possibilities for the answer are a solidly colored circle that's either small or normal. There are no small circles to choose from for the answers, so it therefore stands to reason that the circle in the lower right square is a solidly colored normal circle, and the circle in the lower left square is a small dotted one. The lightest gray circle best fits since it's in line with there being a different solid color for each shape.
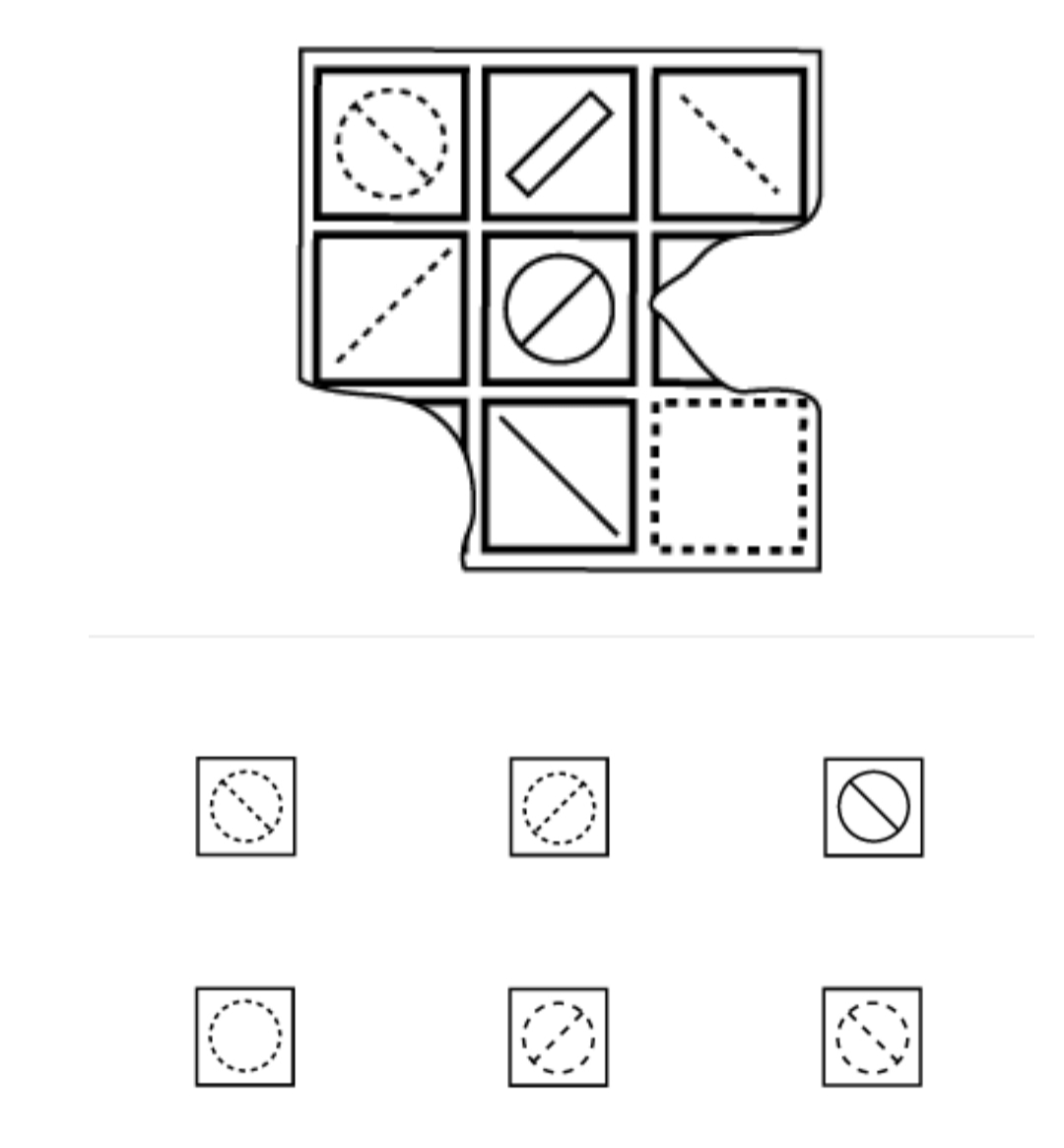
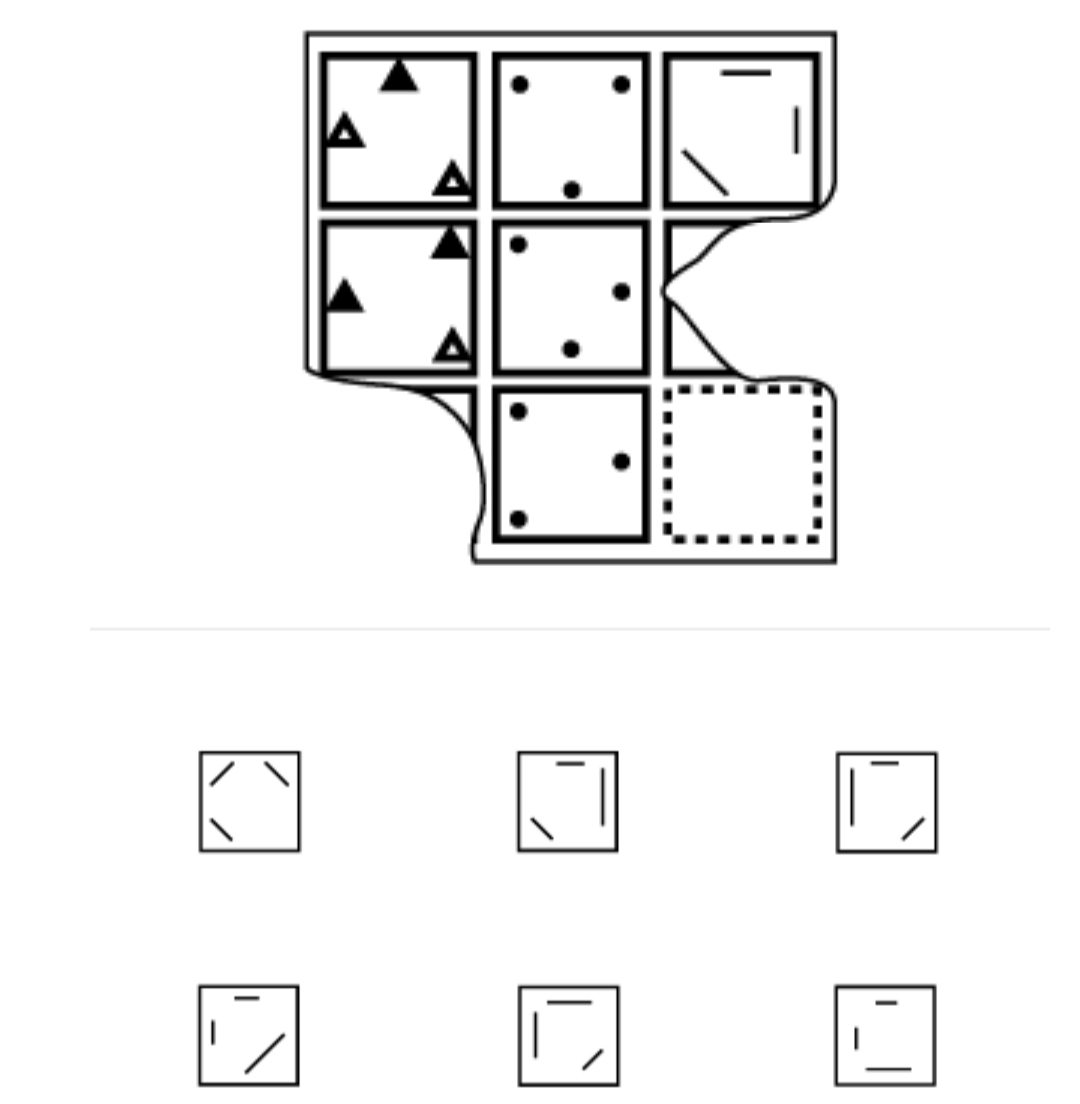
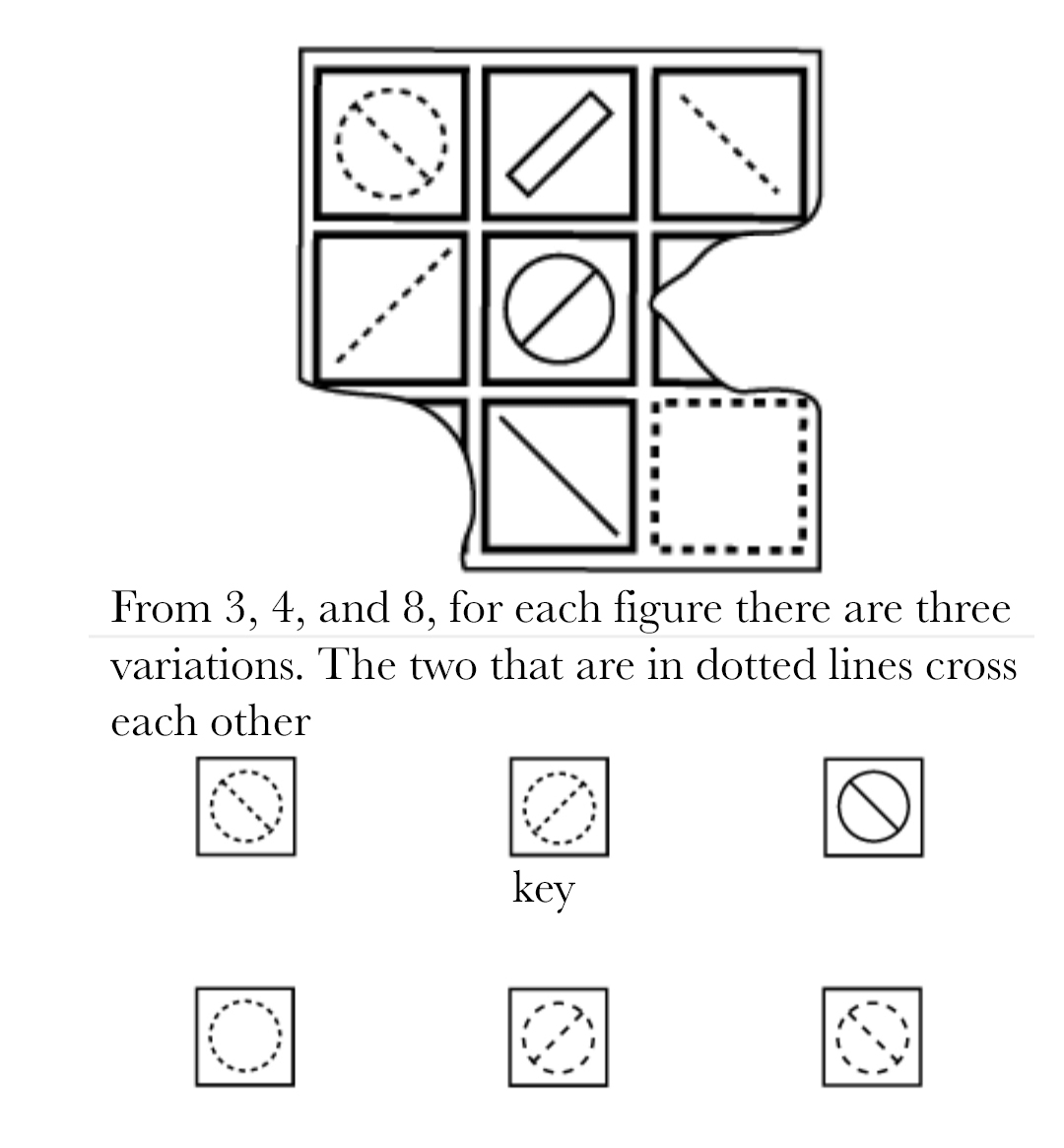
Question 7:
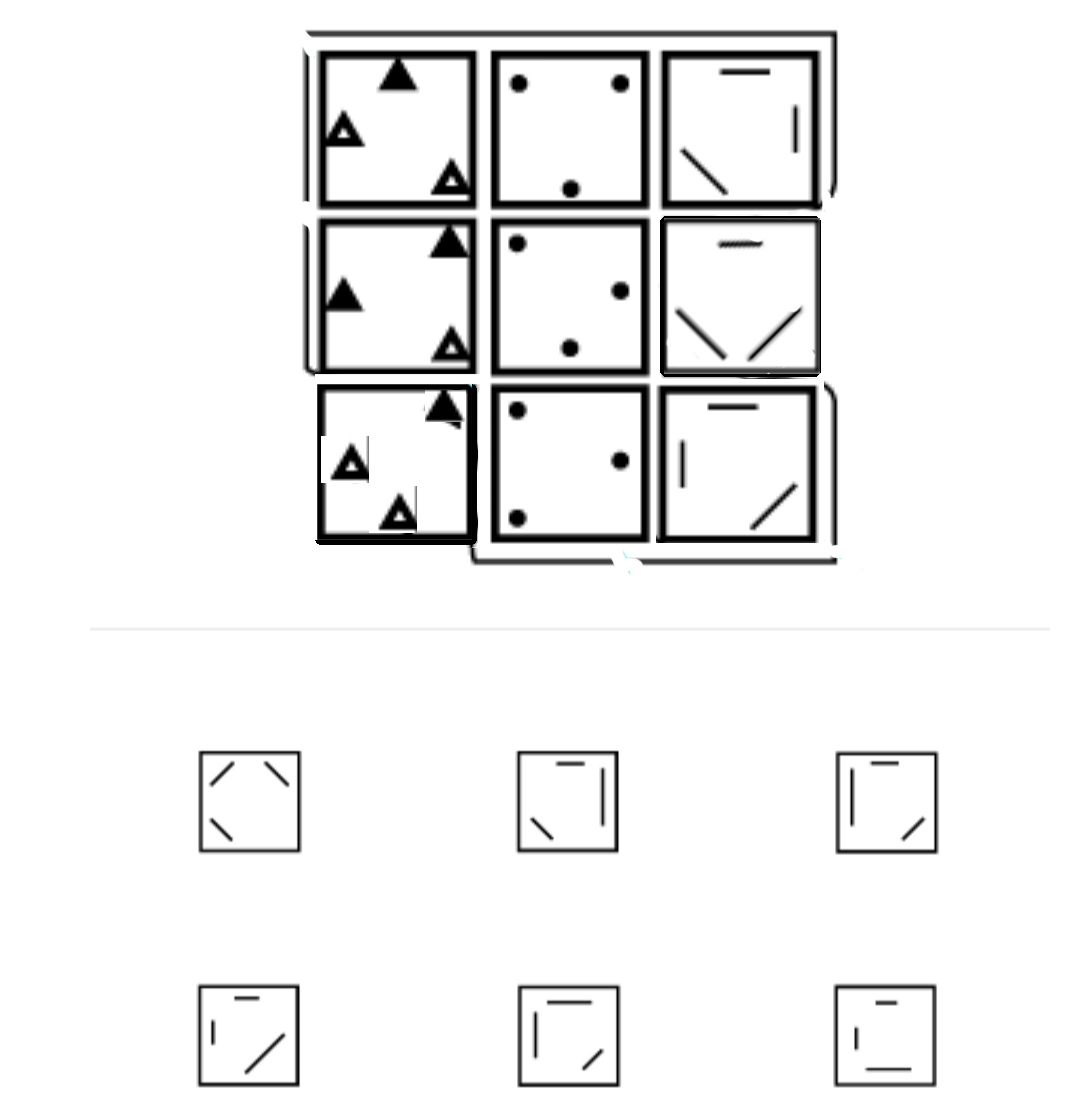This one was the most difficult.
In the first row, we start with 3 triangles, then 3 dots pointing 45 degrees clockwise, and then lines a further 45 degrees clockwise.
We can also see that first set of triangles has two white ones that seem to correspond to the two small lines at the end of the row. Likewise, the black triangle corresponds to big line. Therefore, while the set rotates clockwise, the different attribute cycles counter-clockwise. This is partly obscured by the fact that the dots are always the same.
In the second row, the triangles switch to having the white triangle being the odd one out, and we can see the set has still rotated clockwise, so we can infer that the attribute will still cycle counter-clockwise. Since the more common color for the triangles is black this, however, it stands to reason that being big must be the more common attribute for the lines.
For the third row, since the more common color changed in the second row, it makes sense it would change back in the third row. Working backward from the dots in the middle, the triangle must be pointing in the upper right corner of their square, and the lines must be pointing in the lower right corner. Finally, since being small was the more common attribute in the first, it will change back to that for the final row. That leaves us with C and D.
Now this is where things get really difficult. We know that the different attribute cycles counter-clockwise, but the fact that the dots are all the same makes it difficult to tell where the black triangle must be in the lower left square. Both C and D satisfy the condition that the set rotates clockwise and that the attribute cycles counter-clockwise, so we need to look at what we might have missed in the grid.
If we look at the triangles, we can see it goes from having one side completely white in the first row to having one side completely black in the second row. If we rotate the first triangle so that its "head" lines up with that of the second triangle, we can see that the same side remains having the majority color (i.e. the to the right of the head first has two white and then two blacks). That suggests the odd one out always starts at the same position, and the starting attributes just toggle back and forth for each row. So it's D.
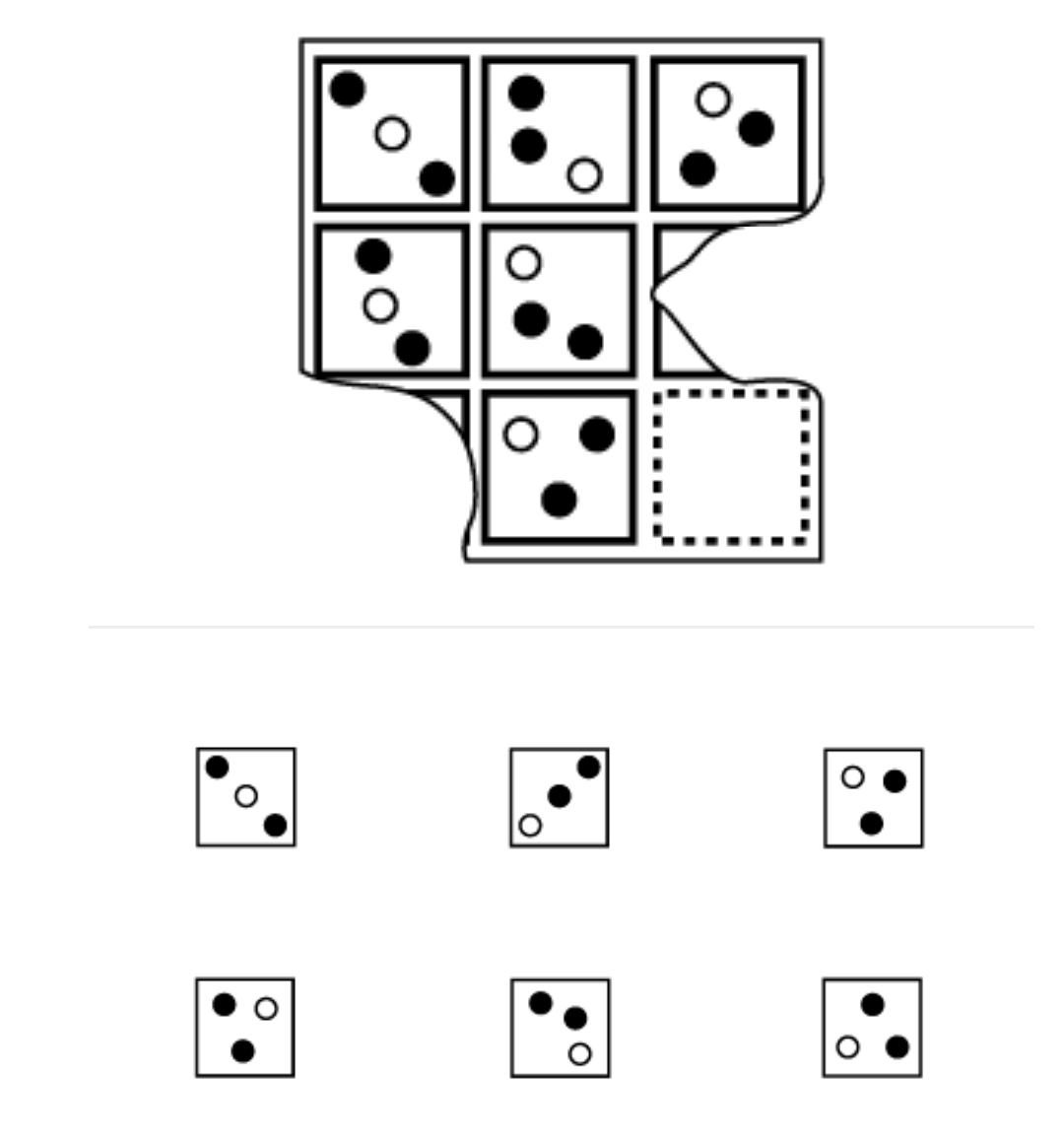
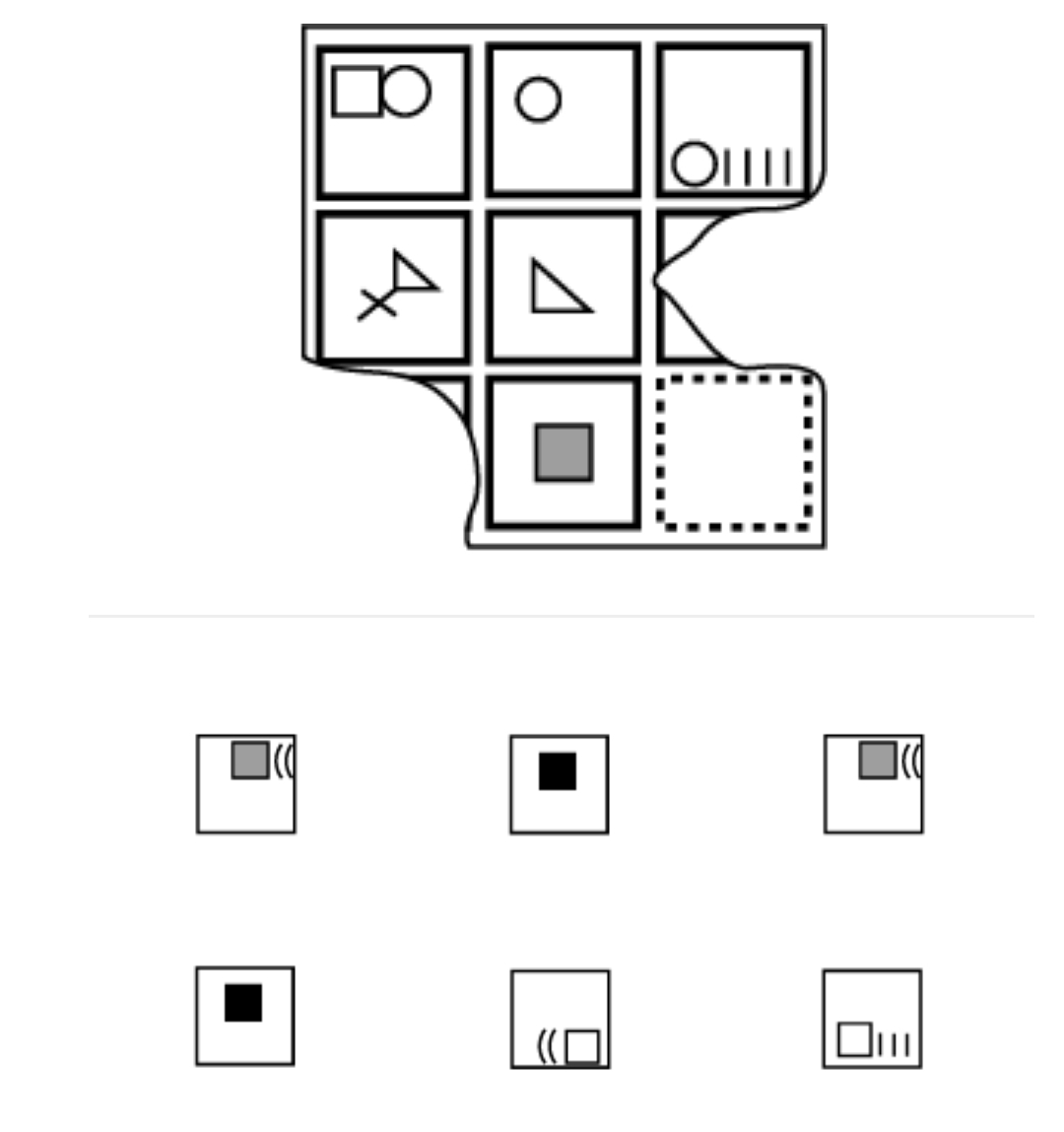
Question 8:
OK, for this one, in the first row, there's a circle to the right of a square, then the circle by itself but smaller, and then the smaller circle down below with the four sides of the square from earlier separated.
In the second, we've got triangles of two different sizes.
The two best candidates thus far are the gray square with the two curves to the right of it or the white square with the three lines to the right of it.
If we pick the white square, we have a problem because there's change happening to the main shape from column 2 to column 3, and the previous two rows show the change occurs in the second column.
Therefore, only the gray box with the curves can be right. Working backwards, we can infer that the first box has is a different color than gray and has an ellipse to its left. Although the first box may be black, it's probably white since a normal-sized, white shape seems to be common to each row.
It would also be in the middle of its grid square since the other shapes do, and that allows for symmetry provided that the last triangle in the second row is a big, white triangle in the middle of its grid square.
So the shape end up at the bottom for the first row, the middle for the second row, and the top for the third row. In addition, undergoes a change unique to each row. In the first row the shape goes from normal to small. In the second row, it goes from small to normal. In the third row, it stays a normal size throughout but changes color.
Ultimately I find it.
is a version of this test and ...
Here at the end of this file you can find the answes ... some of answers are really confusing for example answers to question 2 and 3 that I asked.
But the answer to Question 2 which is B, Question 3 which is A and B, and Question 5 which is F , are nonsense ... Any idea?
Notice that the pictures that you can see in first post may be drawn incorrectly ... please use pdf files to answer my questions .
