3
$\begingroup$

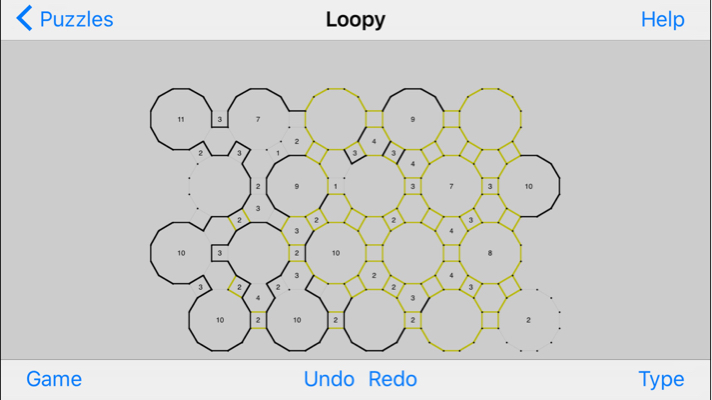
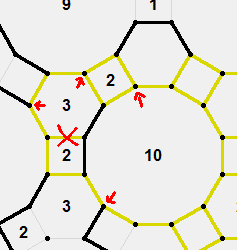
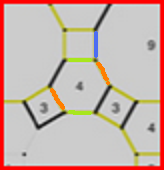
I am stuck on the game Loopy inside of Simon Tatham's Portable Puzzle Collection. I do recommend this puzzle collection, and it's available on several platforms:

The type is 4 X 5 Great Dodecagonal – Hard. The specific game instance is: 5x4t10:B37e94f2329321a1b433374a3bAaA3b432a2Ad2a3c28c3Aa223A32a2a222b4a3a2a. It appears to work on any of the above platforms.

For 3 days now I've been staring at this in brief moments on the bus or just before falling asleep, trying to make progress by pure deduction alone. What am I missing? Or do I have to just start trying things such as connecting two segments and seeing which choices can't be made to work?

Here is my progress so far:

Loopy 4x5 Great Dodecagonal – Hard 5x4t10:B37e94f2329321a1b433374a3bAaA3b432a2Ad2a3c28c3Aa223A32a2a222b4a3a2a

Rules

All the lines start as yellow. You are to make a single unbroken circuit with no intersections. Each number is a clue stating how many of that shape's edges are filled. You win when you make a black circuit that fulfills all the clues perfectly. The yellow lines can be removed as an aid to solving, but do not affect winning.

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ Yellow is how all lines start. You can turn them black or remove them. The numbers tell you how many segments/edges of that shape are filled. You win when you create a single closed loop that fulfills all number clues. Other people figured it out... $\endgroup$
    – ErikE
    Commented Aug 6, 2016 at 18:19
  • 1
    $\begingroup$ Of course you're right, my apologies. I will update the question when I have time. $\endgroup$
    – ErikE
    Commented Aug 6, 2016 at 18:29

2 Answers 2

Reset to default
2
$\begingroup$

Remove the line marked X you forgot to remove. Then use the 3 with the marked corners, then use the 10 with the marked corners. This should get you further a lot.

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ I'm not sure what you mean by "use the 3 with the marked corners." I see that either the top left line and the top right one are solid, or the bottom left line and the top one are solid. Are you saying this implies that the line above the top right 2 is always filled? I get that, but where does the particular focus on the two vertices come from? $\endgroup$
    – ErikE
    Commented Aug 6, 2016 at 23:37
  • $\begingroup$ Yes, that's what I meant. If you like, you can interpret it as "that pair of edges" instead of "that corner". $\endgroup$
    – Anon
    Commented Aug 7, 2016 at 0:48
2
$\begingroup$

From:

loopy

the 4 is either both green or both orange. Either way, the blue line can be filled.

If we fill the lower green line, then we must fill the upper line in the right-most 3-box, and so both orange segments are inaccessible, only leaving the upper green line.

If we fill the lower orange line, then the lower green line is inaccessible, which means that the bottom tip of the left line of the right 3-box must connect to the right line of the same 3-box, and so the top tip of the left line of this 3-box must extend into the orange segment.

Whichever, the upper green/orange line has only one choice - the blue line.

Improve this answer
$\endgroup$
1
  • $\begingroup$ You taught me a new rule. When two segments cannot connect in a particular way (such as the top green connecting to the top right orange, then I can check to see if the adjacent edge (your blue one) is always filled. Thanks! $\endgroup$
    – ErikE
    Commented Aug 6, 2016 at 23:35

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.