Honeydripping around the clock

Question

     

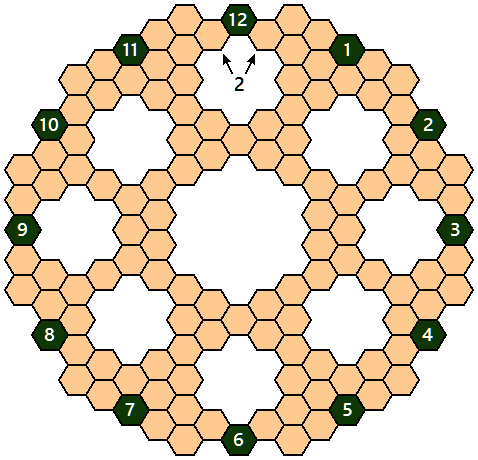
What path could a honeybee follow, beginning and ending at top center, visiting every empty cell exactly once and dripping 2 drops of honey into the last cell?

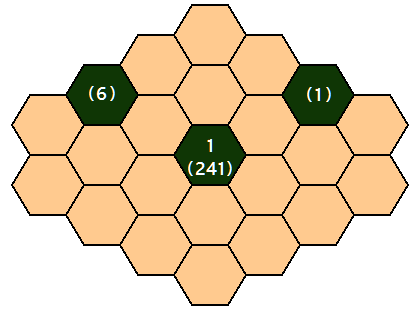
• Start by heading clockwise from the cell at top center, which has 12 honeydrops. Eleven other cells begin with numbers of drops that correspond to hours.

• Each step consists of moving to an adjacent cell and dripping 0, 1, 2 , 3 , 4 , 5 , 6 , 7 , 8 or 9 drops into it based on how many total drops its (1 to 6) neighboring cells, combined, contain at that moment.

• Each number of new honeydrops is the ones digit of the surrounding total.

• The two top cells indicated by arrows are the beginning and end of the path and should each receive 2 drops, with the central 12 included in their totals.

No need to spoilerize a text solution. Site implementation makes that unduly onerous.

Also no need to be daunted by apparent complexity. The path is quite constrained topologically and luck alone probably gives a 1/10 chance of ending correctly.

A little well-directed reckoning can help approach this in a way that eases recovery from inevitable addition mistakes. (Yes, this claim follows much misdirected reckoning and many mistakes.)

The following possible sequence of seven initial steps demonstrates how the path works.

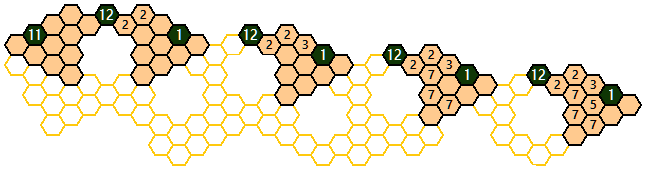
The first two steps here drip 2 drops each because the only adjacent honey on the first step is 12 (last digit = 2) and on the second step is simply 2. The seventh step drips 5 drops as the total of its surrounding cells is 1+3+7+7+7 = 25 (last digit = 5).

This is meant to be convenient on paper or in a text editor.   Here is a template for <pre>...</pre>:

                             ___     ___     ___
                         ___/   \___/ 12\___/   \___
                     ___/   \___/   \___/   \___/   \___
                 ___/ 11\___/   \___/   \___/   \___/ 1 \___
             ___/   \___/   \___/           \___/   \___/   \___
            /   \___/   \___/   \           /   \___/   \___/   \
            \___/   \___/   \___/           \___/   \___/   \___/
         ___/   \___/   \___/   \___     ___/   \___/   \___/   \___
        / 10\___/           \___/   \___/   \___/           \___/ 2 \
        \___/   \           /   \___/   \___/   \           /   \___/
     ___/   \___/           \___/   \___/   \___/           \___/   \___
    /   \___/   \___     ___/   \___/   \___/   \___     ___/   \___/   \
    \___/   \___/   \___/   \___/           \___/   \___/   \___/   \___/
    /   \___/   \___/   \___/                   \___/   \___/   \___/   \
    \___/           \___/   \                   /   \___/           \___/
    / 9 \           /   \___/                   \___/   \           / 3 \
    \___/           \___/   \                   /   \___/           \___/
    /   \___     ___/   \___/                   \___/   \___     ___/   \
    \___/   \___/   \___/   \___             ___/   \___/   \___/   \___/
    /   \___/   \___/   \___/   \___     ___/   \___/   \___/   \___/   \
    \___/   \___/           \___/   \___/   \___/           \___/   \___/
        \___/   \           /   \___/   \___/   \           /   \___/
        / 8 \___/           \___/   \___/   \___/           \___/ 4 \
        \___/   \___     ___/   \___/   \___/   \___     ___/   \___/
            \___/   \___/   \___/           \___/   \___/   \___/
            /   \___/   \___/   \           /   \___/   \___/   \
            \___/   \___/   \___/           \___/   \___/   \___/
                \___/ 7 \___/   \___     ___/   \___/ 5 \___/
                    \___/   \___/   \___/   \___/   \___/
                        \___/   \___/ 6 \___/   \___/
                            \___/   \___/   \___/

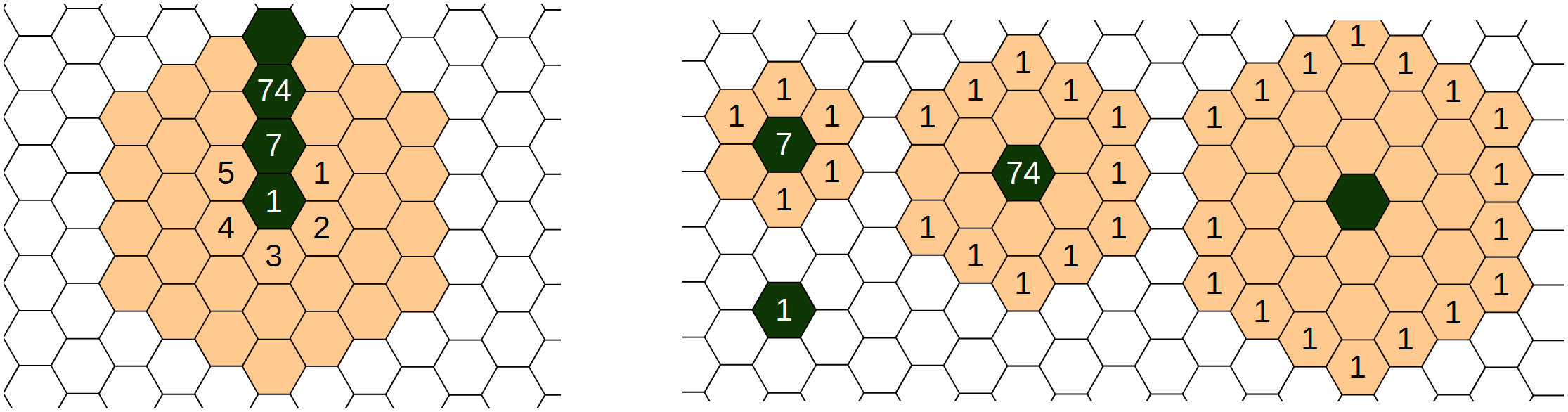
And here is how the seven-step example could begin to resemble a maze:

                             ___     ___     ___
                         ___/   \___/ 12\___/ 2 \___
                     ___/   \___/   \___/ 2  ___  3 \___
                 ___/ 11\___/   \___/   \___/ 7  ___/ 1 \___
                /   \___/   \___/           \   / 5 \___/   \
                \___/   \___/   \           / 7 \       \___/
                    \___/   \___/           \___  7 \___/
                        \___/   \           /   \___/
                            \___/           \___/

This puzzle forthrightly, though incompletely, imitates Two honeycomb hints by Yuriy S.

Answer 1

Okay, so there are probably other paths, but the one I found ends with a honeycomb that looks like this (I deleted some lines so you can see the path taken, like in the maze example given above):

                             ___     ___     ___
                         ___/ 1 \___/ 12\___/ 2 \___
                     ___/ 2  ___  2 \___  2  ___  3 \___
                 ___/ 11\___  9 \___/   \___/ 1 \   / 1 \___
             ___/ 8 \___/ 2  ___/           \     4 \___/ 5 \___
            / 9 \     7 \___  7 \           / 5 \___/ 4  ___  5 \
            \     8 \___  7  ___/           \___  9  ___/ 4  ___/
         ___/ 9 \___/   \___/ 0 \___     ___/ 6 \___/   \___  1 \___
        / 10\   /           \     0 \___/ 9     /           \   / 2 \
        \___/ 9 \           / 0 \   / 0  ___/ 2 \           / 3 \___/
     ___/ 3  ___/           \   / 0  ___/ 1  ___/           \___  5 \___
    / 1  ___/ 6 \___     ___/ 0 \___/   \___  1 \___     ___/ 6 \___  5 \
    \   / 6  ___  6 \___/ 0  ___/           \___  1 \___/ 6  ___  8 \   /
    / 5  ___/   \___  6 \   /                   \   / 6  ___/   \___  8 \
    \___/           \   / 0 \                   / 5 \   /           \___/
    / 9 \           / 6 \   /                   \   / 6 \           / 3 \
    \___/           \   / 0 \                   / 3 \   /           \___/
    / 0 \___     ___/ 6 \   /                   \   / 6 \___     ___/ 9 \
    \     2 \___/ 6  ___/ 0 \___             ___/ 1 \___  6 \___/ 6     /
    / 1 \___  6  ___/   \___  0 \___     ___/ 5  ___/   \___  6  ___/ 5 \
    \___  1 \___/           \___  0 \___/ 1  ___/           \___/ 1  ___/
        \___  3 \           / 8 \___  8 \___  4 \           / 1  ___/
        / 8 \   /           \     8  ___/ 7  ___/           \   / 4 \
        \___/ 5 \___     ___/ 6 \___/   \___  7 \___     ___/ 5 \___/
            \___  1 \___/ 2 \   /           \___  6 \___/ 0 \   /
            / 6  ___/ 9     / 6 \           / 1  ___/ 5     / 5 \
            \___  6  ___/ 0 \   /           \   / 0  ___/ 0  ___/
                \___/ 7 \   / 6 \___     ___/ 1 \   / 5 \___/
                    \___/ 1 \___  2 \___/ 1  ___/ 5 \___/
                        \___  8  ___/ 6 \___  5  ___/
                            \___/   \___/   \___/

As to how I got to this answer, I first set about finding a valid path that visited every cell. The first path I found ended with 4, so then I just needed to tweak the end of the path a little bit to get it to work.

As to how I found a path in the first place

There are a few cells in the clock which are only connected to two other cells
What this means is that cell must be traversed in a fixed order (well, two orders, either forwards or backwards). This happens in 8 places, marked with an X.

                             ___     ___     ___
                         ___/   \___/ 12\___/   \___
                     ___/   \___/   \___/   \___/   \___
                 ___/ 11\___/   \___/   \___/   \___/ 1 \___
             ___/   \___/   \___/           \___/   \___/   \___
            /   \___/   \___/   \           /   \___/   \___/   \
            \___/   \___/   \___/           \___/   \___/   \___/
         ___/   \___/   \___/   \___     ___/   \___/   \___/   \___
        / 10\___/           \___/   \___/   \___/           \___/ 2 \
        \___/   \           /   \___/   \___/   \           /   \___/
     ___/   \___/           \___/   \___/   \___/           \___/   \___
    /   \___/   \___     ___/   \___/   \___/   \___     ___/   \___/   \
    \   /   \___  X \___/   \___/           \___/   \___/ X  ___/   \   /
    / X  ___/   \___    \___/                   \___/    ___/   \___  X \
    \___/           \___/   \                   /   \___/           \___/
    / 9 \           /   \___/                   \___/   \           / 3 \
    \___/           \___/   \                   /   \___/           \___/
    / X \___     ___/   \___/                   \___/   \___     ___/ X \
    \       \___/ X  ___/   \___             ___/   \___  X \___/       /
    /   \___/    ___/   \___/   \___     ___/   \___/   \___    \___/   \
    \___/   \___/           \___/   \___/   \___/           \___/   \___/
        \___/   \           /   \___/   \___/   \           /   \___/
        / 8 \___/           \___/   \___/   \___/           \___/ 4 \
        \___/   \___     ___/   \___/   \___/   \___     ___/   \___/
            \___/   \___/   \___/           \___/   \___/   \___/
            /   \___/   \___/   \           /   \___/   \___/   \
            \___/   \___/   \___/           \___/   \___/   \___/
                \___/ 7 \___/   \___     ___/   \___/ 5 \___/
                    \___/   \___/   \___/   \___/   \___/
                        \___/   \___/ 6 \___/   \___/
                            \___/   \___/   \___/

Then:

From there, I came up with these partial paths, let's use lowercase letters to show the steps from a to s:

                             ___     ___     ___
                         ___/   \___/ 12\___/   \___
                     ___/   \___/   \___/   \___/   \___
                 ___/ 11\___/   \___/   \___/   \___/ 1 \___
             ___/   \___/   \___/           \___/   \___/   \___
            /   \___/   \___/   \           /   \___/   \___/   \
            \___/   \___/   \___/           \___/   \___/   \___/
         ___/ s \___/   \___/   \___     ___/   \___/   \___/ a \___
        / 10\   /           \___/   \___/   \___/           \   / 2 \
        \___/ r \           /   \___/   \___/   \           / b \___/
     ___/ q  ___/           \___/   \___/   \___/           \___  c \___
    / p  ___/ m \___     ___/   \___/   \___/   \___     ___/ g \___  d \
    \   / n  ___  l \___/   \___/           \___/   \___/ h  ___  f \   /
    / o  ___/   \___  k \___/                   \___/ i  ___/   \___  e \
    \___/           \   /   \                   /   \   /           \___/
    / 9 \           / j \___/                   \___/ j \           / 3 \
    \___/           \   /   \                   /   \   /           \___/
    / e \___     ___/ i \___/                   \___/ k \___     ___/ o \
    \     f \___/ h  ___/   \___             ___/   \___  l \___/ n     /
    / d \___  g  ___/   \___/   \___     ___/   \___/   \___  m  ___/ p \
    \___  c \___/           \___/   \___/   \___/           \___/ q  ___/
        \___  b \           /   \___/   \___/   \           / r  ___/
        / 8 \   /           \___/   \___/   \___/           \   / 4 \
        \___/ a \___     ___/   \___/   \___/   \___     ___/ s \___/
            \___/   \___/   \___/           \___/   \___/   \___/
            /   \___/   \___/   \           /   \___/   \___/   \
            \___/   \___/   \___/           \___/   \___/   \___/
                \___/ 7 \___/   \___     ___/   \___/ 5 \___/
                    \___/   \___/   \___/   \___/   \___/
                        \___/   \___/ 6 \___/   \___/
                            \___/   \___/   \___/

Then I started thinking about how to fill the middle circle of the clock.

The only openings into the center are now at 1, 5, 7, and 11.
So I decided the best route would be one that filled in the area around 1 o'clock, did the partial loopy path from 2 to 4 o'clock,
and then, starting near 5 o'clock, circle around the inner loop, exiting at 7 o'clock.
Then you do the partial path from 8 to 10, fill in the area around 11, and then you're done.

As to how I picked specific steps in that more general path, I honestly just picked whichever direction came first if you were thinking clockwise whenever there was a choice to make. As I said, this originally led me to a path ending with the number 4, not 2, but fixing it was fairly trivial, I just changed some choices I made filling in the area around 11 o'clock.

Answer 2

Wrap-up: the making of Honeydripping around the clock

This is not a solution to the puzzle but provides notes from its poser. This type of answer has been approved by the community.

Caution: This post contains information about the solution.

---

This is the third experiment with self-defining hexagonal-tiling mazes inspired by Two honeycomb hints and, especially, Two honeycomb hints, part two by Yuriy S.

    

The first experiment was a simple compact maze with unwieldy sums: H-one-one-oneycomb. Next came a maze with almost-trivial sums and additional guiding features: How to fill a honeymoon.

                    

So it was time to explore structures whose traversals could feel more like journeying than like space-filling. The conceptual point of departure was to simply have a hexagon made of hexagons made of hexagons, which led to a variety of possible forms.

The purest version of the hexagonal concept, marked 12 here, turned out to be too repetitive to be interesting. Version 7 felt like a discovery more than a construction, with it’s beautifully paradoxical harmonization of 6-fold and 7-fold symmetries, as it naturally includes many different local configurations. Version 10 wound up with rounded corners, to avoid unnecessarily many small-scale decisions, and that suggested the final clock format.

Would have been descriptively clean to begin the maze at 1 o’clock and end at 12, but that allowed two very different overall routes, one that was an all-too-regular zig-zag and another much more interesting route that included a sneaky counterclockwise inner loop along with some zig-zags. Both routes had the lucky feature of forcing the hours to be visited in order. Hah, beginning at 12 would allow only the more interesting route.

Now to check if this is plain too easy to solve — or, more likely, too tedious, especially as arithmetic mistakes could require redoing a lot of work. A few sloppy failed test solutions were enough to realize that it wasn't too easy and that, surprise, most arithmetic mistakes had only local effects. Some analysis revealed three particularly forgiving lucky features, labeled A, B and C.

A demonstrates that 2, 3 and 4 o'clock add a combined 0 to the running total. B demonstrates that any sum (or mistake) arriving at 2 or 8 o'clock will be multiplied by 5, which turns all even sums$\scriptsize\raise.2ex/$mistakes into 0 and prepares odd sums$\scriptsize\raise.2ex/$mistakes to become 0 at their inevitable next doubling. C demonstrates that the clock’s inner loop has no net effect on the peripheral loop at 9 o'clock—whew!

More fun than anticipated to analyze and test solve, for hours and hours, this was ready to be posed.