H-one-one-oneycomb

Question

       

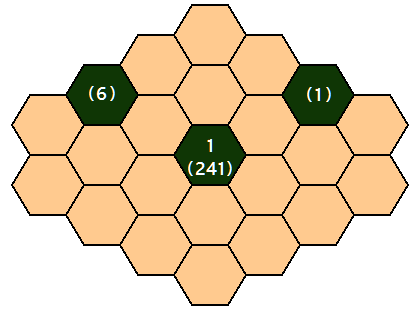
What path could a honeybee follow to fill all cells with honey, beginning and ending at the center and visiting every cell exactly once?

• At first the only honey is 1 drop in the center cell.

• Each step consists of moving to an adjacent cell and filling it with as many honeydrops as its 6 neighbors, combined, contain at that moment.

• The cell marked (1) is empty at first but should receive 1 honeydrop when it is reached. (Thus, the previous cell in the path would have 1 drop and no other adjacent cells would have been visited yet.)

• The cell marked (6) should receive 6 drops when the path gets there.

• The path ends back at the center cell, whose 6 adjacent cells have a total of 241 drops by then.

No need to spoilerize a text solution. Site implementation makes that unduly onerous.

The following sequence of six steps demonstrates how the rightmost cells might be filled first.

Unknown at an hour after post time: Is there a path that ends with a smaller number than 241?
What is the smallest possible ending number without the constraint of (6)? Already ffao and Jonathan Allan have found paths under 140.

This puzzle forthrightly, though incompletely, imitates Two honeycomb hints by Yuriy S.

This is meant to be convenient on paper and in a text editor.   Here is a template for <pre>...</pre>:

                 ___                                         ___
             ___/   \___                                 ___/ ? \___
         ___/   \___/   \___                         ___/ ? \___/ ? \___
     ___/(6)\___/   \___/(1)\___                 ___/ 6 \___/ ? \___/ 1 \___
    /   \___/   \___/   \___/   \               / ? \___/ ? \___/ ? \___/ ? \
    \___/   \___/ 1 \___/   \___/     --->      \___/ ? \___/241\___/ ? \___/
    /   \___/   \___/   \___/   \               / ? \___/ ? \___/ ? \___/ ? \
    \___/   \___/   \___/   \___/               \___/ ? \___/ ? \___/ ? \___/
        \___/   \___/   \___/                       \___/ ? \___/ ? \___/
            \___/   \___/                               \___/ ? \___/
                \___/                                       \___/

And this is how the six-step example could begin to resemble a maze:

                 ___
             ___/   \___
         ___/   \___/   \___
     ___/   \___/   \___/(1)\___
    /   \___/   \___/ 1  ___  1 \
    \___/   \___/ 1  ___/ 4 \   /
    /   \___/   \___/   \     1 \
    \___/   \___/   \___/ 5 \___/
        \___/   \___/   \___/
            \___/   \___/
                \___/

Answer 1

Here is a way to 241:

                 ___
             ___/ c \___
         ___/ b \___/ d \___
     ___/ p \___/ v \___/ e \___
    / o \___/ a \___/ u \___/ g \
    \___/ q \___/ w \___/ f \___/
    / n \___/ r \___/ t \___/ h \
    \___/ m \___/ s \___/ i \___/
        \___/ l \___/ j \___/
            \___/ k \___/
                \___/

                 ___
             ___/ 1 \___
         ___/ 1 \___/ 1 \___
     ___/ 6 \___/61 \___/ 1 \___
    / 4 \___/ 1 \___/56 \___/ 2 \
    \___/19 \___/241\___/ 1 \___/
    / 4 \___/29 \___/52 \___/ 3 \
    \___/ 4 \___/42 \___/ 4 \___/
        \___/ 4 \___/ 4 \___/
            \___/ 4 \___/
                \___/

And here is the smallest I can make:

136

                 ___
             ___/ c \___
         ___/ b \___/ d \___
     ___/ o \___/ v \___/ e \___
    / n \___/ a \___/ u \___/ f \
    \___/ p \___/ w \___/ t \___/
    / m \___/ q \___/ s \___/ g \
    \___/ l \___/ r \___/ h \___/
        \___/ k \___/ i \___/
            \___/ j \___/
                \___/

                 ___
             ___/ 1 \___
         ___/ 1 \___/ 1 \___
     ___/ 3 \___/48 \___/ 1 \___
    / 1 \___/ 1 \___/43 \___/ 1 \
    \___/ 7 \___/136\___/22 \___/
    / 1 \___/11 \___/18 \___/ 1 \
    \___/ 1 \___/15 \___/ 1 \___/
        \___/ 1 \___/ 1 \___/
            \___/ 1 \___/
                \___/