How to fill a honeymoon

Question

       

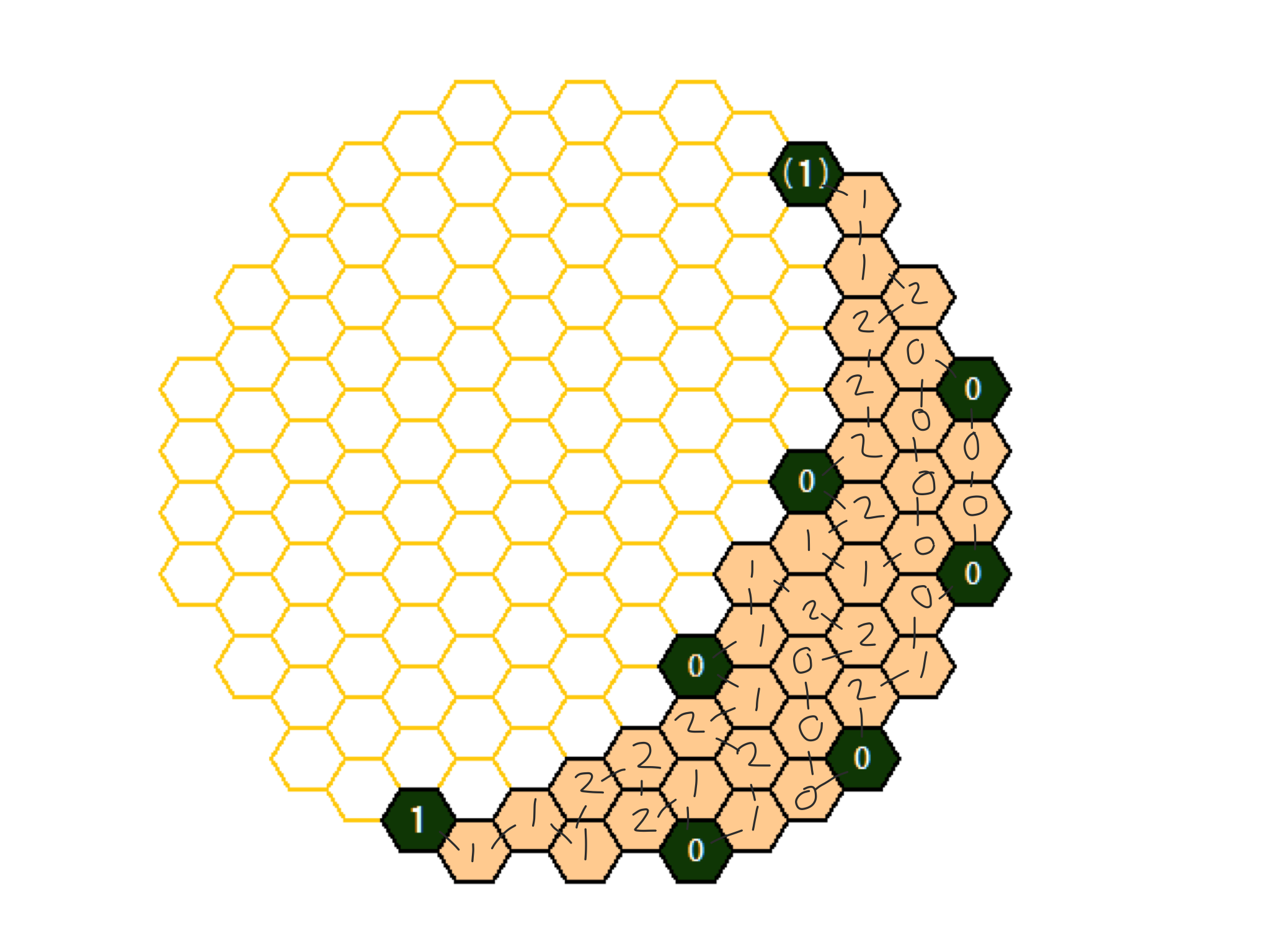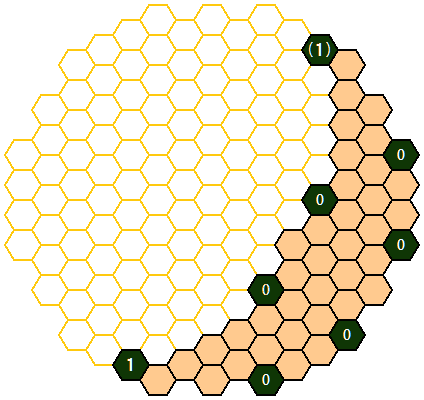
How can a honeybee visit all cells exactly once in this crescent shaped honeycomb, beginning at the bottom tip and ending at the top?

• The starting cell, at lower left, has 1 drop of honey. All other cells begin empty.

• Each step consists of moving to an adjacent cell and filling it with 0, 1 or 2 honeydrops, based on how many total drops its (1 to 6) neighboring cells, combined, contain at that moment.

• The number of new honeydrops is the remainder of the surrounding total when divided by 3.

$$\small \begin{matrix} \textsf{Total adjacent drops} ~&~ 0,3,6,9,12 ~&~ 1,4,7,10 ~&~ 2,5,8,11 \\ \textsf{Number of new drops} ~&~ 0 ~&~ 1 ~&~ 2 \\ \end{matrix}$$

• The top cell, marked (1), is empty at first but should receive 1 honeydrop when it is reached.

• The six cells with 0 should receive 0 drops when they are reached. (The sums of their neighbors’ drops should be multiples of 3 at those moments.) Other cells may also receive 0 drops.

No need to spoilerize a text solution. Site implementation makes that unduly onerous.

The following sequence of eight steps demonstrates how the bottommost 0 cell might be reached.

 

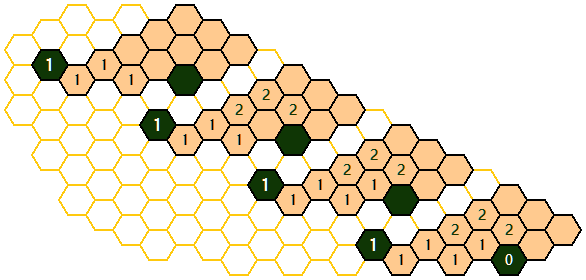
In this example, division by 3 comes into play when the last cell to receive 1 drop, on the seventh step, has a total of 1+2+2+2 = 7 drops in its adjacent cells, giving a remainder of 1 when divided by 3. The eight step correctly reaches the 0 cell as 1+2 = 3, which leaves 0 remainder when divided by 3.

This puzzle forthrightly, though incompletely, imitates Two honeycomb hints by Yuriy S.

This is meant to be convenient on paper and in a text editor.   Here is a template for <pre>...</pre>:

                                                         ___
                                                        /(1)\___
                                                        \___/   \
                                                            \___/
                                                            /   \___
                                                            \___/   \
                                                            /   \___/
                                                            \___/   \___
                                                            /   \___/ 0 \
                                                            \___/   \___/
                                                         ___/   \___/   \
                                                        / 0 \___/   \___/
                                                        \___/   \___/   \
                                                     ___/   \___/   \___/
                                                    /   \___/   \___/ 0 \
                                                    \___/   \___/   \___/
                                                 ___/   \___/   \___/
                                                / 0 \___/   \___/   \
                                                \___/   \___/   \___/
                                             ___/   \___/   \___/
                                         ___/   \___/   \___/ 0 \
                             ___     ___/   \___/   \___/   \___/
                            / 1 \___/   \___/   \___/   \___/
                            \___/   \___/   \___/ 0 \___/
                                \___/   \___/   \___/

And this is how the eight-step example could begin to resemble a maze:

                                                \___/
                                             ___/   \___/
                                         ___/ 2 \___/   \___/
                             ___     ___/ 2  ___  2 \___/   \_
                            / 1 \___/ 1 \   / 1  ___/   \___/
                            \___  1  ___  1 \___  0 \___/
                                \___/   \___/   \___/

Answer 1

I noticed that things got a lot easier the more zeroes you are able to stick in there, and with that in mind, here's my solution:

Sorry it's not in text form, but I solved it on a tablet without a nice text editor. Lines between cells indicate where the bee has to travel.

If you'd like, I can update with a text version when I get to a computer, but I hope this is sufficiently readable on its own.

Answer 2

Working out the upper end in reverse was very helpful for finding a solution, before I'd done that, I had tried about half a dozen things, after solving all the forced moves possible there, my second try made it work.

                                                        /(1)\___
                                                        \_44/ 1 \
                                                            \_43/
                                                            / 1 \___
                                                            \_42/ 0 \
                                                            / 1 \_41/
                                                            \_40/ 2 \___
                                                            / 2 \_39/ 0 \
                                                            \_38/ 0 \_36/
                                                         ___/ 2 \_37/ 0 \
                                                        / 0 \_29/ 1 \_35/
                                                        \_28/ 2 \_30/ 2 \
                                                     ___/ 1 \_27/ 1 \_34/
                                                    / 2 \_26/ 1 \_31/ 0 \
                                                    \_19/ 1 \_25/ 2 \_33/
                                                 ___/ 2 \_20/ 0 \_32/
                                                / 0 \_18/ 2 \_24/ 0 \
                                                \_17/ 2 \_21/ 0 \_23/
                                             ___/ 1 \_15/ 1 \_22/
                                         ___/ 2 \_16/ 1 \_14/ 0 \
                             ___     ___/ 2 \_6_/ 2 \_11/ 0 \_13/
                            / 1 \___/ 1 \_5_/ 1 \_7_/ 2 \_12/
                            \_1_/ 1 \_3_/ 1 \_8_/ 0 \_10/
                                \_2_/   \_4_/   \_9_/