While playing chess Parcly and Tori Taxel, best friends and genies, got bored and transformed all the pieces into pawns to make pretty patterns. They found this 22-pawn arrangement where every 3×3 submatrix (not necessarily contiguous) has at least one pawn.
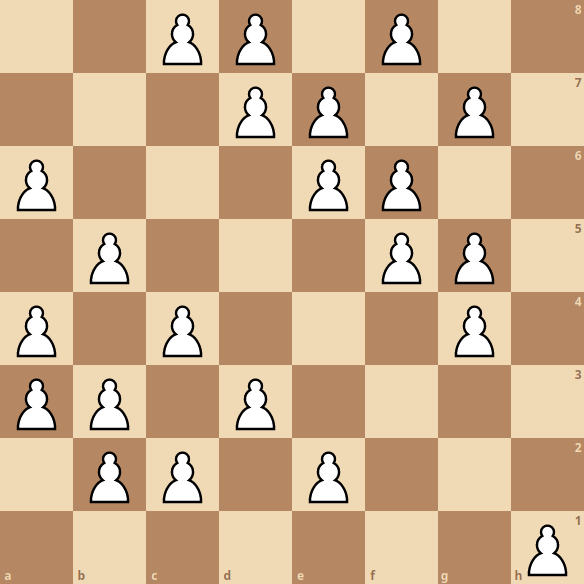
"But," Tori asked Parcly. "What if I remove one pawn?"
Is it possible to arrange 21 pawns so that every 3×3 submatrix is non-empty?
This puzzle was inspired by an MSE question.
