After Black Cavalry successfully captured White princesses,
White empire learns their Black opponent is hungry for more and wants White Twin Queens dead!
White will never let such a disaster happen and prepare their defense.
White castle is built by white's women and men's hands. A billion endeavor for a single white case. A billion ingot for a single black case. Most tremendous labor in White's empire history resulting in 80 more cases.

White's empire also made the greatest academic investment, to bear the smartest researchers in all fields making ALL white Pawns and both Queens more powerful.
Now, each white pawns can kill an opponent on both top diagonals, as they always did, plus on both down diagonals making them 4 cases to threat. That's not all. Each white pawn is now equipped with a poison blade which revenge him and poisons his murderer and making him impossible to perform any move or attack for the next turn.
Ladies Queens have a special tunnel within the castle making them return to their initial case instantly after killing a Black piece.

Now, I must warn you. As your black right hand, the following is top secret.
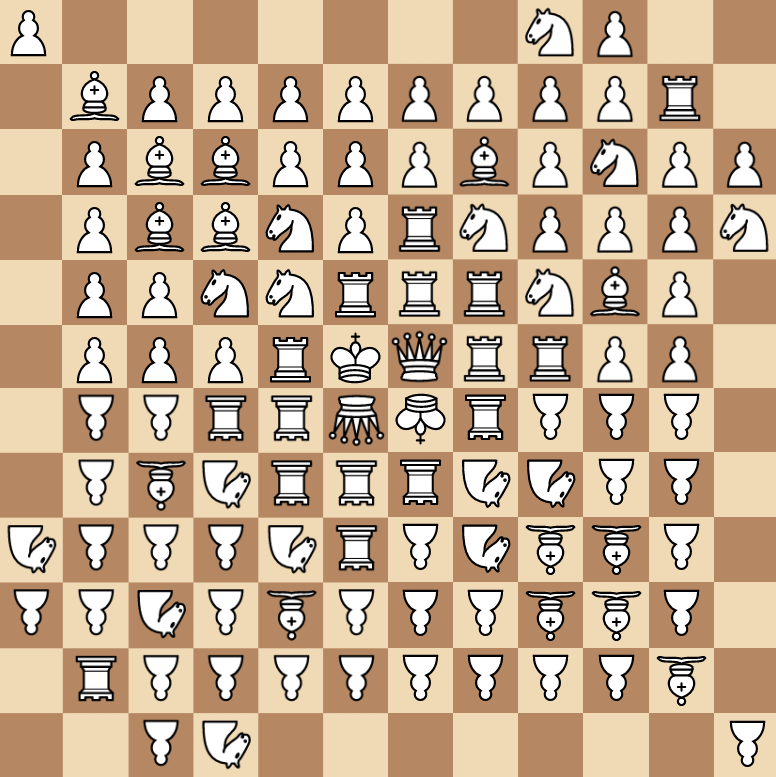
Our best spies got white's castle's exact map to prepare our battle.
How our army attacks and would win
Do not hesitate to look for the small example at the puzzle's end.
1. Battle's length
We will only have $20$ moves before White's empire decide to make their alive queens escape. If exceeding 20 moves, battle is lost.
2. Army's cost
On each move we can place black knights or black bishops. All other pieces, towers, pawns, etc. stay in our castle to avoid counter attack. A black attacking piece can be placed on the check board on a case if and only if it is empty. At move $i\in N=\{1,2,3,...,20\}$ placing one piece costs $i$ units of gold. Example : if we decide to place 2 knights and 3 bishops on step $1$, that costs $5$ gold. 3 knigths on step 3 costs $9$ gold.
3. Army's skills
For each $i\in N$, we have to decide how many knights and bishops we will send on the battle field. After placing them, each black piece either moves or attacks any piece we choose.
4. White defense's skills
Between each steps $i$, i.e. at most $19$ times, Whites defend according to the following rules:
- Black pieces closest to queens killed first
- Kill with white king first - note : killing a King isn't more important than killing any white piece except for twin queens, unlike classic chess
- Kill with white towers on second
- Kill with pawns on third
- Then kill with bishops
- After that, kill with cavalry
- Finally twin Queens attack if necessary, then return to their origin case.
5. Battle's victory
Battle is won when both white queens are killed.
Killing first queen at step $i\in N$ rewards $2000-100i$ gold. Killing last queen at step $i\in N$ rewards $10~000 - 100i$ gold.
My +1 to all battle's victories. Selected answer is battle victory with most gold won
Exemple of battle
Here is a small example on a $3\times 3$ check-board with only one queen so you can understand the rules' spirit.
There will only be one Queen for this small example and battle is won when this White queen dies. First step costs $2$ golds for Blacks. One bishop and one knight.
Black attacks
White defends according to white defense rules on paragraph 4. White defense's skills.
Step 2. costs 6 more golds for black empire. Black decides to move no piece.
White's defense
Step 3. Final one because costs 3 golds and Blacks win
On this example, Black win $2~000 - 30\times 3 - 11 = 1~889$ units of gold but you can easily see this is not an optimal solution!
Surprise on battle day
This section is made thanks to @Don Thousand's and AxiomaticSystem's correct answers. That's why I like Puzzling.SE, you can be so easily surprised by the efficiency of the community for any puzzle you'll create!
One hour before the battle day, black empire discovers every empty case on the battle field that was empty in the map we knew has a pawn in it, except all cases on the borders.
Moreover, White scientists equipped all units with anti cavalry armors making them attacking only starting from step 4.
Finally, Whites got tactical advantage by attacking after Blacks placing pieces, and after black attacks. Instead of only after black attacks in our plans...