6
$\begingroup$

Everyone knows that sinking feeling when you blunder your queen in one move, but it could be worse.

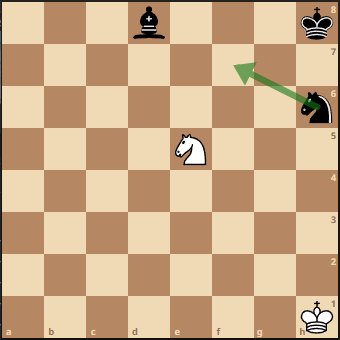
a primitive example

In the position above, Black plays Nf7. This blunders a knight in one move, as well as a bishop-king fork. In total, Black blundered 6 points of material in one move.

How much material can be blundered in this manner in one move?

Restrictions:

  1. The attacking side must capture on every turn.
  2. Every move must be forced (except the first blunder, of course).
  3. Your final score is (enemy material taken) - (your material loss).
Improve this question
$\endgroup$
8
  • $\begingroup$ Are you aware of an optimal solution to this? $\endgroup$
    – bobble
    Commented Apr 4, 2023 at 2:02
  • 1
    $\begingroup$ @bobble No, I'm not. Was that a requirement? I've seen a few puzzles of this nature before (ie. with strict restrictions but no known optimal answer). $\endgroup$
    – ApexPolenta
    Commented Apr 4, 2023 at 2:14
  • 6
    $\begingroup$ It's not strictly required, especially for [chess] since there's a chess-loving lobby hereabouts :) but if you knew one it would help. The idea is to prevent [open-ended] puzzles where the whole thing turns into an never-ending game of answers upon answers one-upping each other $\endgroup$
    – bobble
    Commented Apr 4, 2023 at 2:44
  • 5
    $\begingroup$ The biggest blunder — with the largest material loss — is, of course, to decline the Chess match in favour of Global Thermonuclear War… $\endgroup$
    – Chronocidal
    Commented Apr 4, 2023 at 21:01
  • 1
    $\begingroup$ Are you interested in legal positions only? Given the new, albeit wrong, answer uses an illegal position, you could in theory produce far bigger a score. $\endgroup$
    – Rewan Demontay
    Commented Apr 6, 2023 at 0:51

3 Answers 3

Reset to default
15
$\begingroup$

Score 156.

enter image description here

Thanks to @thisIs4d for helpful discussion.

Black could win by 1... Bg6-c2#

If instead they make the blunder 1... Ne3-g4 white can force the line

[Variant "From Position"] [FEN "Rrrqqqqq/PPPPPPP1/kq1qqqbb/7R/K7/4n3/8/8 b - - 1 1"]

1... Ng4 (1... Bc2#) 2. axb8=Q+ Qa7 3. Rxa7+ Kb6 4. bxc8=Q+ Kc6 5. cxd8=Q+ Qc7 6. Rxc7+ Kd6 7. dxe8=Q+ Qd7+ 8. Rxd7+ Ke6 9. exf8=Q+ Qe7 10. Rxe7+ Kf6 11. fxg8=Q+ Bf7 12. Rxf7+ Kg6 13. Qxg4+ Bg5 14. Rxg5+ Kh6 15. gxh8=Q#

Black's material at the start is 100, and is 0 at the end, netting us 100 points from that. White's material is worth 17 at the beginning, but 73 at the end; 17-73 = -56. By applying the same formula to both sides, you can take advantage of the scoring system's equation setup. Therefore, per restriction #3, our score is 100-(-56) = 156.

(click here to replay the moves on Chess Stack Exchange)

Improve this answer
$\endgroup$
12
  • $\begingroup$ I wonder why you didn't consider 8 queens instead in the first case above, wouldn't that increase the score to 136 (stalemate & checkmate can be avoided considering black king to blunder again)? Also, its good that both are possible situations in a chess game. $\endgroup$
    – thisIs4d
    Commented Apr 4, 2023 at 4:49
  • $\begingroup$ What's the move sequence in the "black could easily win with 1. ... Qh8xh7+" variation? Looks like the white king starts to run out of squares really fast. $\endgroup$
    – Bass
    Commented Apr 4, 2023 at 6:24
  • $\begingroup$ @thisIs4d I don't know how to do it while keeping black's moves forced. $\endgroup$
    – loopy walt
    Commented Apr 4, 2023 at 7:14
  • 1
    $\begingroup$ @loopywalt, can you consider following changes in the first solution above: 1. White queen on a8. 2. White rook on a6. 3. Black queen on a5. Rest is the same as shown above. Now if 1...Qa1, 2 Rxa1, you get your original solution with a +4 score, i.e. 123 $\endgroup$
    – thisIs4d
    Commented Apr 4, 2023 at 8:11
  • 1
    $\begingroup$ @thisIs4d I'm pretty sure that would make it an unreachable position because to get all the white pawns past all the black pawns 8 pieces must already have been captured and there are already 24 pieces on the board. $\endgroup$
    – loopy walt
    Commented Apr 4, 2023 at 10:45
1
$\begingroup$

For fun and an illegal position, using @loopy walt's matrix, here is a score of 206; (149)-(17-74).

enter image description here

FEN: Rqqqqqqq/PPPPPPP1/kq1qqqq1/7R/K2p2q1/4qp2/6r1/1q2b1Rq b - - 1 1

Black blunders 1... Rf2, followed by 2. axb8=Q+ Qa7 3. Rxa7+ Kb6 4. bxc8=Q+ Kc6 5. cxd8=Q+ Qc7 6. Rxc7+ Kd6 7. dxe8=Q+ Qd7+ 8. Rxd7+ Ke6 9. exf8=R+ Qe7 10. Rxe7+ Kf6 11. fxg8=Q+ Qf7 12. Rexf7+ Kg6 13. Qxg4+ Qg5 14. Rxg5+ Kh6 15. Rxh1+ Rh2 16. Rxh2+ Bh4 17. gxh8=Q+ Qh7 18. Rxh7#

Improve this answer
$\endgroup$
0
$\begingroup$

Score 177.

enter image description here

Notice Black only has three available moves. After the blunder Qbc2 and bxa8=Q Black is in zugzwang and must sacrifice each of the remaining Queens. Then after the Black King takes back the Bishop, White can easily clean up all of the Black Rooks with the newly promoted Queens.

[Variant "From Position"] [FEN "qbrbrbrb/bPbPbPbP/rbrbrbrb/brbrbrbr/Kbrbrbrb/brbrbrbk/Bb1bqbqb/bqbqbqbq b - - 0 1"]

1... Qbc2 2. bxa8=Q Qb1 3. Bxb1 Qc2 4. Bxc2 Qd1 5. Bxd1 Qe2 6. Bxe2 Qf1 7. Bxf1+ Qg2 8. Bxg2+ Kxg2 9. dxc8=Q Kh3 10. fxe8=Q Kg2 11. hxg8=Q Kf1 12. Qxg6 Kg2 13. Qxh5 Kh3 14. Qcxe6 Kg2 15. Qxf5 Kh3 16. Qhxg4+ Kg2 17. Qxc6 Kh1 18. Qxb5 Kg2 19. Qxe4 Kh1 20. Qxa6 Kg2 21. Qxc4 Kf1 22. Qgxd5 Ke2 23. Kxb3 Kf1 24. Qcxd3+ Kg2 25. Qexf3#

Captured material 148, own losses 3 from the Bishop and -32 from promotion. Score 148-(3-32) = 177.

Improve this answer
$\endgroup$
2
  • 2
    $\begingroup$ I'd say there is no blunder on Black's part because there is no way they can win, so there is nothing being lost here. $\endgroup$
    – Rewan Demontay
    Commented Apr 6, 2023 at 0:44
  • 4
    $\begingroup$ Nor are all of Black's moves forced, since the Black king has lots of freedom. $\endgroup$
    – Rewan Demontay
    Commented Apr 6, 2023 at 0:47

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.