16
$\begingroup$

This puzzle is part of the Puzzling Stack Exchange Advent Calendar 2024. The accepted answer to this question will be awarded a bounty worth 50 reputation.

< Previous Door Next Door >

This is a Between Thermometer Sudoku.

Normal sudoku rules apply.

For "Between Line" Sudokus:

Digits along a "between line" must be strictly between the digits on the circled ends of the line.

For Thermo Sudokus:

Digits increase as they get farther away from the bulb.

To avoid possible confusion, there is a thermometer in box 2 extending upwards between R3C5 and R2C5, while the "between line" extends from R4C5 to R1C5.

The puzzle is possible without guesswork.

If you would like to practice the variants individually before doing the puzzle:
Between Line Sudoku
Thermo Sudoku

Puzzle: (img. link) (Sudokupad link)

Puzzle

Improve this question
$\endgroup$
3
  • $\begingroup$ Is there a sudokupad version of this? $\endgroup$
    – Mikez
    Commented Dec 11 at 16:03
  • $\begingroup$ @Mikez I can create one quick if you want $\endgroup$
    – CrSb0001
    Commented Dec 11 at 16:05
  • $\begingroup$ @Mikez Added the link. $\endgroup$
    – CrSb0001
    Commented Dec 11 at 16:14

2 Answers 2

Reset to default
11
+50
$\begingroup$

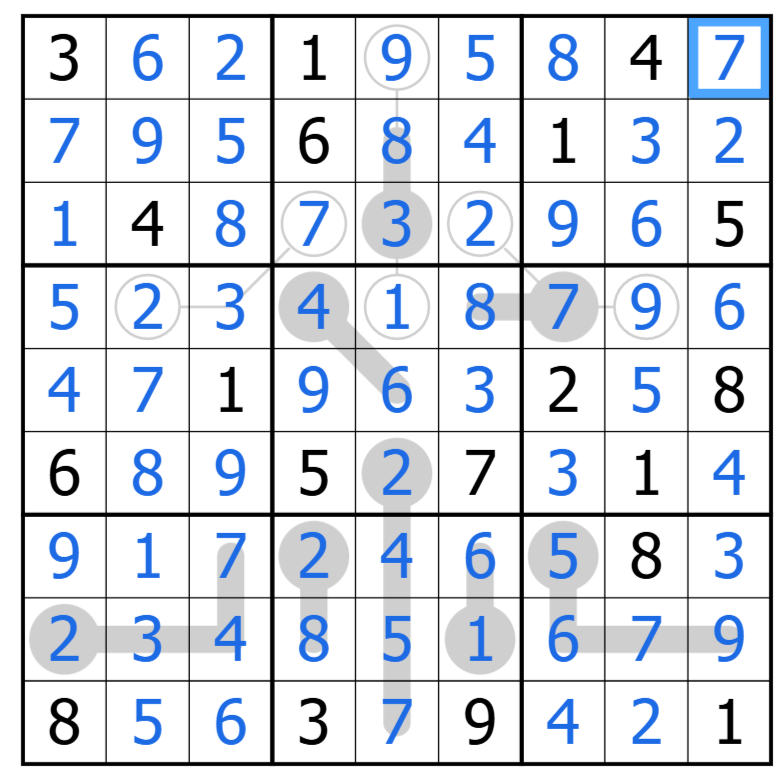
We can place the 1s using only basic sudoku and thermometer rules. If the 8 in box 8 is in r8c5 it would force r9c5 to be 9, so it must be in r8c4. Then we can fill 8 in r4c6 too: 1

In box 7 the 3 must be on the 2nd, 3rd or 4th steps of the thermometer, but it cannot go on the 4th or 3rd step because a 1 would then be forced below it. Thus it goes on the 2nd step (r8c2), and a 2 below it is forced as a result.

Now 9 is in r7c1 or r7c3, so box 9's 9 is on the thermometer on row 8. So it is in r8c9. 2

In box 8, 2 is constrained by the thermometer to lie in r7c4 or r7c6. This shadows into box 9 and fixes 2 in r9c8. We now mark some pencils on the vertical length-4 thermometer – it may only have numbers from 2 to 7 inclusive, and the rules exclude some candidates. 3

Suppose we put 4 in r2c5. Then we force 567 in r789c5, 7 in r3c4, 9 in r5c4 (it can't go on a thermometer bulb) and 2 or 3 in r3c5 and r6c5. This reserves 2 and 3 for those two cells, so 8 and 9 must occupy r15c5. But now r5c5 is unfillable. So r2c6 has 4. 4

4a

We now try 2 in r4c4. Then r4c2 may only be 5, 7 or 9; if r3c4 is 7 then r4c3 has to be 6 or 8 and will collide with a filled cell. Thus r3c4 must be 9. If now r4c2 is 7 it will force r4c3 to 8 and cause the aforementioned collision again. So r4c2 is 5, r4c3 7 and r5c8 7. But then 5 has no place to go in row 5. 5 So r6c5 is 2, and since putting 2 in r4c3 would force a tenth 1 in the grid, 2 is in r4c2. Since the endpoints of a between constraint cannot be the same, r7c4 is 2 too. 5a

It is now clear that 7 must be in the middle column of box 8, hence r9c5, which solves the middle column of boxes. 4 can then only be in row 6 of box 6; this shadows into box 4 and puts 4 in r5c1. Another shadowing deduction puts 4 in r6c9. 6

If 5 is put in r4c8 then r4c7 must be a 3, after which r4c3 cannot be legally filled. If 5 is in r4c7 or r6c7 there won't be a place for 5 in box 9, so r5c8 is 5. We fill in a few more cells. 7

Note that column 2 can only be completed in two ways now. If we try 8-5-9-6 we find we have no place to put 9 in row 2: 8 Thus it must be 6-9-8-5. We solve box 9 and a few more cells by sudoku and thermometer rules. 9

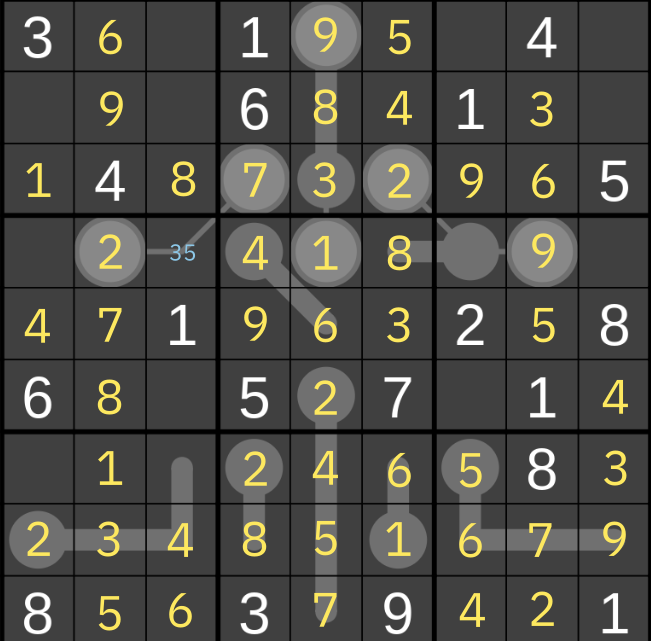
And before you know it the puzzle is solved. finish

Improve this answer
$\endgroup$
9
$\begingroup$

Pretty sure I solved it! Took me a bit, but I got there in the end!

Solved!

Improve this answer
$\endgroup$
2
  • 7
    $\begingroup$ Welcome to Puzzling. On this site, answers to [grid-deduction] questions are expected to explain at least a little of the solving process, for example pictures of intermediate steps, or description of a key deduction. This helps others learn how to solve puzzles and enjoy the solution. See answers to recent grid-deduction puzzles. Please edit your answer with an explanation if possible. $\endgroup$
    – bobble
    Commented Dec 11 at 22:06
  • 1
    $\begingroup$ +1 as you are a new user and it was pretty impressive that you were the first solver of the puzzle (it is correct by the way!) $\endgroup$
    – CrSb0001
    Commented 2 days ago

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.