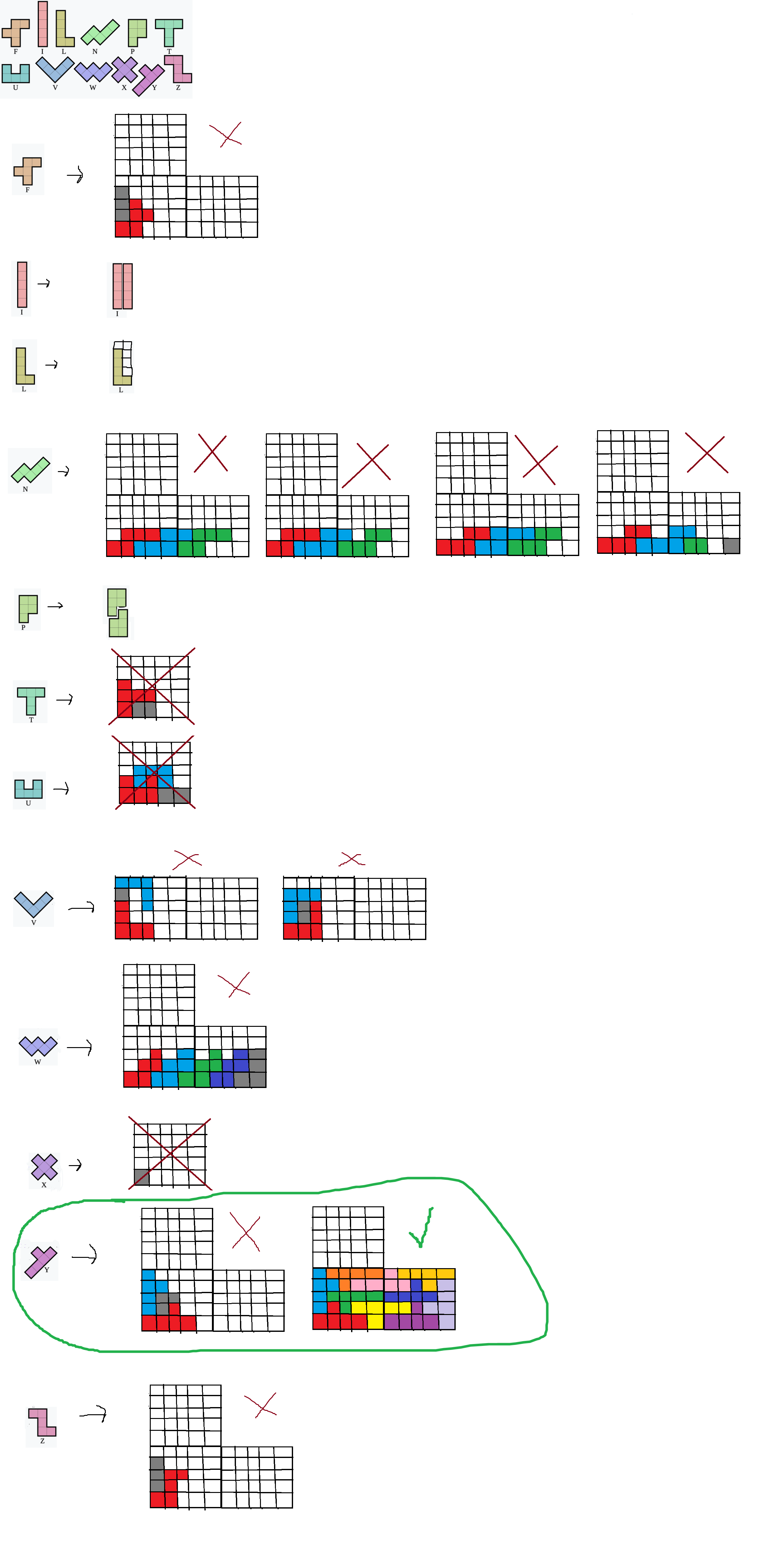
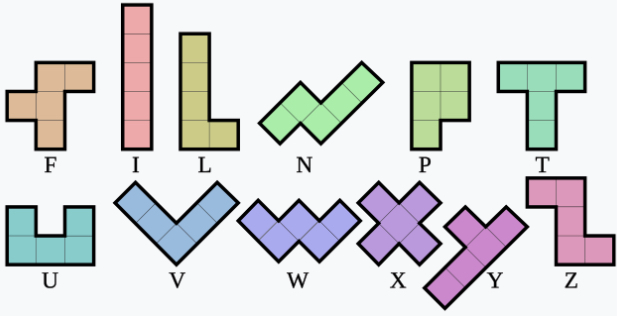5
$\begingroup$

Jules is playing with a pentomino set and suddenly shouts out in excitement. (A pentomino is any shape made from five identical squares by joining them along the full lengths of their edges.) “I have discovered a truly remarkable shape! Ten copies of the shape can be arranged (with some of them possibly flipped over) to form a rectangle, but with any fewer than ten copies, it’s impossible to make a rectangle.”

Which pentomino is Jules’ shape?

The 12 pentominoes are:

12 pentominoes

(image from Wikipedia)

Clarification: “To form a rectangle” means to tile it with no gaps, no overlaps and no part of any pentomino outside the rectangle. The pentominoes can be rotated and/or reflected.

Attribution: momath.org

Improve this question
$\endgroup$
1

1 Answer 1

Reset to default
7
$\begingroup$

Having $10$ pentominos means $50$ tiles. $50=5^2 \times 2$, so it can be $2 \times 25$ or $5 \times 10$. The ones that can form a rectangle of the height $2$ at all can form one without requiring a $2 \times 25$ one, so it's safe to assume it must be a $5 \times 10$ one.

Here's a picture (grey tiles are the "dead ends", the ones that can't be part of a pentomino):

enter image description here

Improve this answer
$\endgroup$
1
  • 2
    $\begingroup$ To display expressions like $2 \times 25$ you can use $2 \times 25$ $\endgroup$
    – Will.Octagon.Gibson
    Commented Sep 18 at 7:21

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.