4
$\begingroup$

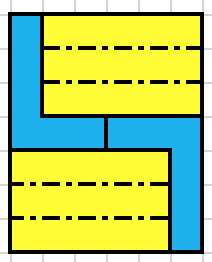
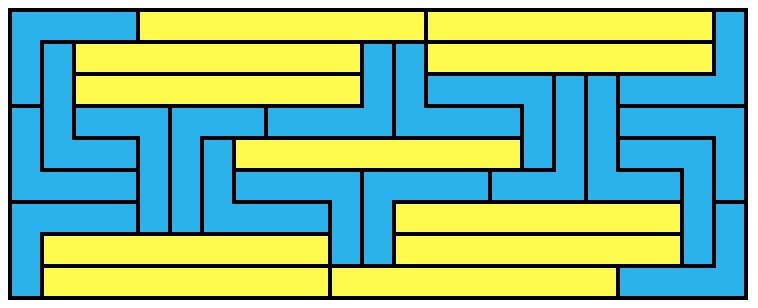
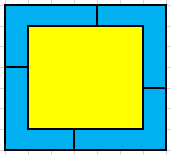
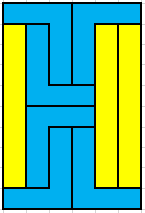
Inspired by Polyomino T hexomino and rectangle packing into rectangle

See also series Tiling rectangles with F pentomino plus rectangles and Tiling rectangles with Hexomino plus rectangle #1

Previous puzzle in this series: Tiling rectangles with Hexomino plus rectangle #2

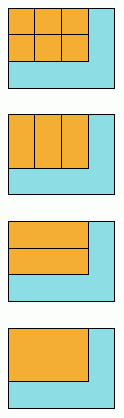
The goal is to tile rectangles as small as possible with the given hexomino, in this case number 3 of the 25 hexominoes which cannot tile a rectangle alone. We allow the addition of copies of a rectangle. For each rectangle $a\times b$, find the smallest area larger rectangle that copies of $a\times b$ plus at least one of the given hexomino will tile.

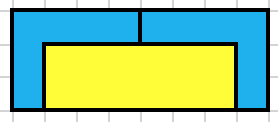
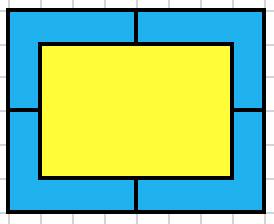
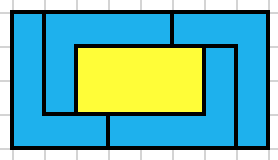
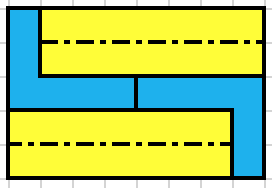
Example with the $1\times 1$, $1\times 2$, $1\times 3$ or $2\times 3$ you can tile a $3\times 4$ as follows:

1x1 1x2 1x3 2x3_3x4

Now we don't need to consider $1\times 1$, $1\times 2$, $1\times 3$ or $2\times 3$ further as we have found the smallest rectangle tilable with copies of the hexomino plus copies of those rectangles.

This is split into two sections: Those tilable by hand, and those probably requiring a computer. Feel free to solve any of them by hand, but please don't post computer-found tilings for those in the no-computer section.

No Computer section All of these should be tiled by hand only. This also means please don't look up answers on the web... This does not preclude you from for example using an image program to manipulate shapes on the screen, just from using a computer to search for or automate the arrangement.

  • Width 1: Lengths 1 to 3 (given), 4 to 8
  • Width 2: Lengths 2, 3 (given), 4 to 9
  • Width 3: Lengths 4 5 8
  • Width 4: Lengths 4 (difficult but worth it) 5 6
  • Width 5: Lengths 5 6

Computer section Master solvers may well solve these by hand.

  • Width 1: Lengths 9 to 12
  • Width 2: Lengths 10 12 14
  • Width 3: Length 7
  • Width 4: Length 7
Improve this question
$\endgroup$

2 Answers 2

Reset to default
4
$\begingroup$

Here are attempts for 2x2:

4x4=16
enter image description here

For 3x4:

4x6=24
enter image description here

This one for 1x4 is slightly smaller:

4x5=20
enter image description here

for 2x6 (and 1x6):

3x8=24
enter image description here

and for 4x6

6x8=48
enter image description here

and for 2x4

4x8=32
enter image description here

Here's one for 2x7 (which works for 1x7 as well):

5x8=40
enter image description here

and here is its 'inverse', for 3x5 and 1x5:

7x6=42
enter image description here

I found a computer-generated one in my archives, for 1x9:

9x23=207
enter image description here

Improve this answer
$\endgroup$
3
  • $\begingroup$ 2x2=4x4 is minimal, 3x4=4x6 is minimal, 1x4=4x6 is not, 1x6,2x6=3x8 both minimal, 4x6=6x8 is, 2x4=6x8 is not $\endgroup$
    – theonetruepath
    Commented Jun 14, 2018 at 5:46
  • $\begingroup$ Here are some updates. Solving these while driving to the office is hard ... $\endgroup$
    – Glorfindel
    Commented Jun 14, 2018 at 6:34
  • $\begingroup$ 1x4=4x5, 2x4=4x8 both minimal $\endgroup$
    – theonetruepath
    Commented Jun 14, 2018 at 6:56
2
$\begingroup$

Minimal 5x5:

7 x 7 = 49
enter image description here

Minimal 1x8:

6 x 10 = 60
enter image description here

Added attempt at 2x5:

8 x 7 = 56
enter image description here

Non-minimal 1x5 attempt:

7 x 7 = 49
enter image description here

Improve this answer
$\endgroup$
3
  • $\begingroup$ 5x5=7x7 is minimal, well spotted. 1x5 can be improved on. 1x8=6x10 also minimal. $\endgroup$
    – theonetruepath
    Commented Jun 15, 2018 at 0:46
  • $\begingroup$ @theonetruepath Thanks, I figured 1x5 wasn't minimal, but wasn't sure how to reduce it. Added a 2x5 attempt. $\endgroup$
    – hagfy
    Commented Jun 15, 2018 at 11:53
  • $\begingroup$ 2x5=7x8 is minimal yes $\endgroup$
    – theonetruepath
    Commented Jun 15, 2018 at 19:35

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.