12
$\begingroup$

Archived Source

Introducing Pentominoes!

It's the same concept as tetrominoes except they use 5 tiles instead of 4. Discounting rotations and reflections, there are 12 different free pentominoes. (If you include reflections, there are 18 but we're going to assume our pieces are all vampires and, thus, don't have reflections.) You can one of each pentomino to form a 6x10 rectangle without any overlap or gaps. You can also do this for 5x12, 4x15, and 3x30 rectangles. (Incidentally, 60 is an anti-prime (or highly composite) number and those are neat.)

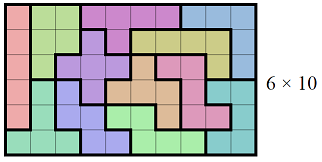
Here's an example of a $6\times10$ pentomino puzzle:

enter image description here

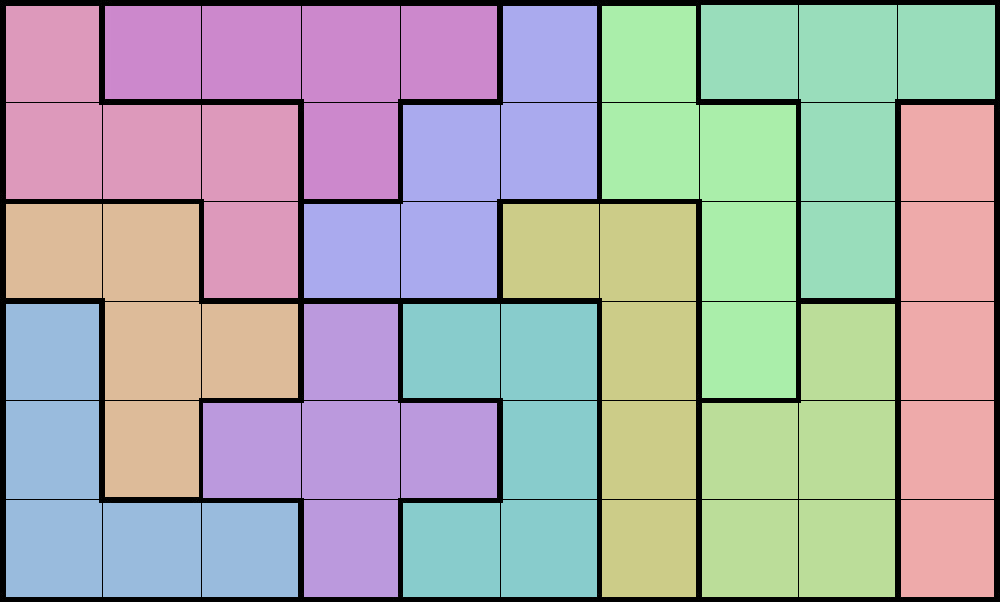
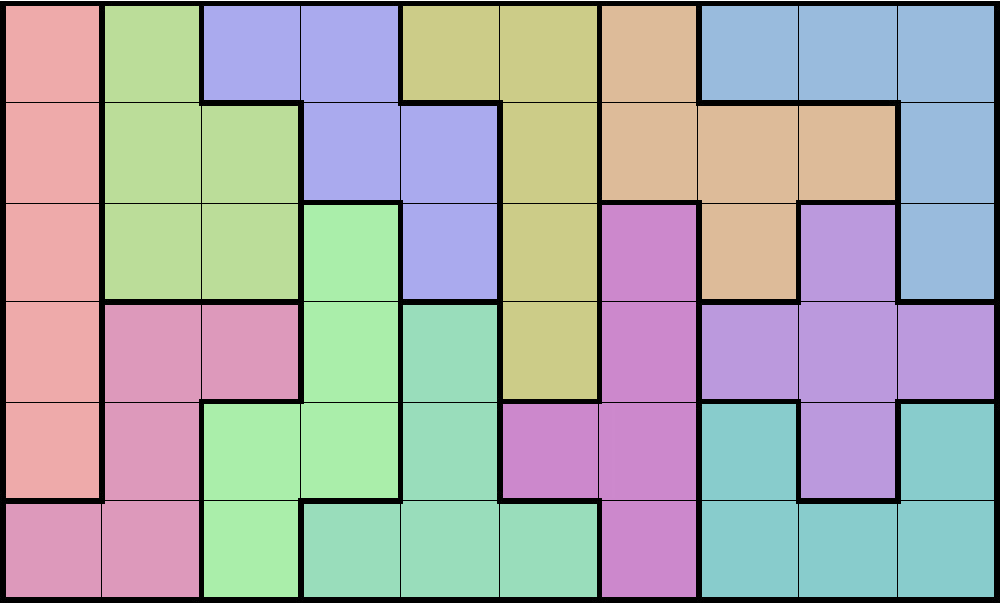
There are 2,339 unique solutions for the $6\times10$ puzzle. Here's the tricky part, though:

Can you arrange each of the 12 free pentominoes in a $6\times10$ rectangle such that each piece touches the edge of the rectangle?

You can find the answer online if you search a bit and nobody here will be able to tell. You will know, though, and the remorse shall haunt you the rest of your days.

Update: One solution has been found. There is exactly one more possible configuration that meets the requirement.

Improve this question
$\endgroup$

1 Answer 1

Reset to default
15
$\begingroup$

I found these solutions while playing around on https://www.scholastic.com/blueballiett/games/pentominoes_game.htm.

First arrangement

Second arrangement

Improve this answer
$\endgroup$
3
  • 1
    $\begingroup$ That's one solution. There is exactly one more. I'll leave it open for a bit to see if someone finds it. $\endgroup$
    – Engineer Toast
    Commented Dec 23, 2016 at 2:07
  • 1
    $\begingroup$ There's another one? Cool!!!! Back to work... $\endgroup$
    – wildBillMunson
    Commented Dec 23, 2016 at 2:07
  • 1
    $\begingroup$ Found it! Thanks for the excellent puzzle!! :) $\endgroup$
    – wildBillMunson
    Commented Dec 23, 2016 at 3:24

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.