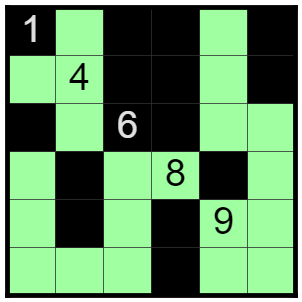
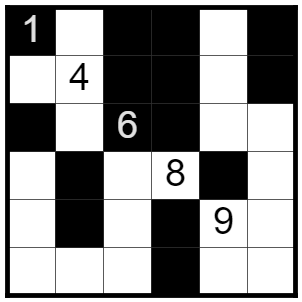
Here's the solution I found:
And here's how I found it:
The 1 must be shaded, as a single unshaded cell would be a rectangular region. We can also see that the 9 must be unshaded, as there is not enough room for a 1x9 on the board, and a 3x3 would run into the 8:
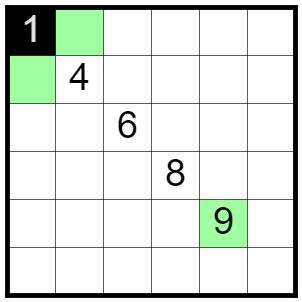
Now, it looks like the 4 can still be either shaded or unshaded, but at this point, if it is shaded, it can only be a 1x4. If that happens (picking one of two directions arbitrarily, since the puzzle is symmetric), we get this layout:

That single unshaded cell at the top must extend outward so as not to be a rectangular region, which immediately completes the 6. We can block it off with unshaded cells, and now we have the 8 as part of a 1x5 rectangle, and we can't extend that to a 1x8, so we've found a contradiction:
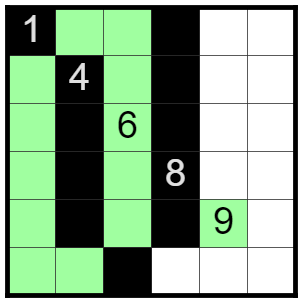
Therefore, the 4 must be unshaded. It has 3 cells as part of its region already, but even taking into account the fact that the grid is symmetrical, there are still two ways to complete the 4, either a T or a Z. Let's arbitrarily pick the Z and see what happens:
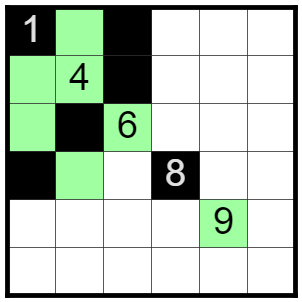
As you can see, the 6 now must be unshaded, and since both of the cells above it and to its left are shaded, it must extend to at least one of the cells that also borders the 8, meaning the 8 is shaded. If the 8 is shaded, it must be a 2x4, but it can't be a 2x4 without running into other shaded cells that would force it to be non-rectangular. Therefore, the 4 region must be a T:
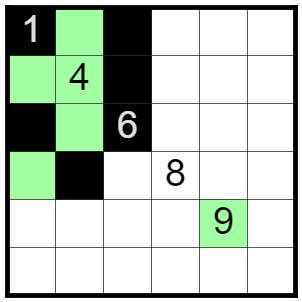
The 6 is now shaded, and it could be a 1x6 or a 3x2, but a 1x6 would force it into the other shaded square we just added below the 4 region, so it must be a 3x2. This means that the 8 is unshaded, and the two cells that would connect the 8 and the 9 must be shaded:
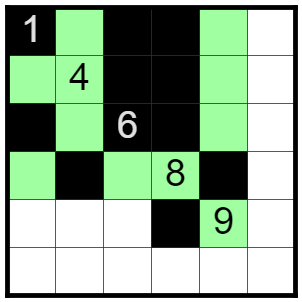
At this point, we need to take into account the extra prime-number-of-shaded-regions rule. We have 6 shaded regions so far, so we need at least 7, but we might be able to fit 11. Let's see if it's even theoretically possible. We need 17 cells to be unshaded between the 8 and 9 regions, but we have only 21 cells that can possibly be part of those regions, so we can only add 4 more regions, meaning we can't get to 11 regions. 7 is our only option. So we need to add 4 more shaded cells while only adding one new shaded region.
We also have to separate the 8 and 9 regions, so either the third, fourth, or fifth cell in the bottom row must be shaded. No matter which one we choose, however, we'll end up with more than 9 cells remaining in the 9 region, and the only way to extend an existing shaded region in this area would split the region in half. Therefore, it looks like our one additional shaded region must be in the 9 area, and we cannot create an extra one by shading the third or fifth cell. Thus, it must be the fourth, extending the existing shaded region by one:
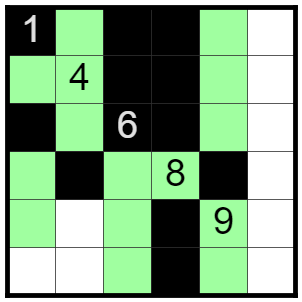
Now, we have 9 cells in the 8 region and 11 cells in the 9 region. The only cell we can shade in the 8 region is the one that's already adjacent to an existing shaded region, and the only two cells we can shade in the 9 region without splitting it are in the top right corner. And with that, the puzzle is complete: