For those who don't know how Hexologic works:
You can place one, two or three dots in each blank space and the rows that end in arrows must all add up to the number in the arrow.
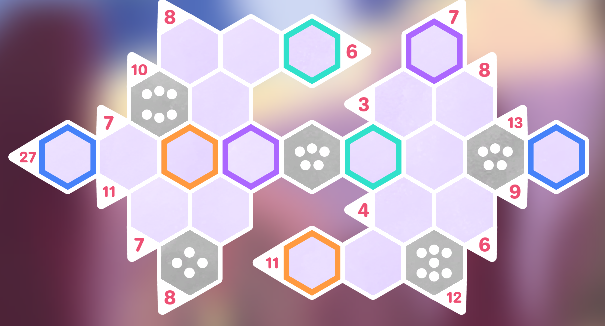
Here is Level 45 (easy mode) in Hexologic, which I have been trying to solve for about a week:
For those who don't know what those colored cells mean, if I click a cell that is colored cyan, then every other cell that is colored cyan is automatically filled in.
Also, about those greyed out cells with numbers already in them, that's already a part of the level
Now, the reason I hate this level so much is because there are so many ways that everything can be satisfied. On top of that, there's honestly so much going on that it just hurts to look at.
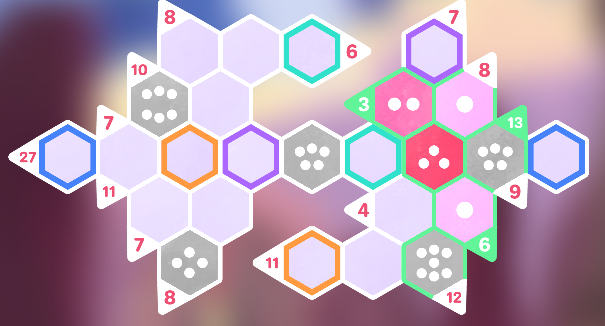
Is there anything that we can tell is correct right away? Yes, actually:
Here's a brief explanation:
- First of all, there is only one way to satisfy the 13.
- Then, we can notice that since we cannot satisfy the 3 if we put 3 dots in one of the cells, that means that there must be a 2 there, and obviously, we can satisfy the 13, 6, and 3 right away.
Then we can do this because there's only one way to satisfy the 4 and the 8:
And then we can satisfy quite a bit more automatically:
Now this is where I am currently stuck because I don't know what to do from here. This is mostly because now this obviously leads to a bunch of Diophantine equations that I am stuck trying to solve so I can deduce the solution logically. (although I hopefully don't have to do so) So my question is: