This puzzle was inspired by this one
Who will be the first to solve this tricky math crossword puzzle completely?
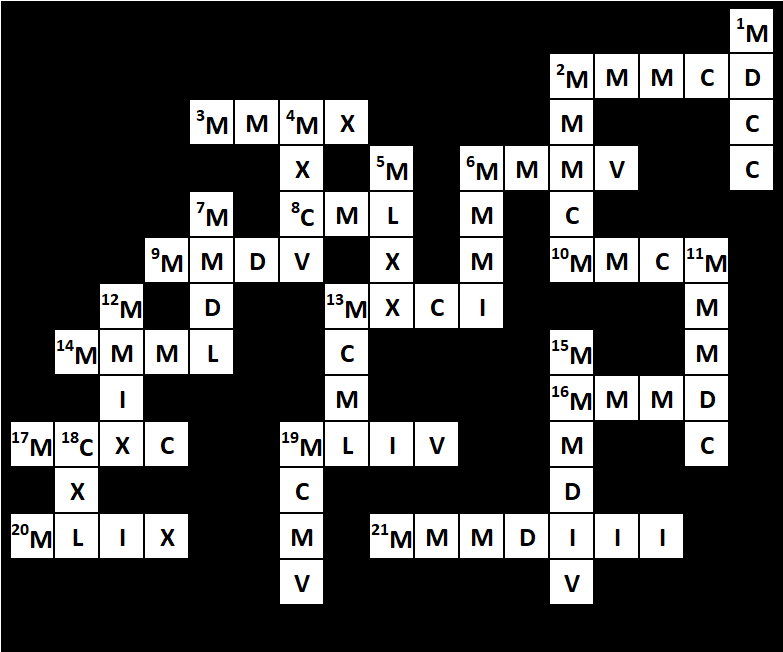
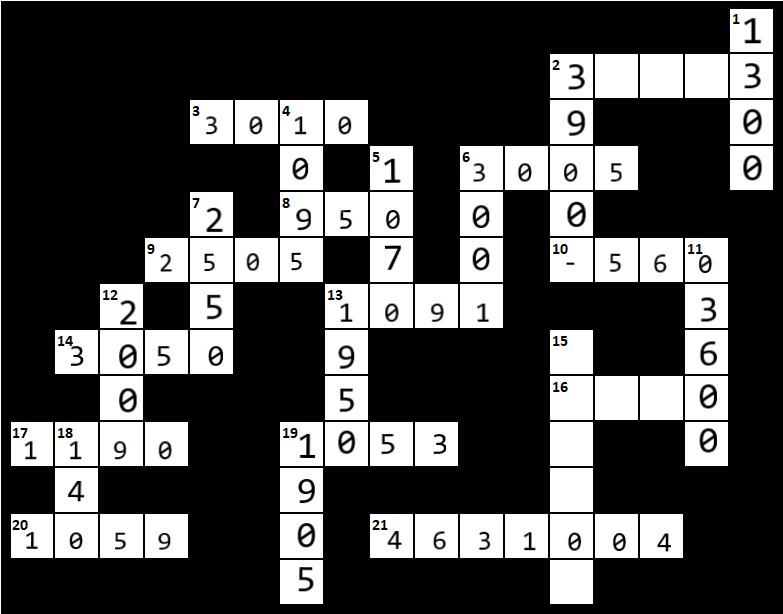
Across
2: Half of ({20 Across} x (smallest perfect number) + 446)
3: (Number of essentially different positions in the most popular futile game) x (only square that is one more than a prime number) - (number of cents in two quarters)
6: Radius of a circle with circumference = 18,881 (rounded to nearest integer)
8: Equals 500 + (x times y), where $3x + 2y = 120$ and $x - 5y = -215$
9: (Distance around an octagon with side = ((a perfect score in bowling) + (a baker's dozen))) + (number of even primes)
10: Opposite of ((basis of percentages) - ((double factorial of 10) - (the least common multiple of 1, 2, 3, 4, 5, 6, 7, 8)))
13: The Bin2Dec of 10001000011
14: (Every number from 1 to 100 added together) - (Floor(Year(Now()),100))
16: (Opposite of (sum of the first nine prime numbers)) + ((1130 in octal)$^2$/100)
17: Miles that equal 1915 km (rounded to the nearest integer)
19: (Number of ways to order a burger with 10 possible toppings) + (sum of the first four squares)
20: (Double any angle in an equilateral triangle) x (smallest integer which is neither prime nor semiprime) + (atomic # of Einsteinium)
21: (Opposite of where Fahrenheit = Celsius) x (sum of the squares of the first four primes) + (Michael Jordan's famous number)
Down
1: Weighted average of 1000 and 2000 where 2000 is weighted at 70% and 1000 is weighted at 30%.
2: Equals {19 Down} x (smallest prime number) + (latitude in degrees of the North and the South geographical poles)
4: (Inches in a foot) x (5B in hex) + (number of non-collinear points needed to determine a plane)
5: (Number of distinct Hamiltonian paths from cell A1 to cell A4 in a 4 x 4 grid) x (column ED in spreadsheet notation) - (basis of duality)
6: (Opposite of an even prime number) + (number of ways to choose 10 things from 15 things (without repetition))
7: Multiply (smallest sphenic number) and (4 score + (number of platonic solids))
11: Area of a rectangle with sides (sum of the first four pentagonal numbers) and (number of degrees in a right angle)
12: (Six factorial) x (number of triangle sides) - (twin prime of 149)
13: (Hypotenuse length of right triangle with sides 300 and 400) x (smallest composite number) - (number of states in the USA)
15: ((Rhombus edge count) x (dodecahedron edge count) x ((number of ways 4 items can be arranged) + 5)) + (number of hours in a day)
18: (All the values in an order-3 magic hexagon added together) - (smallest number that is the sum of two non-zero square numbers in two distinct ways)
19: Subtract (median of the set (77,81,94,96,98,99)) from (highest number expressible using only two unmodified characters in Roman numerals)
Hint #1:
The clue for the twist part is among the clues. Your work up to this point will not be in vain, but will need to have the twist applied to it.