Crossword, but math!

Question

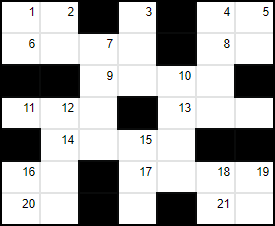
This kind of puzzle is from my mom's puzzle magazines. There it is called Figure Logic. It is essentially a crossword, but with math. In crosswords, all words must be real words. In Figure Logic, no numbers can start with 0. (EDIT) Also, all digits 0-9 appear.

XA/D means "X Across/Down". So 1 Across is half of 5 Down. Sorry about this!

Clues

Across
1. 5 down / 2
4. One digit is twice the other
6. 15 down + 20
8. Even
9. One digit is the sum of the others
11. Contains the only occurrence of a digit
13. Odd
14. Divisible by 1D
16. Divisible by 3
17. 5 x 10 down + 5
20. Consecutive digits in some order
21. Consecutive digits in some order

Down
1. Not prime
2. Non-consecutive digits
3. All digits are even
4. A perfect number - 2D
5. Divisible by 11
7. 13 across + 130
10. Living people were born this year
12. 15 down + 13 across
15. 1 across x 20 across
16. Can be written as a factorial
18. 4 across + 8 across
19. Prime

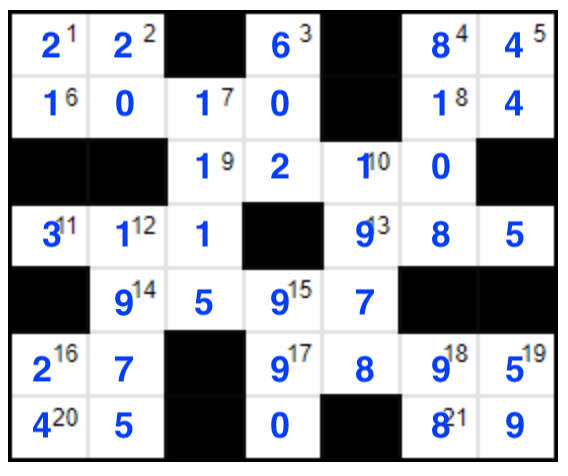
There is a unique solution which can be found without guessing. If you're confused about any definitions, please Google, or comment and I can explain. Also, calculators are allowed and likely required for some of the big numbers.

Any feedback is appreciated.

Answer 1

This was fun, thanks for posting it - hopefully my answer is correct!

Explanation:

16D = 24 since this is the only 2-digit number that can be written as a factorial, and 4D = 8xxx since 8128 is the only perfect number that is 4 digits, and since 2D is a two-digit number, the difference cannot be less than 8000.

Therefore: 4A = 84, 5D = 44, and 1A = 22.

Now if we look at 4D again, we can see that 8128 - 2D is between 8128 - 28 = 8100, and 8128 - 20 = 8108. Note that 4D ≠ 8128 - 29 = 8099 as this would result in 8A starting with the digit 0. So 4D = 810x which means that 8A = 14.

Therefore: 18D = 98. Also since 17A is a multiple of 5, it must end in the digit 5 or 0. Since 19D cannot start with the digit 0, it must start with the digit 5. Since 21A starts with an 8, it must be 87 or 89, but combining this with 19A since 59 is prime but 57 is not we have 19D = 59, and 21A = 89.

This next step is important! We have that 17A = xx95, so 5 x 10D = xx90, a 4-digit number ending in 90. Since multiplying by 5 is the same as multiplying by 10 and dividing by 2, 10D / 2 must end in the digit 9, meaning that 10D ends in the digit 8. So we have 10D = xxx8 but since 10D is a year living people were born in, 2018 and 2008 won't work since this would result in 13A starting with the digit 0. Therefore, 10D is a year in the 1900s, or 10D = 19x8. Using a calculator to check all of the possibilities for the third digit of 10D such that 17A = 5 x 10D + 5, we determine that 10D = 1978, and 17A = 9895.

Now we see that 20A is either 43 or 45, meaning that 15D is either 22 x 43 = 946, or 22 x 45 = 990. Using what we know about 17A, we can see 15D = 990 and thus 20A = 45.

Therefore: 6A = 1010, 1D = 21, 2D = 20, 4D = 8108.

Since 12D = 15D + 13A = 990 + 98x, the last digit of 13A is 5, making 13A = 985. Then 12D = 1975 and 7D = 1115. It also checks out that 1D = 21 is a multiple of 14A = 9597 (when I originally solved this problem, I was missing information for 1D and 14A, so I had to verify that 9x97 = 9597 was the only number of this form that was a multiple of a number in the twenties since I had only the first digit of 1D - oh well!).

Now since 9A = 1x10, in order for one digit to be the sum of the others, the last remaining digit is 2, making 9A = 1210. The only two remaining digits that haven't been used are 6 and 3. Since 3D = x02 is composed of all even numbers, we get 3D = 602, and 11D = x11 must be 11D = 311 satisfying the only occurrence of the digit 3.