Previous Level:- Lasers: Colour-Converters (Level 10)
Also before solving this, you must take a look at this level:- Lasers: Double-Sided Mirrors (Level 8)
This will be a more lengthy puzzle, here I will generalise puzzle 8.
Rules :
- There will be lasers that are shaped like an arrow. The arrows pointing in the respective direction shows where the laser goes and the colour shows the colour which it gives out.
- There will be boxes that are respectively coloured and these boxes need to get touched by the lasers in order to find a solution. In order to find the solution, you can make a move by rotating or moving the lasers or the mirrors, or moving the grey tiles or the coloured boxes 90° clockwise.
- A mirror reflects a laser's path in exactly 90° angle clockwise or counter-clockwise, depending on the path. Double-sided mirrors can reflect 2 laser paths in 2 particular directions.
- Each box should receive the light of one single laser. In future levels, a box may receive the light of 2 or more lasers.
- Lasers(the arrows of the lasers) and Grey Tiles, along with the sides of the Mirrors would block other lasers' paths.
- (Bridges)/(Doubled-Bridges) have a specific colour to allow lasers to cross through a box from a particular direction from the same colour, or else it would block lasers from coming through other directions as well as lasers with different colours. Bridges cannot be rotated but in future levels, they may be rotated.
- Brown tiles (or tiles surrounded by brown lines) can neither be rotated nor be moved, they will be static.
- You can move objects (like lasers, mirrors, grey-tiles, bridges, etc.) such that they move as far as possible in the grid in a particular direction until they reach the edge, or they collide with another piece. Brown objects cannot be moved.
- Blue lines allow the movement of all pieces, but blocks lasers' paths, typically the same thing what a grey/brown tile does.
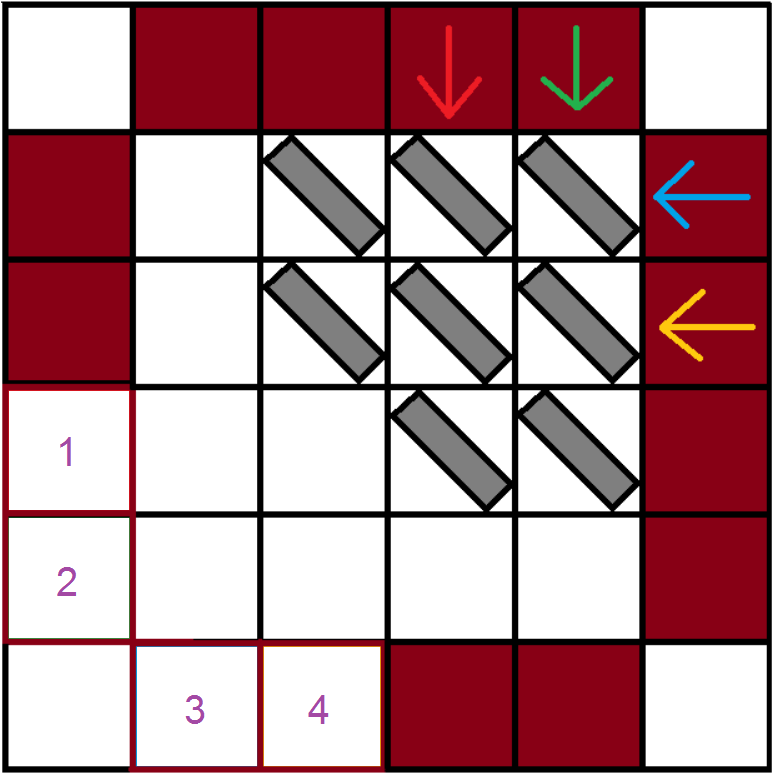
This was the picture of puzzle 8. Instead of the coloured boxes, only the numberings are done. Just to make sure, in puzzle 8 we solved the part when {1,2,3,4} = {red , green , blue , yellow}.
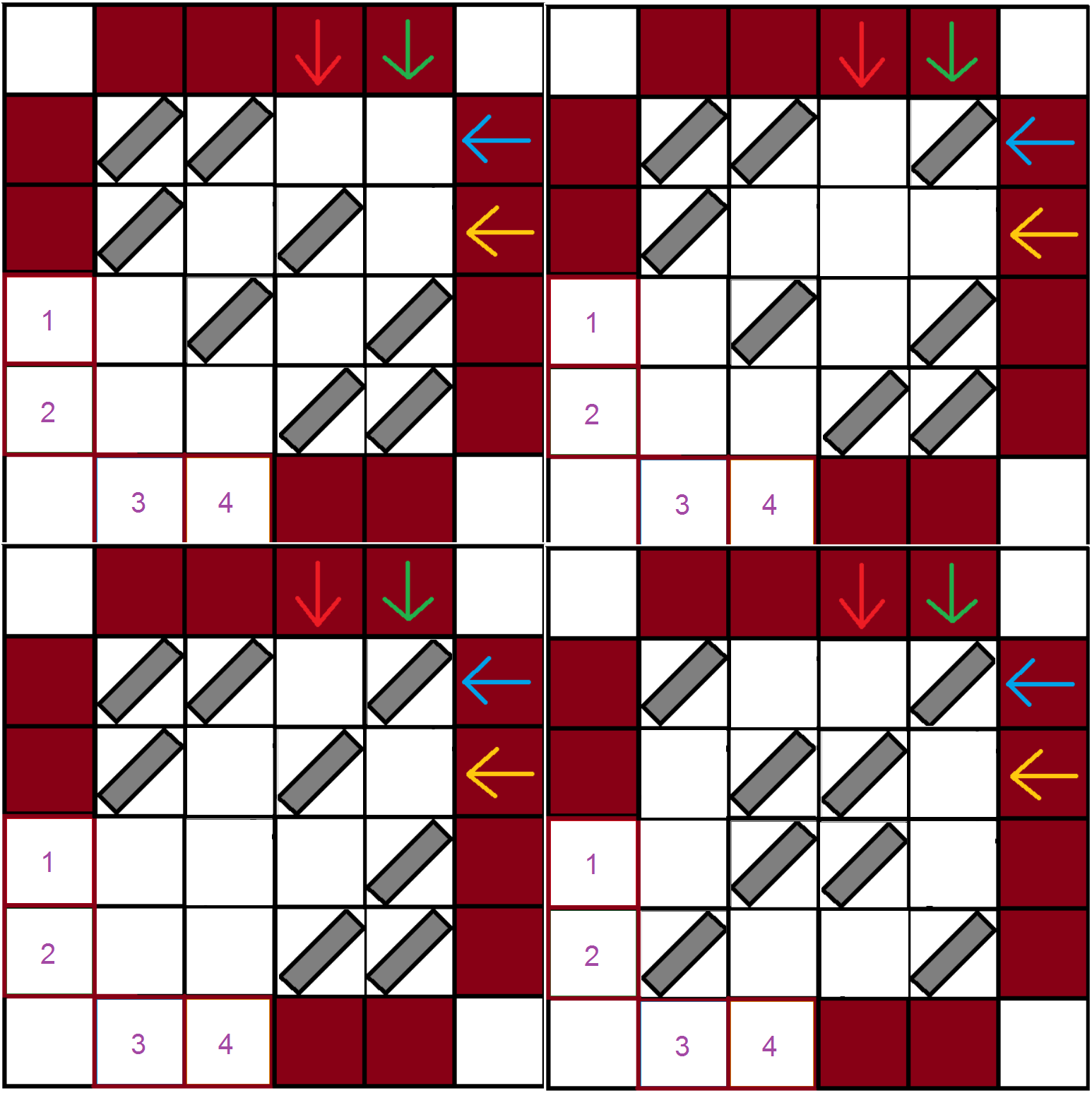
Goal:- Suggest or Try to find a suitable way to make sure that the other configurations of colours of {1,2,3,4} are solvable in this puzzle or not. (For example, try to find if {1,2,3,4} = {blue , green , red , yellow} is solvable or not}). There are total 4! configurations, so we only need to check 23 of them. Note that there must be an argument to show the claim.