9
$\begingroup$

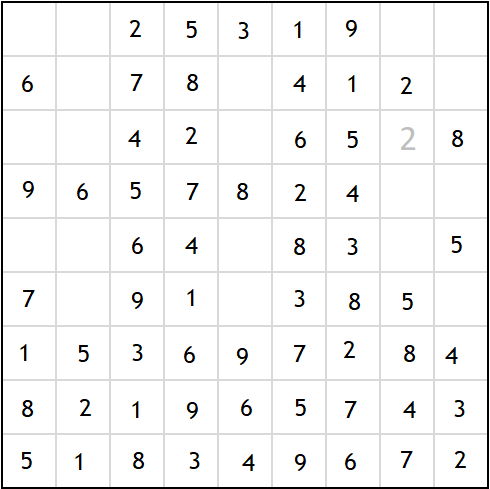
An entry in Fortnightly Topic Challenge #47: "Wacky Sudokus"

Other puzzles in this series

Welcome to the twelfth puzzle in this series! For more information about the series, see the first puzzle and the introduction. Enjoy!

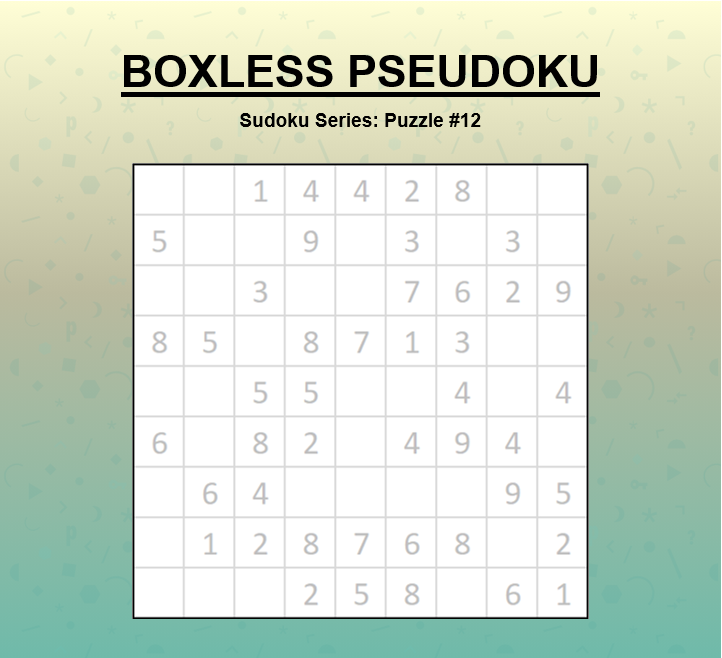
             enter image description here

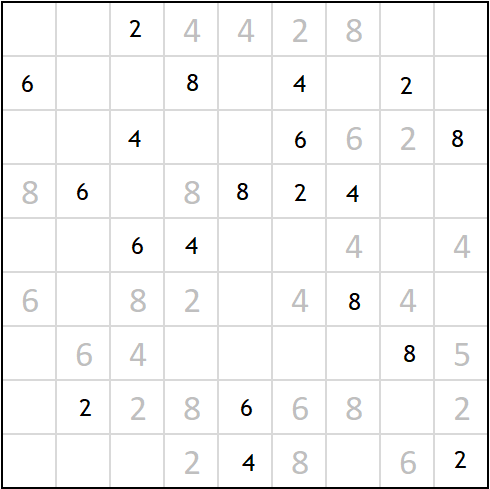
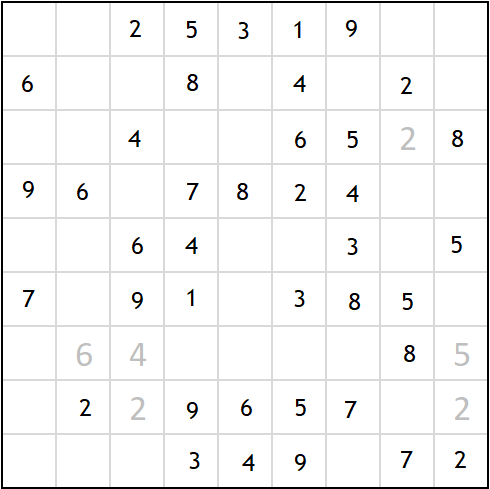
I got bored of giving you actual clues, so here are some fake ones

Google Sheets Link

RULES:

  • Normal Sudoku rules apply
    • However, there are no boxes in this grid, just rows and columns
  • The numbers given are incorrect, the actual entry is either one more or one less than the given number

Only three left! Hopefully everyone has enjoyed so far, there are still 2 more to be released in the next 2 days which are quite interesting ones. Good luck!

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ Alright so since the boxes are missing, we only need to fill this sudoku given the condition that all the rows and columns only have each number from 1 to 9, am I right? $\endgroup$
    – Anonymous
    Commented Jan 21, 2021 at 17:46
  • $\begingroup$ @Anonymous correct, only the rows and columns need that $\endgroup$
    – Beastly Gerbil
    Commented Jan 21, 2021 at 18:01

1 Answer 1

Reset to default
10
$\begingroup$

COMPLETED GRID

enter image description here

REASONING

To begin:

Any pseudo-clues that are 1s or 9s must actually be 2s and 8s, respectively. Then any 3s in a row/column with an actual 2, and 7s in a row/column with an actual 8, must resolve to 4s and 6s, respectively. Then we can repeat with existing clues to see what's forced, and in fact we're able to fill in almost all of the odd pseudoclues in this manner. The grid at this stage:

Progress

A deduction:

In the top row, the two pseudo-4s must resolve to 3 and 5 respectively, forcing the 2 in that row to resolve to a 1. The exact same situation occurs in row 6. Similar logic in row 8 forces the 6 pseudoclue there to resolve to 5. We can then chase the implications of this to get almost all the remaining clues. The grid thus far:

Progress

Moving into the unshaded squares:

In row 8, the remaining 2 pseudoclues are 1 and 3, meaning the unfilled squares are 4 and 8, which we can place. In column 6, we can also place the missing 7 and 8. Placing the 7 allows us to resolve most of the remaining pseudoclues at the bottom, except the 5 in R7C9. Sudoku rules resolve columns 3, 4 and 7, and then rows 7-9. The grid thus far:

Progress

The rest of the grid is just Sudoku fill-ins.

Improve this answer
$\endgroup$
4
  • $\begingroup$ Woahhhhh that was quick! I didn't expect this to be solved quite that quick even if it was quite easy :P $\endgroup$
    – Beastly Gerbil
    Commented Jan 21, 2021 at 18:02
  • $\begingroup$ @BeastlyGerbil: was definitely a neat puzzle...I was just lucky to see the little flap of logic pretty early on :-) $\endgroup$
    – Jeremy Dover
    Commented Jan 21, 2021 at 18:05
  • $\begingroup$ I didn't expect the logic too hold up too long, especially considering that I've done a Pseudoku before (linked) so people probably already know the tricks. Now to see if I can try and solve yours, because right now its giving me a headache :P $\endgroup$
    – Beastly Gerbil
    Commented Jan 21, 2021 at 18:07
  • $\begingroup$ @BeastlyGerbil I don't think it's giving a hint to say that my puzzle is a tough nut. My QC test solve took me about 5 hours. Granted, I wrote down EVERY logical step to make sure the solution was unique. But still... $\endgroup$
    – Jeremy Dover
    Commented Jan 21, 2021 at 18:10

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.