The Blind Killer - a new series! SS#1

Question

^{An entry in Fortnightly Topic Challenge #47: "Wacky Sudokus"}

Other puzzles in this series

---

Welcome to a new sudoku series! This series will run for the duration of the current Fortnightly Topic Challenge: 'Wacky Sudokus'.

For the past month I have created 14 different sudokus, all with a unique twist. I will post one a day until the end of this FTC.

Each puzzle will consist of a picture of the puzzle, a google sheet version and a set of rules. Clicking on the photo will give a higher quality version of the grid. For any clarification about anything ask in the comments and I'll try and help!

I won't add this introduction at the start of every puzzle, but will instead link this puzzle as well as the FTC.

The puzzles will be of varying difficulty, some will be fairly easy, others much harder. I'll accept a solution when I post the next puzzle in the series. A solution will be accepted which shows:

• the completed grid
• some explanation (preferably on how you started and anything you found hard/interesting)

I hope this will be an enjoyable mini series for everyone. So, without further ado, here is the first puzzle!

Bonus: At the end of the 14 puzzles, I will award a +50 bounty to the 'best' answer, the one that I think best displays the solution path, logic involved, is easy to read and overall looks the best!

---

         

---

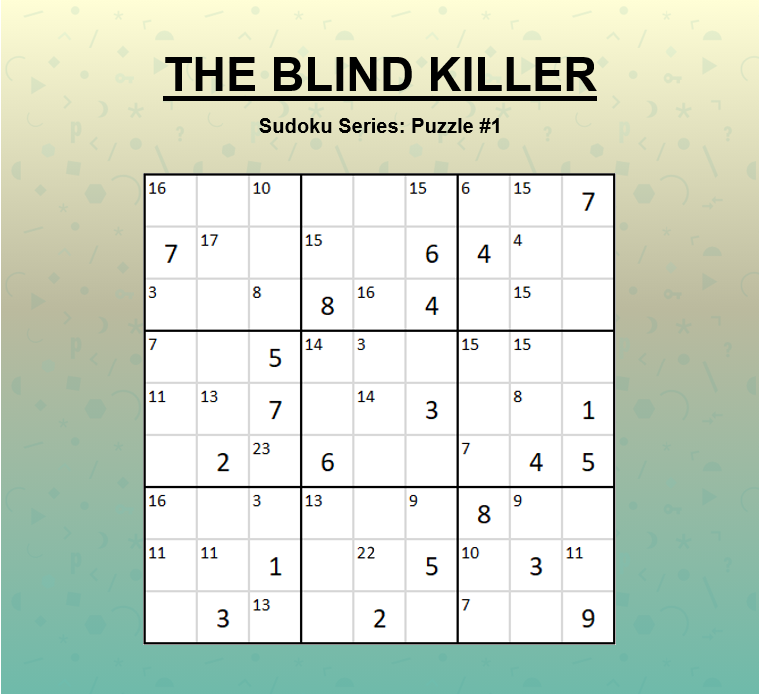
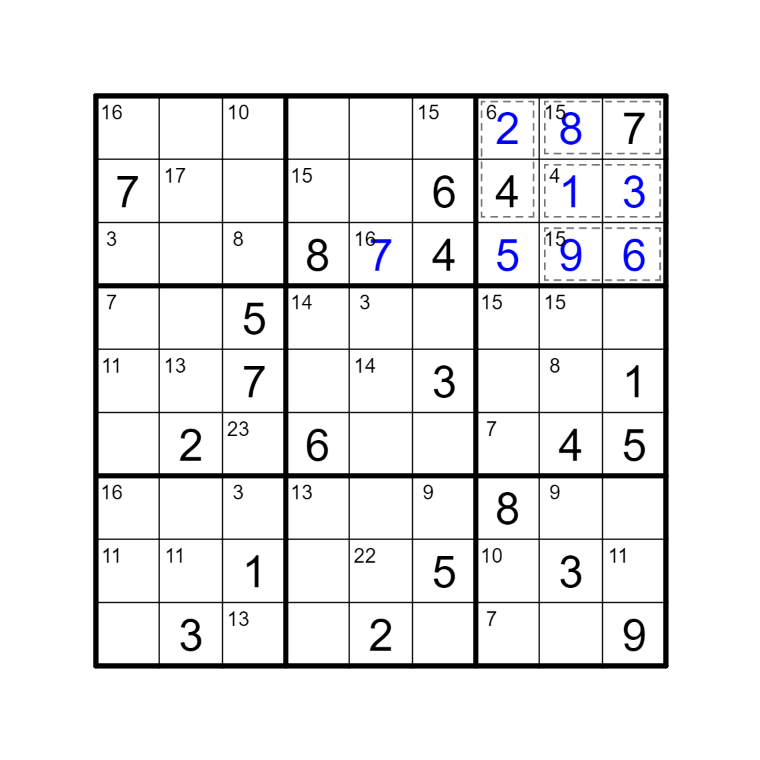
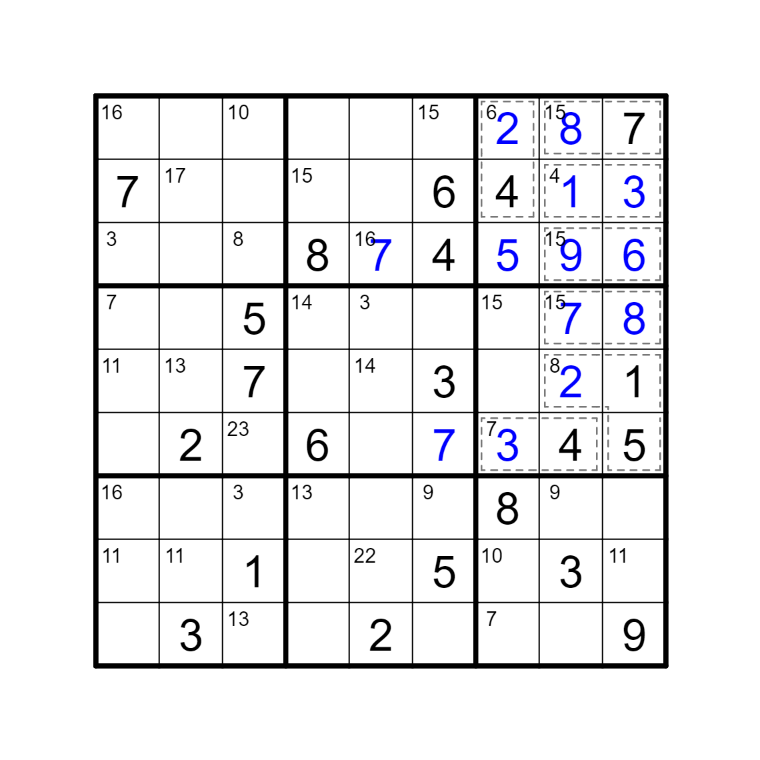
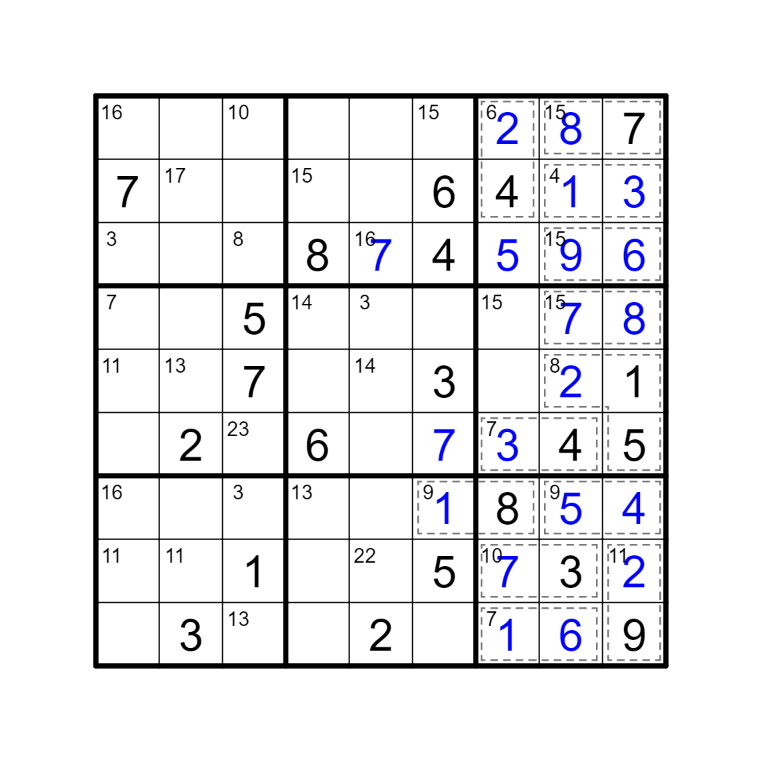
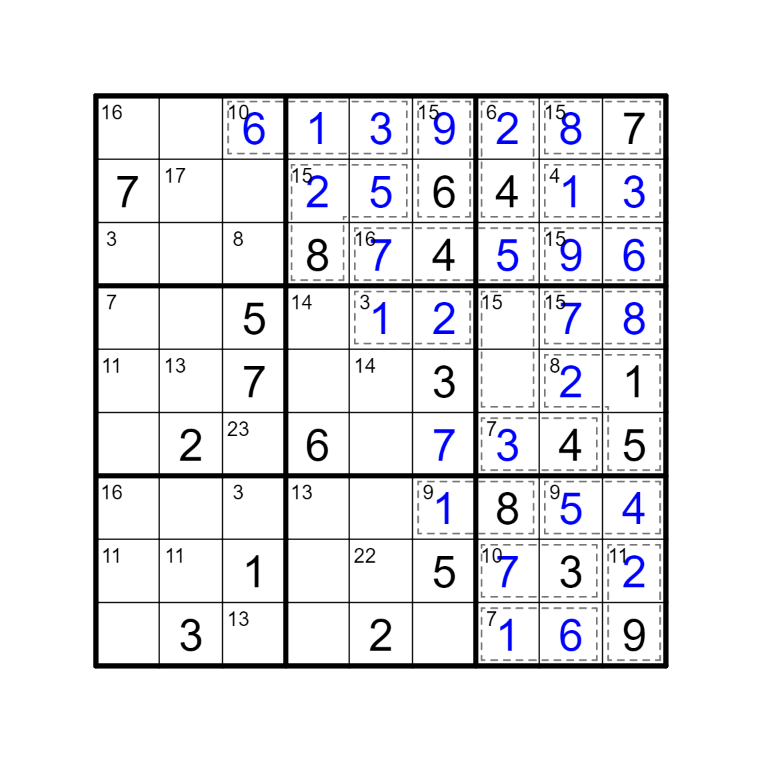
This killer sudoku appears to have the regions missing... looks like you'll have to work out where these regions are too!

Google Sheets Link

RULES:

• Normal Sudoku rules apply
• The grid is also separated into regions of 2 or 3 cells. The sums of all the regions are given as the smaller text. The sum is displayed in the top left (top then left) cell of each region
• The same digit may not appear twice in the same region

Good luck!!!

Answer 1

Step 1:

First, place '7' in R3C5 due to Sudoku rules. Then, focus on the upper right 3 x 3 square. The '4' killer sum can only extend to the right and it can only be made from (1 + 3). Since there is already a '1' in C9 and a '3' in C8, we can fill '1' in R2C8 and '3' in R2C9. Then, this also forces the '15' killer sum just above to extend to the right (since killer sums must appear at the top left of their group). Therefore, R1C8 is '8'. Then, notice that the '15' killer sum in R4C8 can only extend to the right. This must mean that the '15' killer sum in R3C8 must comprise of 2 cells, and the digits must be '6' and '9'. Due to the '9' in C9, we know '9' is in R3C8 and '6' in R3C9. This leaves '5' for R3C7.

The grid at this point:

Step 2:

Next, we focus on the middle right 3 x 3 square. The '8' killer sum must extend to the right. Therefore, the '15' killer sum just above it must comprise of only 2 cells and the sum must be 7 + 8 (since it cannot be (9+6)). 7 must be in R4C8 and 8 in R4C9. Then, the '7' killer sum in R6C7 can only be completed by extending to the right and including '4'. This means R6C7 is a '3'. The '15' killer sum in R4C7 must extend downwards and comprise of only 2 cells, so its sum must be (9 + 6). This leaves '2' for the '8' killer sum.

The grid at this point:



Now, focus on the lower right 3 x 3 square. The '11' killer sum can only extend downwards, which means R8C9 must be a 2. C7 is only missing a '1' and '7' for the bottom two cells. '7' cannot go in R9C7, so it must be in R8C7. The '7' killer sum in R9C7 must extend right, so R9C8 must be a 6. This means that the '9' killer sum in R7C8 must be (5 + 4). The '8' in R7C7 must be part of the '9' killer sum in R7C6. The grid at this point:

Step 3:

Now, focus on the top. The '5' in R3C7 must be part of the '16' killer sum in R3C5, which nicely completes it. This means the '15' in R1C6 must be completed by including the 6 below it, making R1C6 a '9'. Calculating the 2 '15' killer sum + the '7' and the '4' in the top middle 3 x 3 square sum comes to 41, so the sum in R1C4 + R1C5 must be 4. This must therefore be 1 and 3. The '15' in R2C4 must therefore extend down and right. Its sum must come from (2 + 5 + 8), and since there is a '2' in C5, 2 must go in R2C4 and 5 in R2C5. Next, look at the '3' killer sum in R4C5. Its sum must be (1 + 2) and since there is already a '1' in C6, we can resolve this. This also helps to resolve the placement of 1 and 2 in R1. Then, the cells R1C4 and R1C5 must be part of the '10' killer sum, which means R1C3 is a 6. The grid at this point:



Then, focus on the middle left. The '3' in R3C1 can only be resolved with (1 + 2) and since there is a '2' in C2, 2 must be in R3C1 and 1 in R3C2. The '8' killer sum in R3C3 can only extend downwards and R3C3 must therefore be a 3. Then, R4 is missing a '6' and a '9'. Since the middle square contains a '6', R4C4 must be a '9'. Then, '14' killer sum in R4C4 can only extend downwards and R5C4 must be a '5'. This also resolves the '6' and '9' ambiguity in R4C7 and R5C7. Then, the '13' killer sum in R5C2 must extend to the right and include the '7'. Therefore, R5C2 must be a '6'. '9' cannot be a part of the '11' killer sum and must appear in R6C3. This leaves '8', '1' and '2' for the '11' killer sum, which can be resolved since there is already a '1' in R5. The grid at this point:

Step 4:

The killer sums in the top left square can both be resolved via sudoku rules. Then, the '23' killer sum in R6C3 can only be resolved by extending to the right twice. Since it already contains a '9' and a '6', the last number must be '8'. This also resolves the '14' killer sum in R5C5. '8' must appear in R9C6 and this '8' must be a part of the '22' killer sum, so this places a '9' in R8C5. The '3' in R7C3 must be resolved by including the '1' below it, so R7C3 must be a '2'. The grid at this point:



Then, the '16' killer sum in R7C1 can only extend downwards and must be (9 + 7). 9 already appears in C2, so 9 is in R7C1 and 7 in R7C2. Then, the '11' in R8C2 can only be resolved by extending downwards and including the '3', which means R8C2 is an '8'. The remaining cells can then easily be deduced via Sudoku rules. The final grid: