4
$\begingroup$

I was thinking about ring-ring puzzle. Suddenly, the 'word' rong-rong came to my mind and made me laugh for no reason. So, I'm messing around with ring-ring rules that fit the rong-rong 'word'.

Rules: Fill the empty cells of the grid by drawing rectangles that consist of horizontal and vertical lines between cells' centers. The sides of different rectangles may intersect, but not overlap. Corners of rectangles may not touch. Every rectangle that intersects with another rectangle must be a square shape.

The Puzzle:

rong-rong puzzle

Improve this question
$\endgroup$
4
  • 1
    $\begingroup$ My reading is that they aren't allowed to share sides, but their sides can intersect at right angles -- but only if both rectangles are actually squares. (And that when one rectangle is nested inside another that doesn't count as "intersecting" because it's the outlines of the rectangles that we care about.) $\endgroup$
    – Gareth McCaughan ♦
    Commented May 9, 2022 at 1:05
  • $\begingroup$ @bobble A rectangle called intersect another rectangle when that's rectangle's side cross another rectangle's side, just as Mr. Gareth said. $\endgroup$
    – Nusi
    Commented May 9, 2022 at 7:31
  • $\begingroup$ And the goal is to make every square to have a line, either a corner or a straight through, or a cross? $\endgroup$
    – justhalf
    Commented May 9, 2022 at 10:59
  • $\begingroup$ @Gareth McCaughan Thank you for posting that comment! I had the conditions backwards and spent a few hours yesterday fooling around with it. Once I understood it correctly, it wasn't nearly as difficult. $\endgroup$
    – JLee
    Commented May 9, 2022 at 12:41

2 Answers 2

Reset to default
2
$\begingroup$

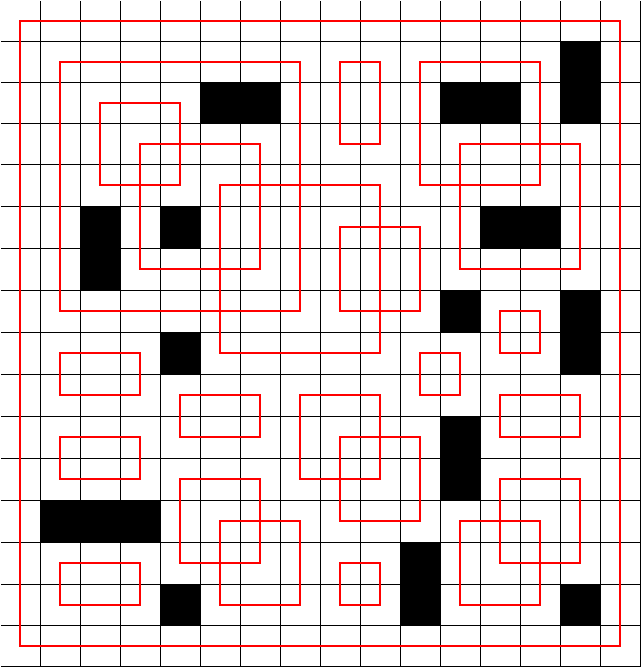
Solution:

Step-by-step:

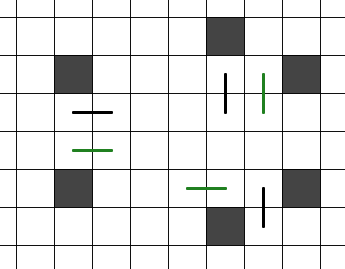
Let us introduce some basic logical steps. Since cells next to a wall cannot contain intersections, we can deduce the green lines from the black lines in the following picture

With that in mind, we can make the following initial deductions

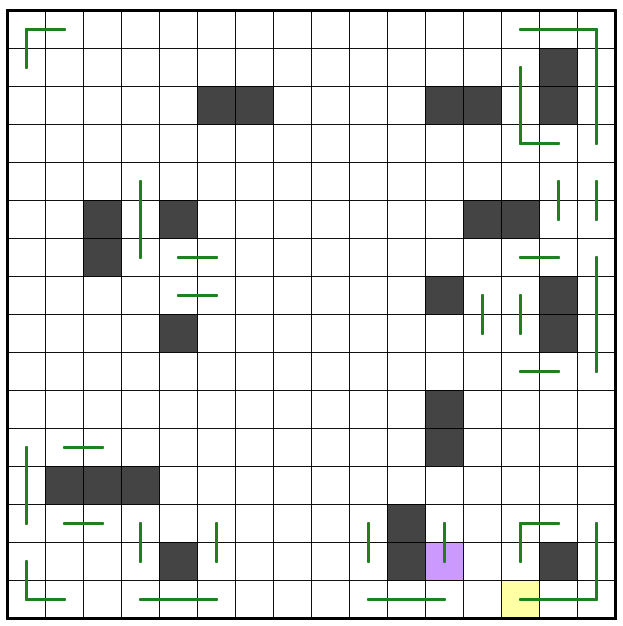
Next, let us look at the yellow cell in the above picture. If it continued upwards, the cell next to it would also have to be a corner from left to up and, consequently, the purple cell could not be continued legally. Therefore, the yellow cell must continue straight and we can make some more easy deductions based on the square requirement of intersections.

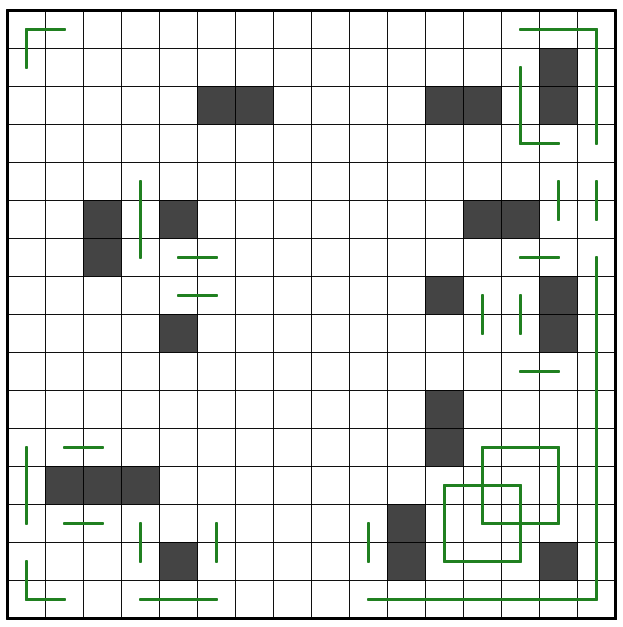
We can make some more easy deductions on the right side as well as the lower left.

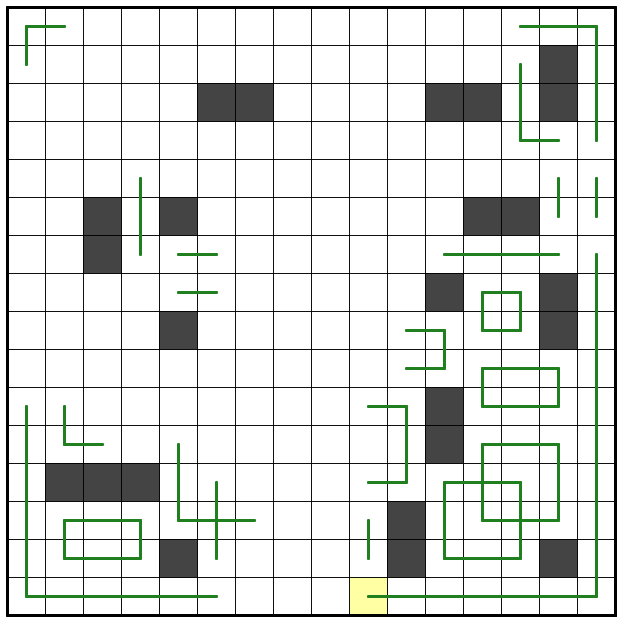
Now, we cannot continue up from the yellow cell, because we would be forced to have an intersection with that rectangle and it is not square. Therefore that cell must continue straight and the cell above it must turn left. Again, we can make the same deduction for the cell on the left of the yellow cell, and it must continue straight again.

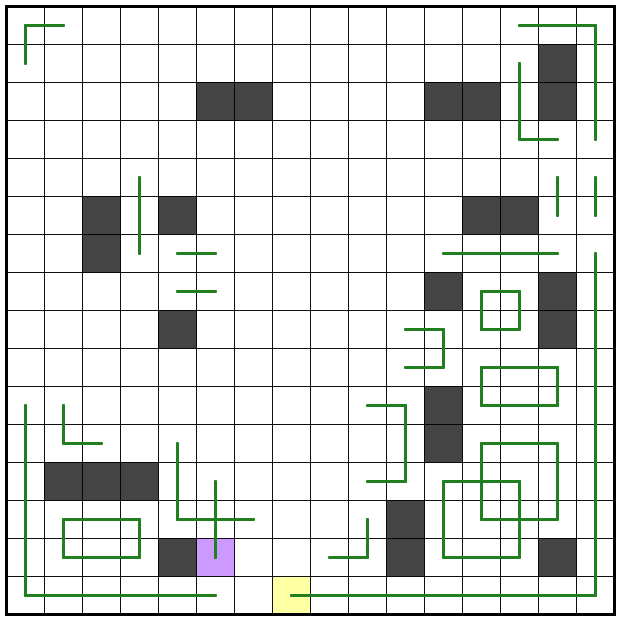
Again, the yellow cell cannot continue up, because then the cell on its left would also have to be a corner from left to up and there would be no way of legally continuing the rectangle with the purple cell. However, it also cannot turn up from the next cell because it would immediately force either the rectangle above the yellow cell or the rectangle above the purple cell to share sides (or corners) with other rectangles. Therefore the whole bottom row is part of the same rectangle. Now, this big rectangle is actually forced to go through the whole boundary because otherwise it would have to intersect with other rectangles. With that, we can also make some other easy deductions.

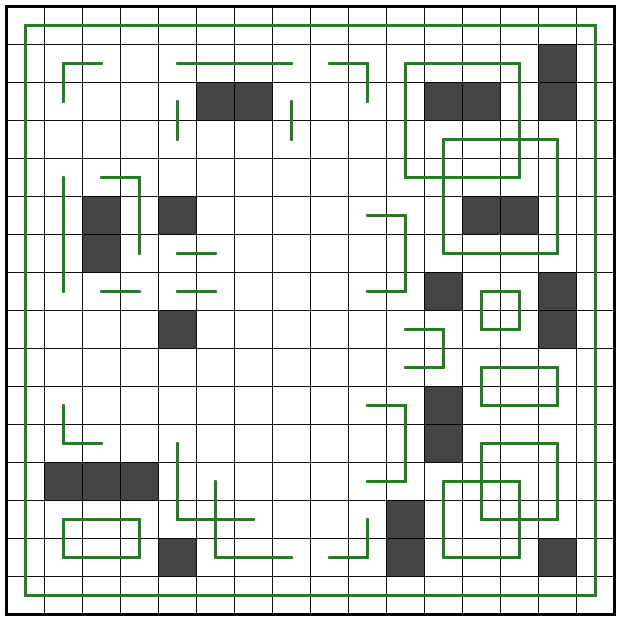
And some more
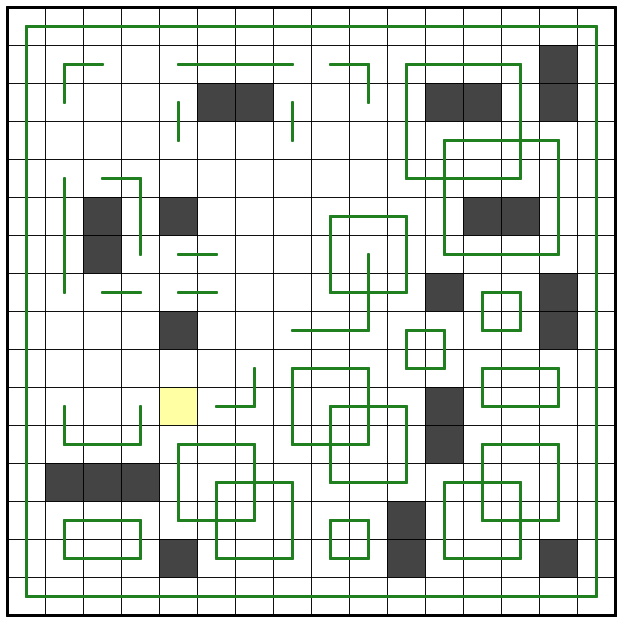
Now, if we look at the yellow cell, it cannot go straight horizontally because there would be no way of forming a square of the rectangle. Therefore, it must go up and since the rectangle must be two cells tall, it cannot intersect to the left but must instead go right.

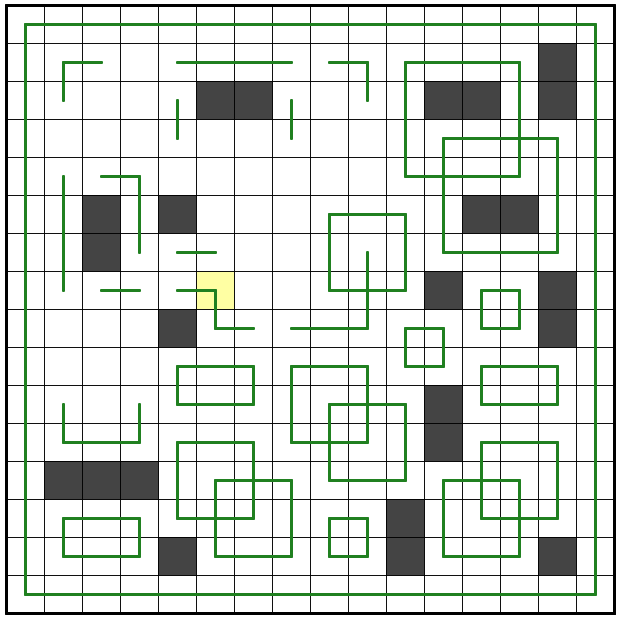
The yellow cell in the above picture must be an intersection, which forces some bigger squares.

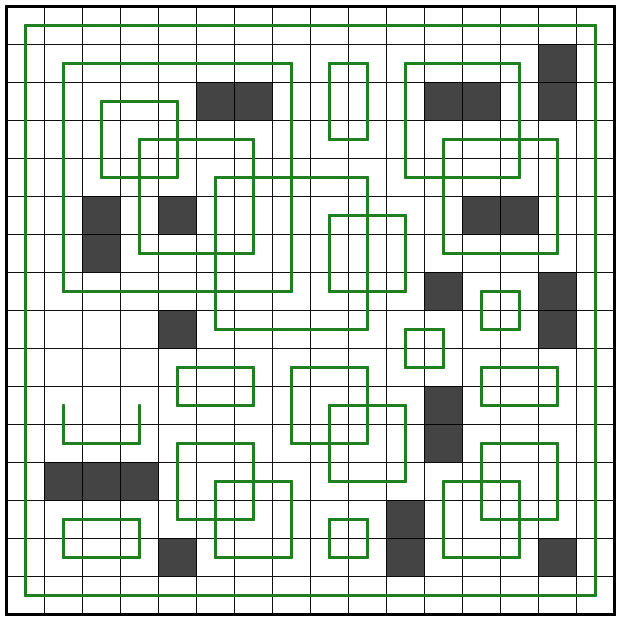
It is easy to find the only way to fill the remaining area with two rectangles, which gives the solution.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Great step-by-step explanation. +1 Maybe I intuitively sensed some of those rules, or maybe I just got lucky, but I stumbled upon the answer in 10-15 minutes. $\endgroup$
    – JLee
    Commented May 9, 2022 at 13:00
  • $\begingroup$ Yes. How did you get the initial black lines? $\endgroup$
    – Parcly Taxel
    Commented May 9, 2022 at 17:19
  • $\begingroup$ @ParclyTaxel The picture with the black lines is just an example that is not related to the actual puzzle. For the first deductions with the actual puzzle, we get the trivial lines in the corners and anywhere else with only two neighbours, and from those the next step is the lines similar to the example picture. $\endgroup$
    – user39583
    Commented May 9, 2022 at 19:00
0
$\begingroup$

If I understood the rules correctly, this is one possible answer:

enter image description here

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.