Queens of the Square Sudoku Table

Question

 .  6  . | 8  .  3 | .  7  . 
 .  .  1 | .  .  . | .  6  9 
 7  .  . | .  .  . | .  .  5 
---------+---------+--------
 .  .  . | 9  .  . | .  1  . 
 .  .  . | .  .  . | .  .  4 
 .  .  5 | .  1  . | .  .  . 
---------+---------+--------
 5  4  . | .  8  . | .  .  7 
 .  .  . | 5  .  . | .  .  . 
 .  9  7 | 3  .  . | .  .  . 

• Normal Sudoku rules apply
• If the 2's were queens on a (9 by 9) chessboard, they would be unable to capture each other

Answer 1

Solution

  9  6  4 | 8  5  3 | 2  7  1 
  3  5  1 | 2  4  7 | 8  6  9 
  7  2  8 | 6  9  1 | 3  4  5 
 ---------+---------+--------
  4  8  3 | 9  2  5 | 7  1  6 
  6  1  9 | 7  3  8 | 5  2  4 
  2  7  5 | 4  1  6 | 9  8  3 
 ---------+---------+--------
  5  4  2 | 1  8  9 | 6  3  7 
  1  3  6 | 5  7  2 | 4  9  8 
  8  9  7 | 3  6  4 | 1  5  2 
 

Methodology

The first thing to realise is that

with pure Sudoku deductions, we can't get very far at all. I was hoping to be able to solve it like a normal Sudoku with the occasional extra deduction of the form "2 can't be there because then it would share a diagonal with another 2", but as a pure Sudoku I don't think we have enough information even to find a single 2.

I got as far as the following

using pure Sudoku deductions:

  .  6  . | 8  5  3 | .  7  . 
  .  5  1 | .  .  . | .  6  9             247 in middle, 38 outer
  7  .  . | .  .  . | .  .  5             169 in middle
 ---------+---------+--------
  .  .  . | 9  .  . | .  1  . 
  .  .  . | .  .  . | .  .  4 
  .  .  5 | .  1  . | .  .  . 
 ---------+---------+--------
  5  4  . | .  8  . | .  .  7 
  .  .  . | 5  .  . | .  .  . 
  .  9  7 | 3  .  . | .  .  . 
 

(There are a couple of extra notes on the right which narrow down the possibilities for some cells. In the 2nd row, 3 and 8 must be in the left and right boxes with 2, 4, 7 in the middle box; then the three cells in the 3rd row and middle box must be 1, 6, 9 in some order.

The next thing to realise is that

in placing the 2s in this puzzle, we're essentially solving an N queens puzzle with $N=9$. That means there are only a limited number of possible solutions. Specifically, 46 possible configurations plus their various rotations and reflections, or 352 total configurations. Of course the non-2 numbers that we have already placed will cut down those possibilities a lot, but that would still be a lot of checking to do, and I couldn't even find a clear list of the 46 fundamental configurations.

The next step involves an assumption made in hope, namely that

one of the 2s will be at the corner of the whole board. Because then, excluding the row and column for that corner, we have an 8x8 board which forms the classical eight queens problem, all of whose fundamental solutions are listed on Wikipedia.

Checking through those, we see that

keeping the Sudoku rules in mind (specifically, exactly one 2/queen in every 3x3 box), only a few of those twelve 8x8 configurations will give a valid 9x9 configuration. Namely: number 4 with another queen at the top left, number 5 with another queen at the top left, number 5 with another queen at the bottom right, number 9 with another queen at the top right (numbers as in the Wikipedia list). This gives respectively:

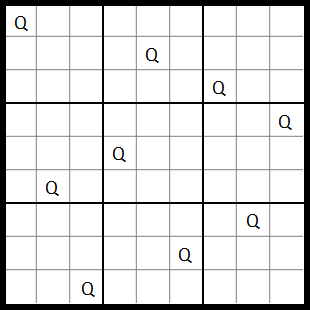
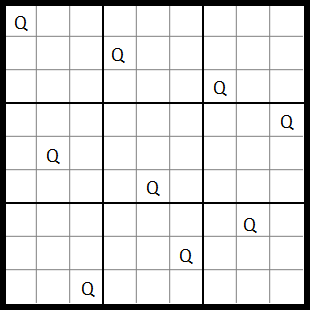
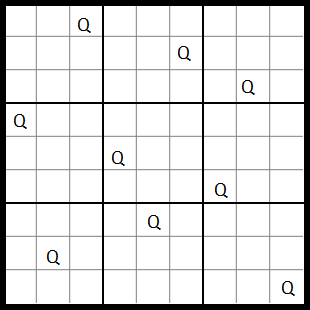
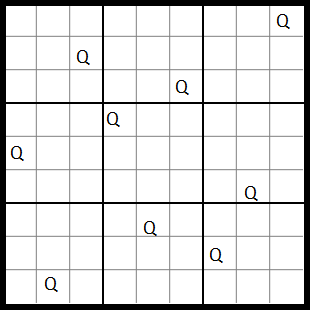
Checking these with the board we've got so far, we find that

none of them is possible, in any rotation, except the second one with a 180 degree rotation, namely:

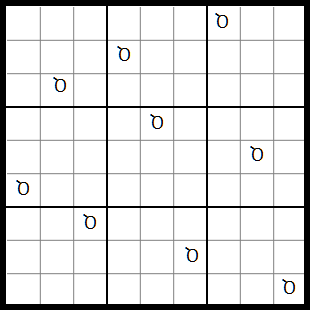
Using this, the board becomes

  .  6  . | 8  5  3 | 2  7  . 
  .  5  1 | 2  .  . | .  6  9             47 in middle, 38 outer
  7  2  . | .  .  . | .  .  5             169 in middle
 ---------+---------+--------
  .  .  . | 9  2  . | .  1  . 
  .  .  . | .  .  . | .  2  4 
  2  .  5 | .  1  . | .  .  . 
 ---------+---------+--------
  5  4  2 | .  8  . | .  .  7 
  .  .  . | 5  .  2 | .  .  . 
  .  9  7 | 3  .  . | .  .  2 
 

which can then be solved like a normal Sudoku.

Uniqueness?

I don't actually know if this solution is unique, because I haven't gone through all possible nine-queen configurations. I do know that the Sudoku solution is unique, starting from the last partially filled board shown above.

Feedback section

Great puzzle! You've got two well-known but different puzzles playing into each other in a very interesting way. In hindsight it seems like an "obvious" combination to make, since both Sudoku and queen puzzles involve a configuration of things on a square board which can't share rows or columns with each other, but I don't think I've seen this combination done before, and it was very non-obvious to solve.

Answer 2

Solving this problem with my own solver produced 24 solutions. Two more in addition to the one in the accepted answer are

   9  6  2 |  8  5  3 |  4  7  1
   8  5  1 |  7  4  2 |  3  6  9
   7  3  4 |  6  9  1 |  8  2  5
 ----------+----------+---------
   4  2  8 |  9  7  6 |  5  1  3
   6  1  9 |  2  3  5 |  7  8  4
   3  7  5 |  4  1  8 |  6  9  2
 ----------+----------+---------
   5  4  6 |  1  8  9 |  2  3  7
   1  8  3 |  5  2  7 |  9  4  6
   2  9  7 |  3  6  4 |  1  5  8
 
 
   9  6  2 |  8  5  3 |  4  7  1
   3  5  1 |  7  4  2 |  8  6  9
   7  8  4 |  6  9  1 |  3  2  5
 ----------+----------+---------
   4  2  8 |  9  7  6 |  5  1  3
   1  7  9 |  2  3  5 |  6  8  4
   6  3  5 |  4  1  8 |  7  9  2
 ----------+----------+---------
   5  4  6 |  1  8  9 |  2  3  7
   8  1  3 |  5  2  7 |  9  4  6
   2  9  7 |  3  6  4 |  1  5  8
 

The solution will be unique if, e.g., two additional numbers are added in the second-to-last row of the sudoku:

 .  6  . | 8  .  3 | .  7  . 
 .  .  1 | .  .  . | .  6  9 
 7  .  . | .  .  . | .  .  5 
---------+---------+--------
 .  .  . | 9  .  . | .  1  . 
 .  .  . | .  .  . | .  .  4 
 .  .  5 | .  1  . | .  .  . 
---------+---------+--------
 5  4  . | .  8  . | .  .  7 
 .  .  . | 5  7  . | .  .  8 
 .  9  7 | 3  .  . | .  .  . 

Then the solution is exactly the one in the answer by Rand al'Thor.