(Here is an experimental Sudoku created by me, however, the "box" constraint is not originally mine, but it'd be fun to try out this constraint.)
- Normal Sudoku rules apply.
- Additionally, each 1x3 (or 3x1) box either contains the numbers
(1,4,7),(2,5,8), or(3,6,9)in any order.
(click for a higher resolution)
Step 1:
For starters, we can make the following deductions.
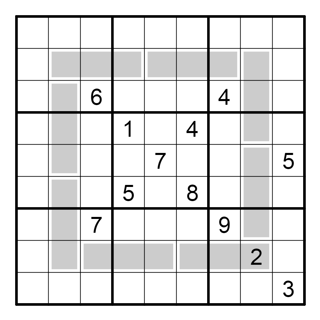
The 1 x 3 group in row 8, columns 6-8 can deduced immediately due to the '8' in R6C6. As a result, we can also place a '5' in R9C8 and an '8' in R1C8, due to the fact that they cannot appear in the 1 x 3 groups in column 8. 7 must belong to the 1 x 3 group in row 8, columns 3-5, and it must be in R8C4 due to '7' already in column 5. The '147' group must the 2nd 1 x 3 group in column 8, because the '4's eliminates any possibility of it being in the first. This allows for the placement for '7' in R6C8.
Step 2:
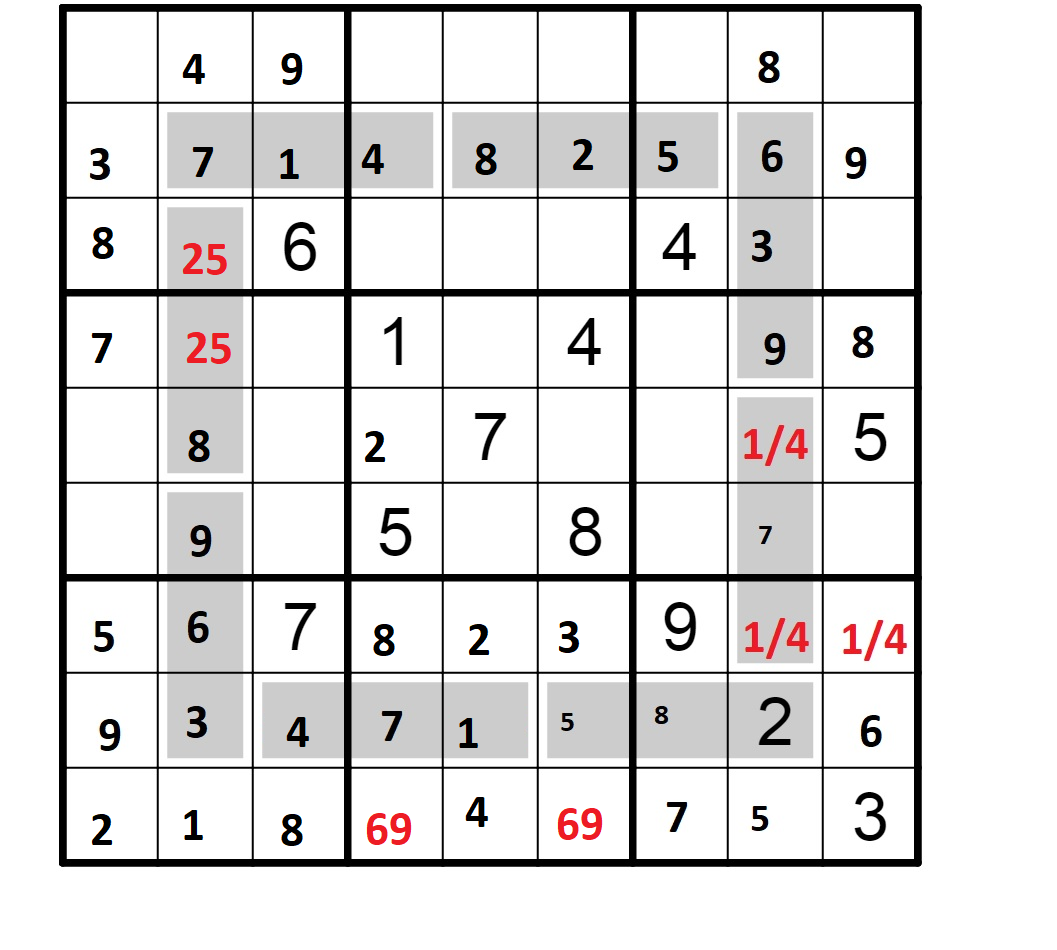
Next, we can fill in cells where there is only one possibility (8 in R4C9, 5 in R7C1, 7 in R9C7). 6 can be placed in R8C9, because there isn't a '369' group in row 8 and 3 and 9 are already in the bottom right square. Now, note that there can't be a '369' group horizontally in row 2. This means the square in R2C9 must be 3,6 or 9, but since 3 and 6 are already placed, it must be a 9. In R2C1, it must be a '3' since 6 is already there. The knock-on deduction gets us to:
Step 3:
At this point, I got stuck. However, I noticed that the '147' and '258' groups must appear somewhere in row 2. Depending on which one is the first group horizontally in that row 2, the places for the 1 x 3 cells in column 2 can be deduced as well. So, I decided to try that '147' group must appear in the 2nd '1 x 3' cell in row 2. (If this results in a contradiction, then we will know '258' should appear in that group instead). Making this try gives us the following grid based on process of elimination.:
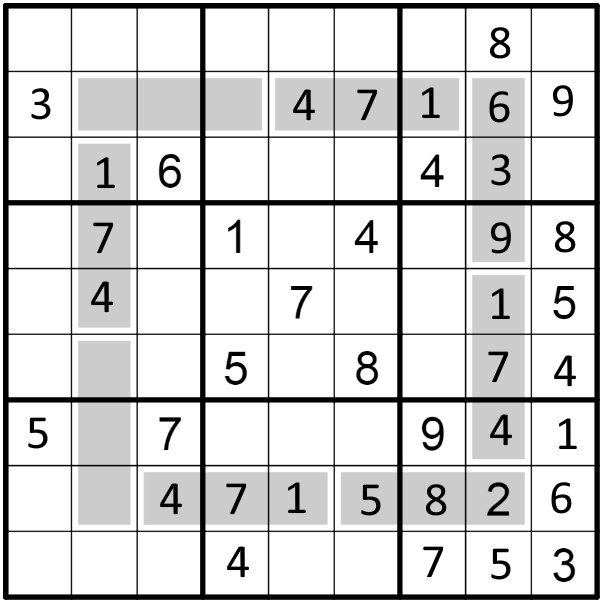
Step 4:
Next, using the grid before, we can use process of elimination to place some more 1,4,7 and in the process also solve for the 1st 1 x 3 group in row 2.
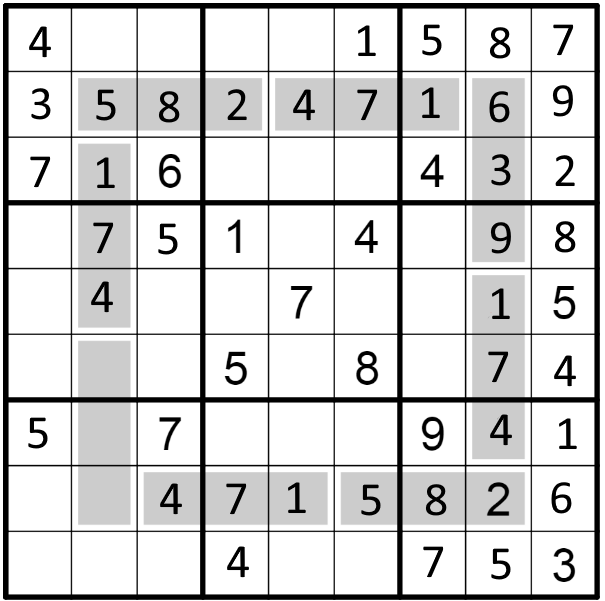
Step 5:
Following that, we can note that '5' can only go in R3C5 and '8' can only go in R3C4. Additionally, the cell in R1C2 must be a 2,5,8, but since 5,8 are already filled, it must be a 2. This and other deductions get us to:
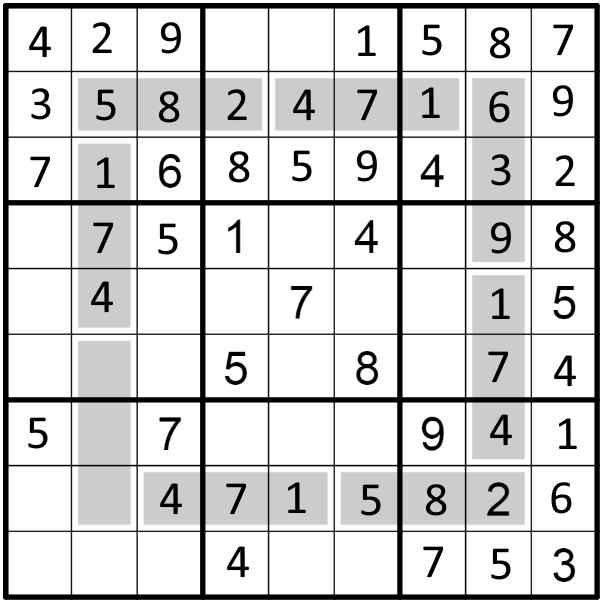
Step 6:
Next, note that in row 8, 3 must appear in R8C2 since there is already a '3' in Column 1. This allows us to place 9 in R8C1 and also solve for the last 1 x 3 group in column 2. This also allows us to place a '9' in R9C5 and some other knock-on deductions. So far, no contradictions!:
Step 7:
Using some more process of elimination logic, we can place a '8' in R5C1 and '2' in R7C6. Using the knock-on deductions from there:
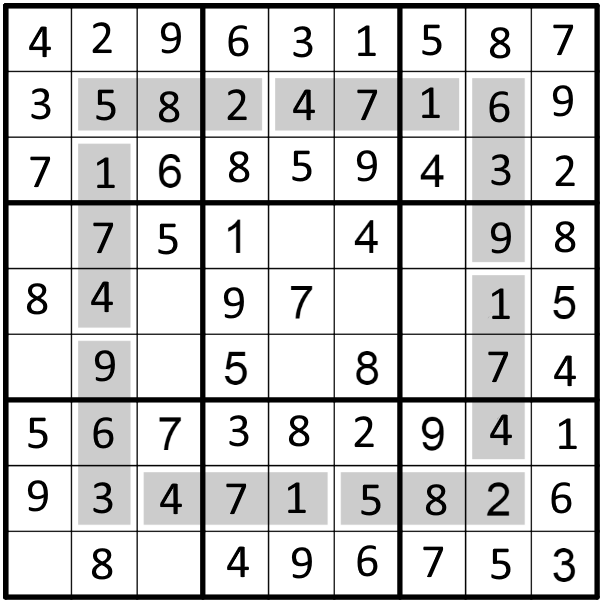
Step 8:
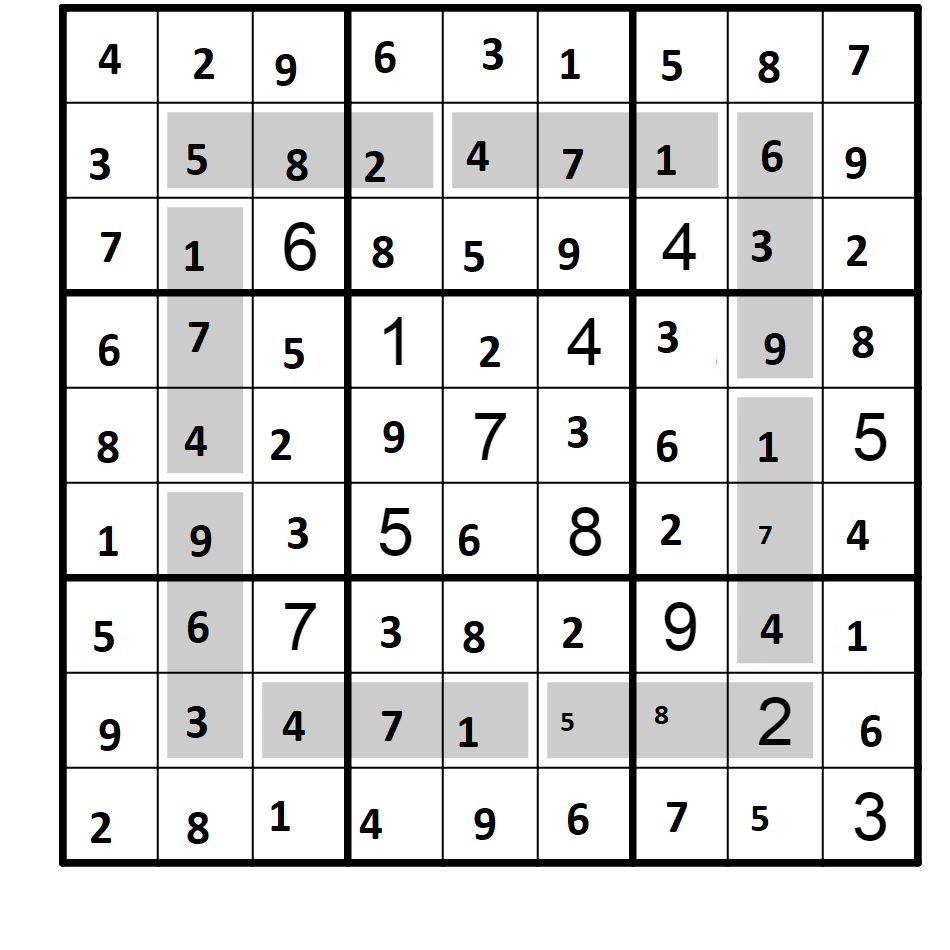
Next, we can notice that in column 5, only 2 and 6 are left. This means '3' must appear in R5C6. This deduction allows us to completely finish writing in all the '3's. Next, in row 5, there is also a 2 and 6 missing, but since 6 already appears in column 3, 2 must appear in R5C3. This allows us to place a 6 in R5C7. Based on the chain deductions that follow from there, we can fill in all the remaining digits and there were no contradictions. So this is the final solution:
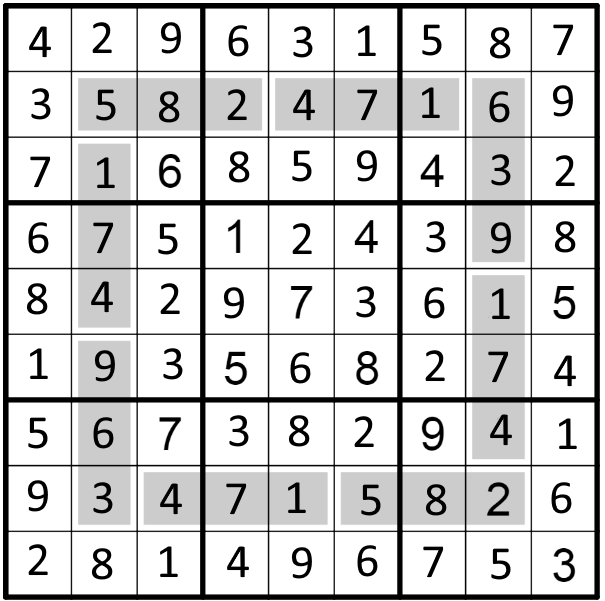
Note:
I decided that I show the other attempt as well to show that one leads to a contradiction and the above is the only solution to the problem.
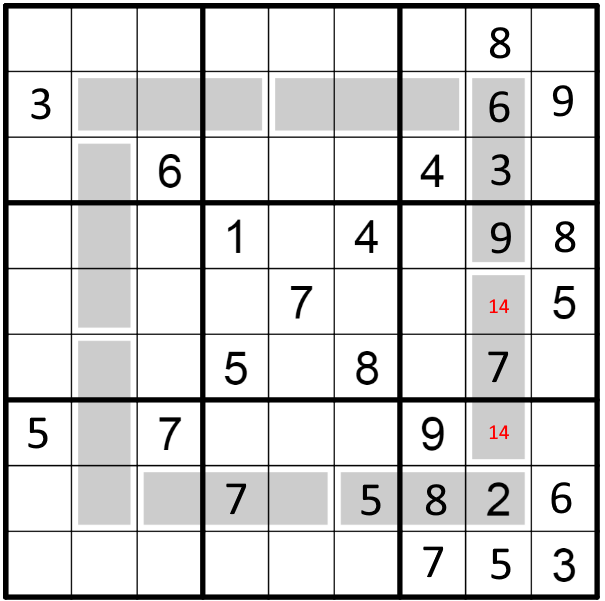
Placing '258' in the second 1 x 3 group in row 2 will eventually lead to the following contradiction:
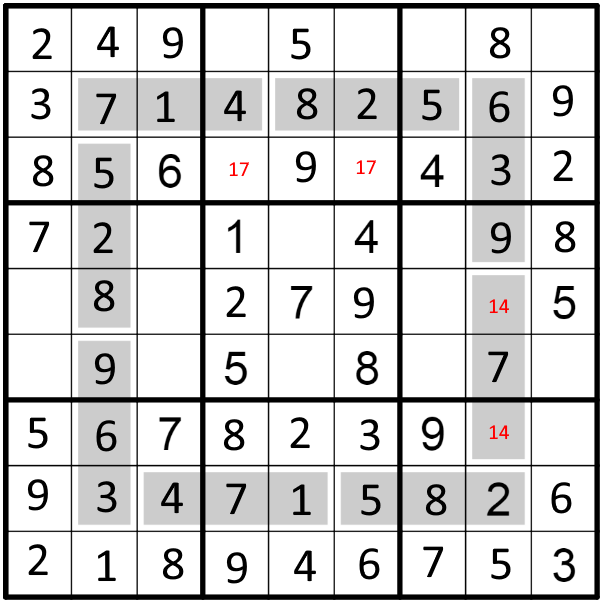
As can be seen, the only remaining numbers that can be placed in R3C4 are '1' and '7' but both of them already appear in the same column. Thus, the second 1 x 3 group in row 2 must be '147' as shown before.
As you can see, I also tried my hand and, finally, made it. I also got stuck and into a contradiction (as per the image).
Do you have more of these?
