Double n-queen puzzle, with sudoku rules

Question

Put 9 blue queens and 9 pink queens, with rules :

• If the blue queens are removed, the pink queens do not attack each other.
• If the pink queens are removed, the blue queens do not attack each other.
• Each 3×3 red square only has 1 blue queen and 1 pink queen.
• Some queens have been placed as a guide.
• There is only 1 solution.

Answer 1

Here is a solution, found by trying a lot, looks like a Sudoku:

Answer 2

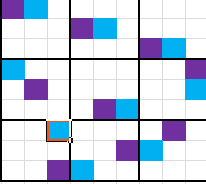
My (incorrect) solution:

Qq. ... ...
... Qq. ...
... ... Q.q

q.. ... ..Q
.Q. ..q ...
... .Q. .q.

..q ... .Q.
... ..Q q..
..Q q.. ...

Where Q indicates a purple queen and q indicates a blue queen.

The way I solved it:

There is only 1 possibility for the blue queen in the middle lower square. From there, the blue queen in the central square (<-- this is where I went wrong) and subsequently in the middle right square and the top left square can be determined, from which we get the remaining blue queens as well.
Now, only 1 possibility is left for the pink queen in the bottom left square. From there, the other pink queens are easily determined.

EDIT: Ah, I somehow made a mistake eliminating one of the fields in the central square. Will post an updated version with explanation, though I presume I will end up at the same solution as Skyvask.

New solution, which is (as expected) the same as Skyvask's:

Qq. ... ...
... Qq. ...
... ... Qq.

q.. ... ..Q
.Q. ... ..q
... .Qq ...

..q ... .Q.
... ..Q q..
..Q q.. ...

Where Q indicates a purple queen and q indicates a blue queen.

Found by:

The middle lower blue queen is still determined, which gives the lower left queen. That gives the lower left pink queen as well, which determines the pink queen in the lower middle square. Then, the middle top pink queen is determined, from which we can also deduce, in order, the lower right, lower left, and middle right and top right pink queens. We can also deduce the top left blue queen now. From there, we get the central blue queen, and can then easily fill in the middle right and top right blue queens.

Since all of the above is logically deduced, this also proves that is a unique solution.