2
$\begingroup$

Similar to: Unlucky tiling: Arrange thirteen right isosceles triangles into a square

Five graded difficulty isosceles right triangle into square tilings

V.hard problem, 20 right isosceles triangles into a square

Each tiling has only one solution, these might be possible by hand, computers allowed.

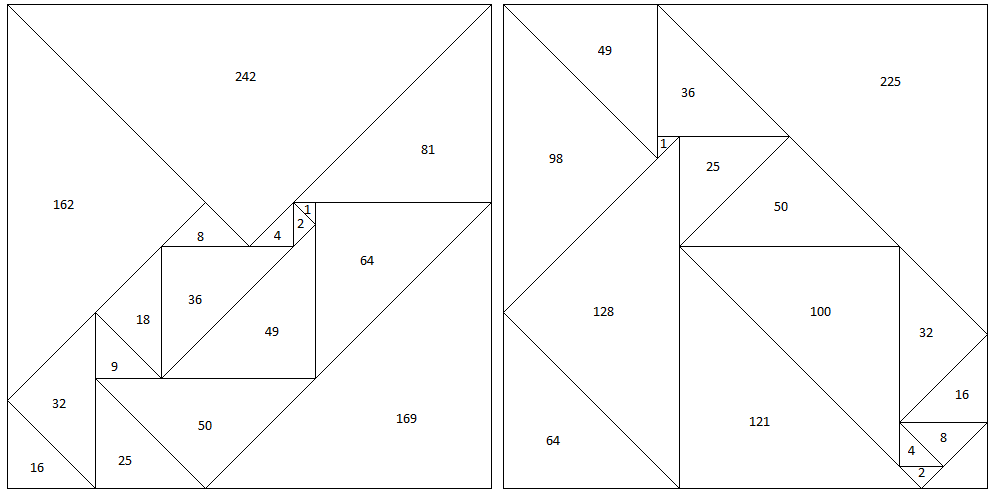
The two challenges are to arrange $17$ right isosceles triangles of the listed areas into a square of area $968$ with no gaps or overlaps. The square has a diagonal of length $44$.

$1, 2, 4, 8, 9, 16, 18, 25, 32, 36, 49, 50, 64, 81, 162, 169, 242$ $1, 2, 4, 8, 9, 16, 25, 32, 36, 49, 50, 64, 98, 100, 121, 128, 225$

The answer tick will be given to whomever posts the solution to the second puzzle first, or the first if nobody gets the second. Only because the second looks slightly harder to me.

By way of illustration/clarification, here are the right isosceles triangles of area

$1, 2, 4, 9, 16, 18, 50$

arranged into a $10\times 10$ square:

10x10_7

Improve this question
$\endgroup$
2
  • $\begingroup$ #pouts# the last one wasn't even solved yet! I'm still working on it lol $\endgroup$
    – NL628
    Commented Apr 23, 2018 at 4:01
  • $\begingroup$ No rush. I'll give you another half hour. $\endgroup$
    – theonetruepath
    Commented Apr 23, 2018 at 9:27

2 Answers 2

Reset to default
4
$\begingroup$

Here are the solutions to both questions:

enter image description here

Improve this answer
$\endgroup$
1
  • $\begingroup$ Yup that's em. I'll put up mine in my style too. $\endgroup$
    – theonetruepath
    Commented May 22, 2018 at 5:15
0
$\begingroup$

In my pic style for completeness:

44_17_17

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.