Reassembling the Marquetry

Question

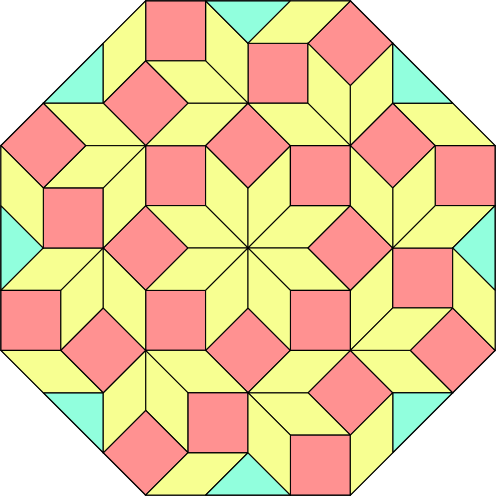
You decided to visit a woodworker's home. This woodworker is very famous for their skills in marquetry - that is, setting pieces of wood to create patterns - and had a piece of a very geometrical nature assembled on top of their work bench, not yet glued together.

Unfortunately, you are a rambunctious young whippersnapper with no patience for these old things, so you decide to play an impromptu game of "the floor is lava" while in the workshop. In the course of this game, you accidentally knock the whole workbench over, spilling the contents all over the floor and causing the assembled design to scatter in many pieces.

You notice that the pieces are of three varieties as pictured and described below:

• There are $24$ square pieces. Let us say they have side length $1$.

• There are $40$ rhombuses. Each rhombus has two angles measuring $45^{\circ}$ and two measuring $135^{\circ}$. All their side lengths are $1$ as well.

• There are $8$ isosceles right triangles. They have two sides of length $1$ and one side of length $\sqrt{2}$.

You decide that, in order to avoid trouble, you should reassemble the pattern based on the brief glimpse you got of the assembled piece before you carelessly careened into it. You recall three things: Firstly, the pieces fit together to form a regular octagon, leaving no empty space inside the octagon nor any overlap of multiple tiles. Secondly, you recall that squares did not border* other squares, nor did triangles border triangles, or squares border triangles. Finally, you recall that if two rhombuses bordered each other, then they were in rotated differently - that is, two bordering rhombi were never mere translations of each other.

You figure that as long as you reassemble a pattern satisfying all that, the woodworker will probably not notice any difference. How can these tiles be put together to form a suitable octagon?

(*"Border" here means "share an edge with in the completed tiling")

Answer 1

(All credit for the neatness of the diagram goes to 2012rcampion -- the diagram I posted was a mess and he, entirely unprompted, replaced it with the rather nice one now seen above. Thanks, 2012rcampion!)

This was largely trial and error, beginning with the observations that

the side length has to be $2+\sqrt{2}$ and therefore we need one triangle-hypotenuse on each side

and (to save effort)

looking only for highly symmetrical solutions.

The first thing I tried was to

put a triangle in the middle of each edge and a rhombus on each side, but that is readily seen not to work. Next was the pattern above, starting at the edges and maintaining rotational symmetry; doing what felt most natural at each stage produced an octagonal ring with a smaller internal octagon to be completed; then there was only one halfway plausible way to place the squares, after which everything else was forced.

Answer 2

Gareth already found my first solution, so here's another:

... and another, this time with some more mirror symmetry.

Answer 3

Since what I have so far is in a comment I'll post my derivation of it

Firstly, the pieces fit together to form a regular octagon, leaving no empty space inside the octagon nor any overlap of multiple tiles

The area of the octagon must be the sum of the area of the tiles
Square tiles have area $S=1\times1=1$
Rhombus tiles have area $R=1\times1\times\sin(\frac\pi4)=\sqrt{\frac12}$
Triangle tiles have area $T=\frac12\times1\times1=\frac12$

So the octagon has area $O=24S+40R+8T=24+\sqrt{800}+4=28+\sqrt{800}$
hence the octagon has sides of length:\begin{align}o&=\sqrt{\frac48(28+\sqrt{800})\tan(\frac\pi8)}\\&=\sqrt{(14+\sqrt{200})\tan(\frac\pi8)}\\&=\sqrt{(14+\sqrt{200})(\sqrt2-1)}\\&=\sqrt{14\sqrt2+\sqrt{200}\sqrt2-14-\sqrt{200}}\\&=\sqrt{14\sqrt2+20-14-10\sqrt2}\\&=\sqrt{4\sqrt2+6}\\&=\sqrt{(2+\sqrt2)(2+\sqrt2)}\\&=2+\sqrt2\end{align}
So each of the $8$ sides of the octagon must abut with a triangle's hypotenuse, of which we have only $8$ so we may place them first, subject to being slid along the sides of the octagon.

...nor did triangles border triangles, or squares border triangles

So $16$ of our rhombuses must be used straight away adjoiniing the other two sides of each triangle