10
$\begingroup$

An Eisenstein triple is related to 60 degree triangles and a special case of the cosine law. But we need not worry about that except to note that a specific example of an Eisenstein triple is $7^2 = 5^2 - 5\times8 + 8^2$ which we can rewrite as $7^2 + 5\times8 = 5^2 + 8^2$

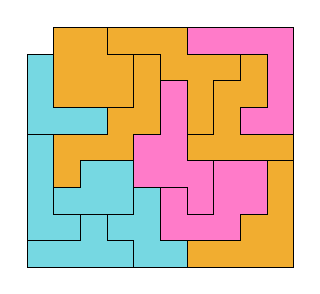
Which means we can have a puzzle involving eight octominoes and five pentominoes, colored as per the given 9x10 rectangle with missing corner.

Your tasks:

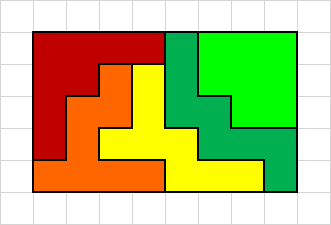
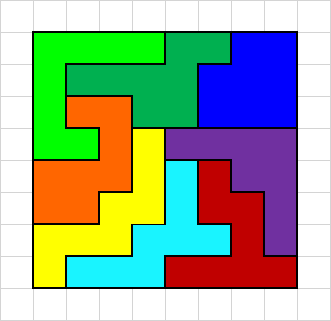
  1. Arrange the five blue pentominoes into a 5x5 square

  2. Arrange the five orange octominoes into a 5x8 rectangle

  3. Arrange the five blue pentominoes plus the three pink octominoes into a 7x7 square

  4. Arrange the five orange plus three pink octominoes into an 8x8 square.

All these have a single solution (ignoring rotations and reflections). You are allowed to flip pieces over. And to keep things simple the color on the other side is the same. Using a computer will just spoil it for you, these are at a 'hand tiling' difficulty level.

Bonus question:

  1. Arrange all 13 pieces into a 9x10 with missing corner, like the diagram here, but in such a way that each color forms a single connected area. Touching at a corner is not touching in this instance. There are seven ways to do this (ignoring R&R as usual). If you feel that you haven't done enough, find all seven.

enter image description here

Improve this question
$\endgroup$

2 Answers 2

Reset to default
12
$\begingroup$

Partial answer, Tasks 2-4

Finished Task 2

enter image description here

Finished Task 3 (what a doozy)

enter image description here

Finished Task 4 (easier than 3)

enter image description here

Improve this answer
$\endgroup$
1
  • $\begingroup$ Nice going. These aren't easy. you're right. I hadn't realised that task 3 would be harder than 4. Answer awarded. $\endgroup$
    – theonetruepath
    Commented Oct 17, 2019 at 23:10
9
$\begingroup$

Partial Answer

Finished Task 1

enter image description here

Improve this answer
$\endgroup$
5
  • $\begingroup$ How do y'all do the magic square color grid thingy? It's really useful for these polyomino tilings. $\endgroup$
    – Avi
    Commented Oct 17, 2019 at 2:22
  • 2
    $\begingroup$ @Avi i use microsoft excel :) $\endgroup$
    – Omega Krypton
    Commented Oct 17, 2019 at 2:23
  • $\begingroup$ Oh, fancy :) I'll try it out $\endgroup$
    – Avi
    Commented Oct 17, 2019 at 2:24
  • 5
    $\begingroup$ @PilsNot3 Hmm.. I'm going to argue that while this is a small answer, this does make a significant progress. There are only 4+1 tasks so answering 1 should be ok. Moreover, it can be confirmed as a progress: it's not a "guess" like in cryptic clue, but this answer's correctness can objectively determined (i.e. did 5x5 square). $\endgroup$
    – athin
    Commented Oct 17, 2019 at 4:58
  • 1
    $\begingroup$ @athin Yeah, I was probably being too critical with the downvotes; my thinking was that since the questions are related to each other it wouldn't be enough to just answer one and leave the others alone, but perhaps that doesn't make partial solutions like these invalid. And considering how quickly (~30 mins after initial posting) the partial solutions came in, I wasn't sure if they genuinely represented the farthest progress made or were just posted to gain rep quickly, but maybe I judged wrong. $\endgroup$
    – HTM
    Commented Oct 17, 2019 at 5:59

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.