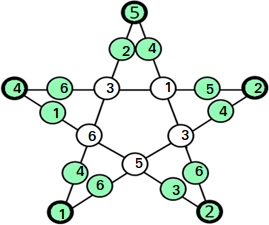
Fill in all the blank circle with numbers 1 to 6, so :
- Each lines of 6 circles, contains 6 different numbers.
- Each corner (consist of 3 greens circle) sums to 11.
- There is only 1 unique solution.
$\begingroup$
$\endgroup$
1
asked Nov 2, 2017 at 3:41
-
$\begingroup$ this reminds me of one of my question, though cant think of solving this without a computer :/ $\endgroup$– OrayCommented Nov 2, 2017 at 8:43
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
Solution:
Reasoning:
The only possible combinations for the green corners are:
1-4-6
1-5-5 - only if 1 is in the start corner
2-4-5
2-3-6
3-4-4 - only if 3 is in the star corner
3-3-5 - only if 5 is in the start corner
And we start from the top corner:
The only valid combinations are 2-4-5 and 3-3-5
I decided to try with 2-4-5 because this will force me to put the 2 on the left side because of the 2 on the lower right corner
Next step
Try to fit in the lower right corner because we already have a digit there
The possible combinations are 2-4-5 and 2-3-6.
The only valid one is 2-3-6 because of the 4 and 5 in the top green 3 cell corner. Putting it like this:
will make us move to the lower left corner with the valid combinations: 1-4-6, 2-3-6, 3-4-4.
Trying them 1 by 1 resulted in contradictions.
So we back trace and change the order of 6 and 3 in the lower right corner
Next step, lower left corner:
possible combinations: 1-4-6, 2-3-6, 3-4-4
Trying with 3-4-4 and 2-3-6 results again in conflicts.
Note: I'm not adding all my tries here because the answer will become boring and unreadable. And I don't want to upload 60 pictures.
Trying with 1-4-6. (2 possible combinations). Just pick one. If it fails...try the other
In this combination we are left with the numbers 3 and 6 for the line from top to lower left. Obviously 3 goes on the upper empty cell because otherwise it will conflict with the line from upper left to lower right
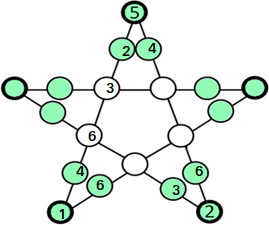
Next: We can fill now the line that goes from top to lower right.
only available digits are 1 and 3 and 1 obviously goes above 3.
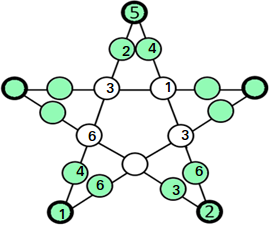
Moving on to the upper left corner.
We cannot use any combination that contains a 3 because there are already 3s on both lines that start from that corner. We cannot use 1-5-5 either because there is already a 1 on the horizontal line from that corner. So we are left with 1-4-6.
Obviously 6 has to be on the horizontal line.
And 1 cannot go directly in the corner because of the horizontal line This make the lowest white cell be 5
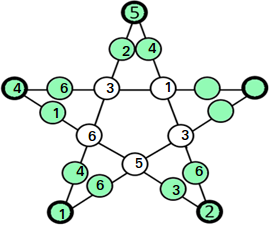
Last step:
We are left with 3 numbers to put in the upper right corner.
2, 4, 5.
There is only one combination that fits.
$\begingroup$
$\endgroup$
2
It tooke me 2 hours to solve it and this is my answer:
-
1$\begingroup$ Since this is grid-deduction, can you add your solve process, or was this pure trial and error? $\endgroup$ Commented Nov 2, 2017 at 9:35
-
1