To solve this, we first express the problem in a new way: Instead of having a laser bouncing between two mirrors, we have a laser moving straight through a different space; to accomplish this, we draw what you would see if you were standing in the setup - that is, where the mirrors are, we place a new, reflected image of the whole space! That is, we make copies of the $1^{\circ}$ wedge by mirroring it over the rays that bound it. The result looks something like this:
 where the line segments represent the mirrors and the red line is the laser. This is basically what you'd see if you were standing inside such a setup (although you'd see many copies of yourself too). We may regard this as a sort of "covering space" for the old one as each point in the old one is copied many times into the new one.
where the line segments represent the mirrors and the red line is the laser. This is basically what you'd see if you were standing inside such a setup (although you'd see many copies of yourself too). We may regard this as a sort of "covering space" for the old one as each point in the old one is copied many times into the new one.
This makes the problem simple: How many consecutive line segments can the red line cross? Well, let's call the distance from the center of the circle to the laser $d$. And, for simplicitly, lets call the radius of the inner circle $1$ and the outer circle $2$, since we only need to preserve the ratio between those measurements (which is 10m : 20m originally). Then, a laser crosses a line segment measuring an angle of $\theta$ to it if:
$$\sin(\theta)\leq d\leq 2\sin(\theta)$$
We thus require that the smallest value of $2\sin(\theta)$ of any line we cross is at least equal to than the greatest value of $\sin(\theta)$ in the lines that we cross - otherwise, no suitable $d$ exists.
Using this inequality, suppose we have that the leftmost line $\alpha$ to the line, and the rightmost line is at an angle of $\beta$ (which will be greater than $\alpha$). We may assume that $|\alpha|\geq|\beta|$, as the two are related by reflection. Moreover, we may then write $\alpha=(n+c)^{\circ}$ where $n$ is an integer and $-\frac{1}2\leq c\leq \frac{1}2$ - meaning $90^{\circ}+c$ will be the maximum $\sin(\theta)$ to appear. Then, all we need to satisfy for the intervals for $d$ to intersect is:
$$\sin(90+c)\leq 2\sin(\alpha)$$
$$\sin(90+c)\leq 2\sin(\beta)$$
Now, I'm sure there's an elegant way to solve this. I'm kind of a hack though, so we'll just make some guesses: Suppose that $c$ were $\frac{1}2$. Then we can find that the integer $n$ satisfying
$$\sin(90+\frac{1}2)\leq 2\sin(90+n+\frac{1}2)$$
are exactly those in the interval $[-60,59]$, meaning we can, from any point, cross $60$ to either side of it (as $c=\frac{1}2$ is the most extreme value possible) - for a maximum of $121$ reflections.
However, letting $c$ be zero, it's a fairly common fact from highschool trigonometry that $\sin(30^{\circ})=\sin(150^{\circ})=\frac{1}2$ that $\alpha=30^{\circ}$ meaning that we can hit every mirror making an angle between $30^{\circ}$ and $150^{\circ}$ with the laser - this achieves the maximum of $121$ reflections. To do this, we do as follows:
Aim the laser at the outer edge of one of the mirrors, impacting the mirror at a $30^{\circ}$ angle. It will reflect $121$ times.
A picture of this would probably just look like a lot of red lines. However, the same logic applies if the angle between the mirrors were $5^{\circ}$ and predicts $25$ reflections. That looks like this:
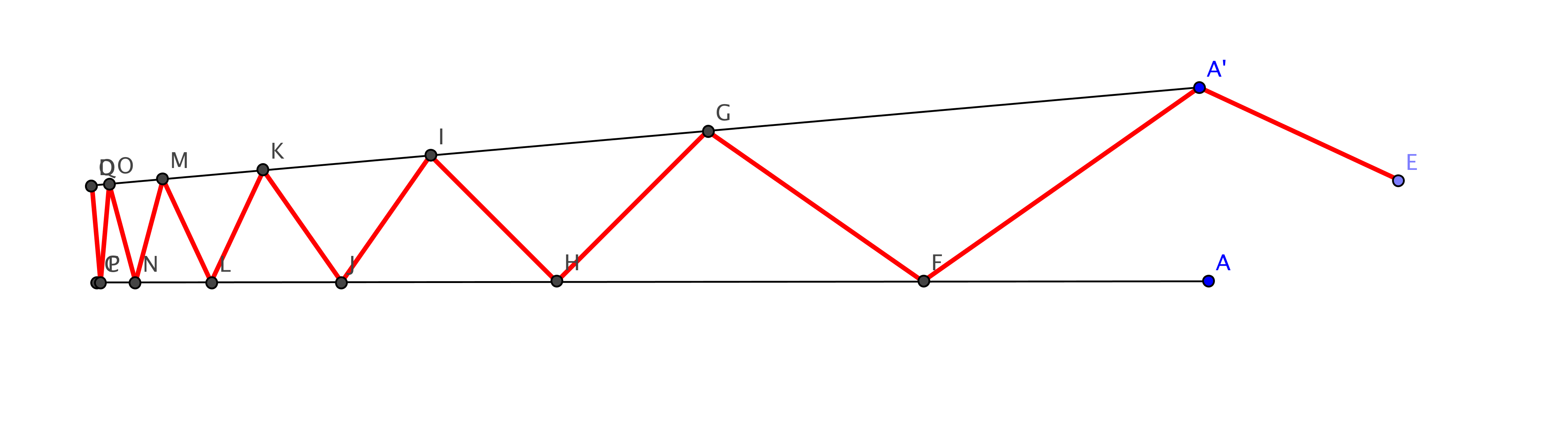 Notice that it hits one mirror perpendicularly, and hence retraces its steps. In other words, some advice:
Notice that it hits one mirror perpendicularly, and hence retraces its steps. In other words, some advice:
Don't look in the direction you point the laser.


 where the line segments represent the mirrors and the red line is the laser. This is basically what you'd see if you were standing inside such a setup (although you'd see many copies of yourself too). We may regard this as a sort of "covering space" for the old one as each point in the old one is copied many times into the new one.
where the line segments represent the mirrors and the red line is the laser. This is basically what you'd see if you were standing inside such a setup (although you'd see many copies of yourself too). We may regard this as a sort of "covering space" for the old one as each point in the old one is copied many times into the new one. Notice that it hits one mirror perpendicularly, and hence retraces its steps. In other words, some advice:
Notice that it hits one mirror perpendicularly, and hence retraces its steps. In other words, some advice: