2
$\begingroup$

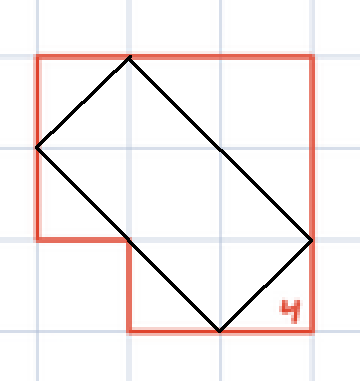
It's been a long time since I've posted an origami puzzle!

An origami puzzle is solved with some thickness $x$ iff it can be folded into a rectangle (possibly a square) with thickness $x$ everywhere.

Identify a puzzle in the official ORIGAMI PUZZLES that can be solved with some thickness $t'$ that is a non-trivial factor of its labeled thickness $t$ (that is, $t'|t$ and $t'\ne t$) and solve it as such.

Although solutions are not required to be applicable in real life, using an actual sheet of paper is a great visualization tool.

A puzzle for this type of orgami puzzle game made by Ωmega_3301, similar to this question.

Improve this question
$\endgroup$
2
  • $\begingroup$ I can fold #14 to thickness 5, #25 to 5, #26 to 7, #27 to 11 and #29 to 12. Does it count as trivial? $\endgroup$
    – Florian F
    Commented May 21 at 6:43
  • $\begingroup$ How does that answer my question? $\endgroup$
    – Sny
    Commented May 21 at 10:35

1 Answer 1

Reset to default
3
$\begingroup$

$t = 4$, $t' = 2$:
enter image description here

Found this when originally solving the first few example puzzles.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Intended, well done $\endgroup$
    – Sny
    Commented May 20 at 23:00

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.