An infinite series of origami puzzles

Question

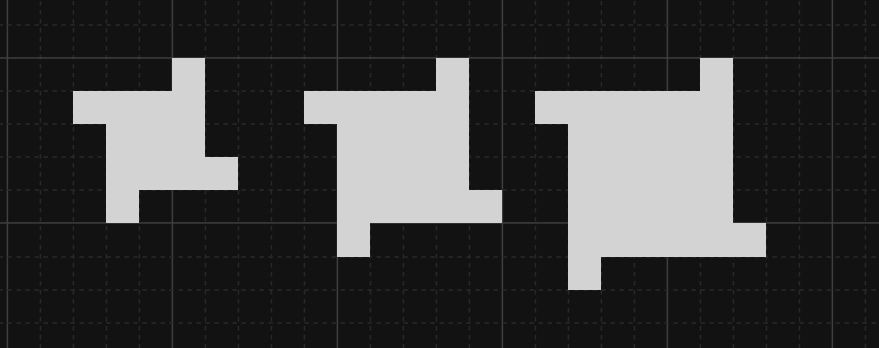
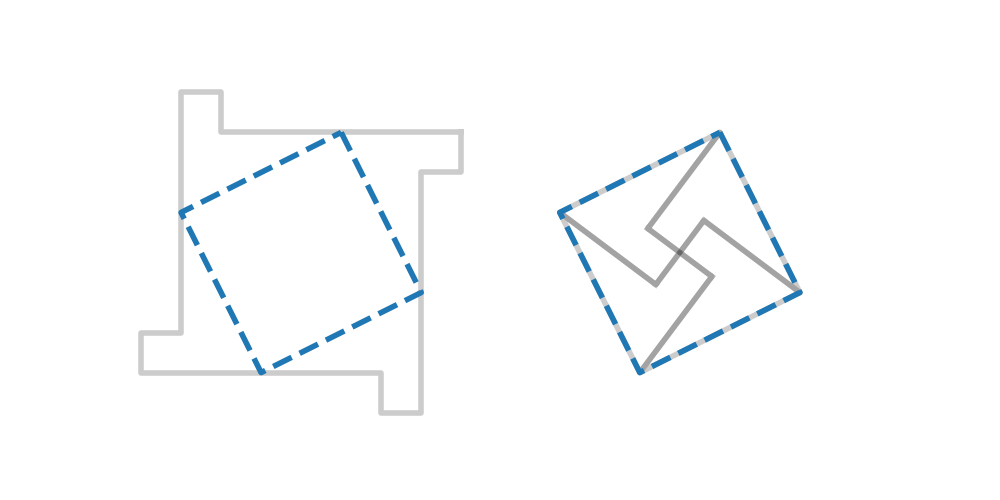
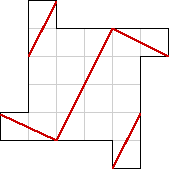
fold the 3 shapes below into rectangles with two layers of paper everywhere. the white part of the image is the puzzle.

I'm sure you noticed the pattern in the shapes. now extend it and solve all shapes of that pattern. this will need a general solution.

in case it's not clear, the pattern is a large square (side >2) in the center with 4 small squares at the side.

Similar to ORIGAMI PUZZLES completed version which is also made by me.

Albert.Lang · Accepted Answer · 2024-05-16 07:21:24Z

9

See picture:

The corners of the blue square (which indicates where to fold) are the midpoints of the four long sides of the given dodecalateral (is that a word?).

answered May 16 at 7:21

Albert.Lang

9,41017 silver badges58 bronze badges

$\begingroup$ good job! that's my intended solution too. i'll just assume you found out how to generalise it since it's rather obvious :) $\endgroup$
– Omega_3301
Commented May 16 at 7:51
3

$\begingroup$ dodecagon! technically, triangles are called "trigons", and quadrilaterals are called "tetragons", but Anglophones hate regularity (somewhat facetiously), so… $\endgroup$
– Someone
Commented May 16 at 12:45

Add a comment |

Florian F · Accepted Answer · 2024-05-16 19:24:58Z

4

And if you don't like the solution given, here is another one!

Equally easy to generalize.

answered May 16 at 19:24

Florian F

31.4k4 gold badges70 silver badges145 bronze badges

$\begingroup$ cool! i didn't consider this one, but i guess it makes sense if you did another puzzle in my original puzzle book... i won't say which one it is because that would be pretty spoilerly $\endgroup$
– Omega_3301
Commented May 17 at 14:42
$\begingroup$ @Omega_3301 oh I know exactly which one you are referring to 😉 $\endgroup$
– PDT
Commented May 18 at 6:16
$\begingroup$ yes ofc you do haha i saw your comment :) $\endgroup$
– Omega_3301
Commented May 18 at 11:25

Add a comment |

2 Answers 2