6
$\begingroup$

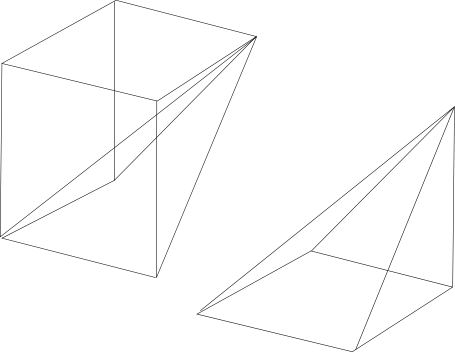
I had asked this question on Facebook.

Imagine a cube. Put it on a flat surface, so that four vertices are at the bottom and four vertices are on top. Select any vertex on top, and connect it to each of the four vertices at the bottom. This now encloses a part of the cube. Cut this enclosed part off, and you are left with another shape.

How many faces does this leftover shape have?

I feel the answer is seven, but my friends say five. This may be a relatively easy question for this site, but I found it quite hard to visualize.

Improve this question
$\endgroup$
3
  • $\begingroup$ by "points on top" you mean top corners, right? And how do we "Connect this point to each of the 4 points...?" $\endgroup$
    – JLee
    Commented Apr 22, 2015 at 15:27
  • $\begingroup$ @JLee Yes, 4 top corners and 4 bottom ones. Connect using imaginary line segments. (whether on the cube or passing through it). $\endgroup$
    – ghosts_in_the_code
    Commented Apr 22, 2015 at 15:29
  • 1
    $\begingroup$ This related question has the answer to yours. puzzling.stackexchange.com/questions/8429/… $\endgroup$
    – Ethan Bolker
    Commented Apr 22, 2015 at 18:24

4 Answers 4

Reset to default
18
$\begingroup$

You have two pieces one has 5 faces the other 7.

You and your friend are talking about different pieces.

Two parts of a cube

The piece on the left has a total of 7 faces (5 that were on the outside of the cube and 2 that are formed inside by cutting.)

The piece on the right has 5 faces (3 that were on the outside of the cube and 2 that are formed inside by cutting.)

The shape enclosed by the points is the one on the right (5 faces) the piece left over is on the left (7 faces.)

Improve this answer
$\endgroup$
3
  • $\begingroup$ Thanks, the picture makes it so clear! So was my question not clear enough that people assumed the wrong shape? $\endgroup$
    – ghosts_in_the_code
    Commented Apr 22, 2015 at 15:36
  • $\begingroup$ Yes I think that is the source of confusion between you and your friend. You both have the right answer to slightly different questions. $\endgroup$
    – Bob
    Commented Apr 22, 2015 at 15:41
  • 2
    $\begingroup$ The correct answer to the question as worded is 7. The shape that is enclosed by the 5 points named in the question has 5 sides. You have cut this enclosed shape out and are asked to consider "another shape" that is left over after this cut. If you intended the answer to be 5, consider rewording the last two sentences as "You have now cut out a new shape. How many faces does this new shape have?" $\endgroup$
    – Ian MacDonald
    Commented Apr 22, 2015 at 16:03
1
$\begingroup$

The shape that was cut out would be a square pyramid. Therefore it would have 5 faces: the 4 triangular faces formed when cutting it out of the cube as well as the square bottom face of the cube.

The leftover shape would have 7 faces: the top face of the cube, the other triangular halves of the 2 cube faces that were cut to form faces of the pyramid, the 2 internal faces adjacent to the other faces of the pyramid, and the 2 side faces of the cube that were untouched in the formation of the pyramid.

If you chose a point internal to the top face of the cube instead of one of the top vertices, you'd still get a square pyramid as the shape you cut out. However, then the leftover shape would have 9 faces. In that case the 4 sides of the cube would all stay intact as faces of the leftover shape, and you would have 4 faces corresponding to the 4 triangular faces of the pyramid.

Improve this answer
$\endgroup$
2
  • $\begingroup$ We are cutting out the square pyramid from the cube, and seeing the leftover shape. $\endgroup$
    – ghosts_in_the_code
    Commented Apr 22, 2015 at 15:34
  • $\begingroup$ Ah, I missed that in my first read. Edited with information about the leftover shape as well. $\endgroup$
    – Togashi
    Commented Apr 22, 2015 at 15:39
1
$\begingroup$

I guess it depends what you mean by "connect this point to each of the 4 points at the bottom" if its by a straight line (regardless of matter) then your answer is:

5. If your base points are numbered 1 to 4, and your origin point >!is labeled A. Then you will have the bottom face (straight vertical line >!from A to the point immediatly below (1). You will also have two side faces going up vertically from the base as triangles as defined by lines from A to (2) and (3) which are the points next to (1). Finally the final two faces will be a diamond slanted from point A to (4 - across from 1), each half of the diamond resting on the 2 vertical triangles and the line from A to 4.

Picture

A picture worth the whole explanation

Improve this answer
$\endgroup$
3
  • $\begingroup$ How is 4 possible? Could you add a diagram? $\endgroup$
    – ghosts_in_the_code
    Commented Apr 22, 2015 at 15:30
  • $\begingroup$ haha ill correct its, 5, the diamond is 2 faces. Jiminion has it right $\endgroup$
    – Spacemonkey
    Commented Apr 22, 2015 at 15:33
  • $\begingroup$ aaaand now with edit to the question answer is 7 but as there is already more complete answers , not much point in editing further $\endgroup$
    – Spacemonkey
    Commented Apr 22, 2015 at 15:43
0
$\begingroup$

The answer is:

5. There are the 3 faces on the bottom and each vertical side next to the upper point. The new revealed surface is two faces with a ridge extending from the upper point to the lower diagonal point.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.