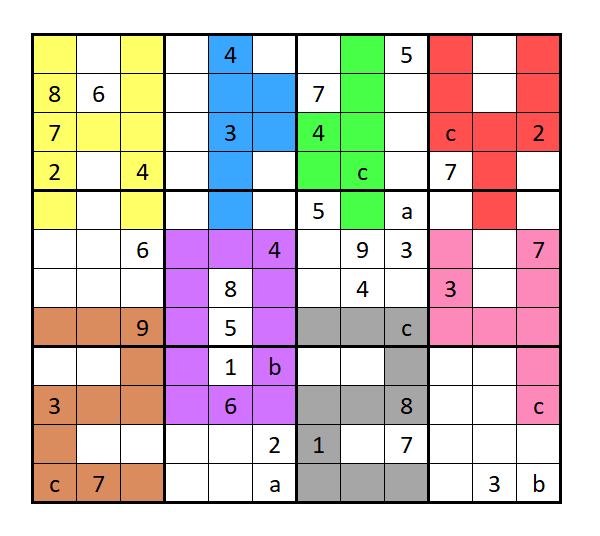
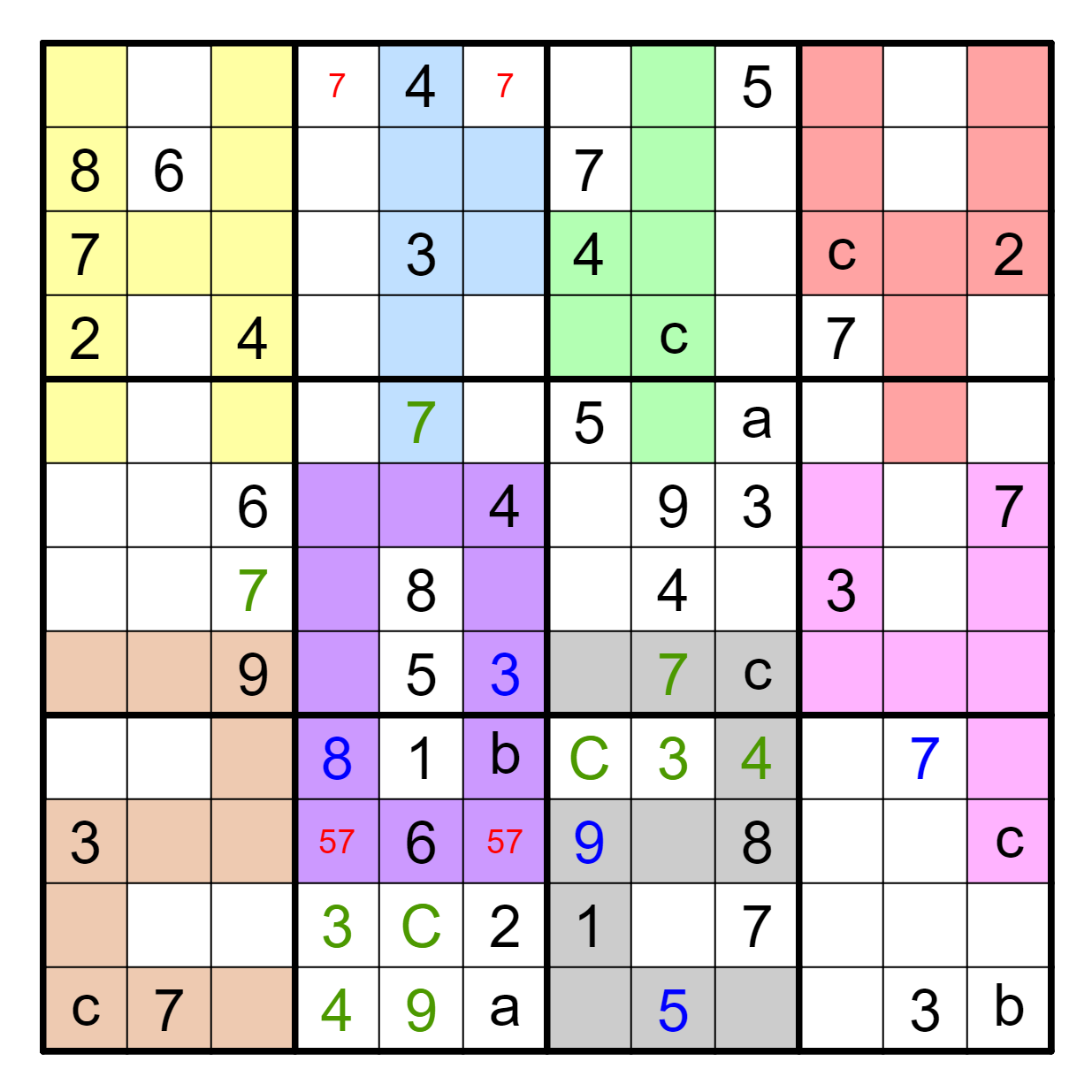
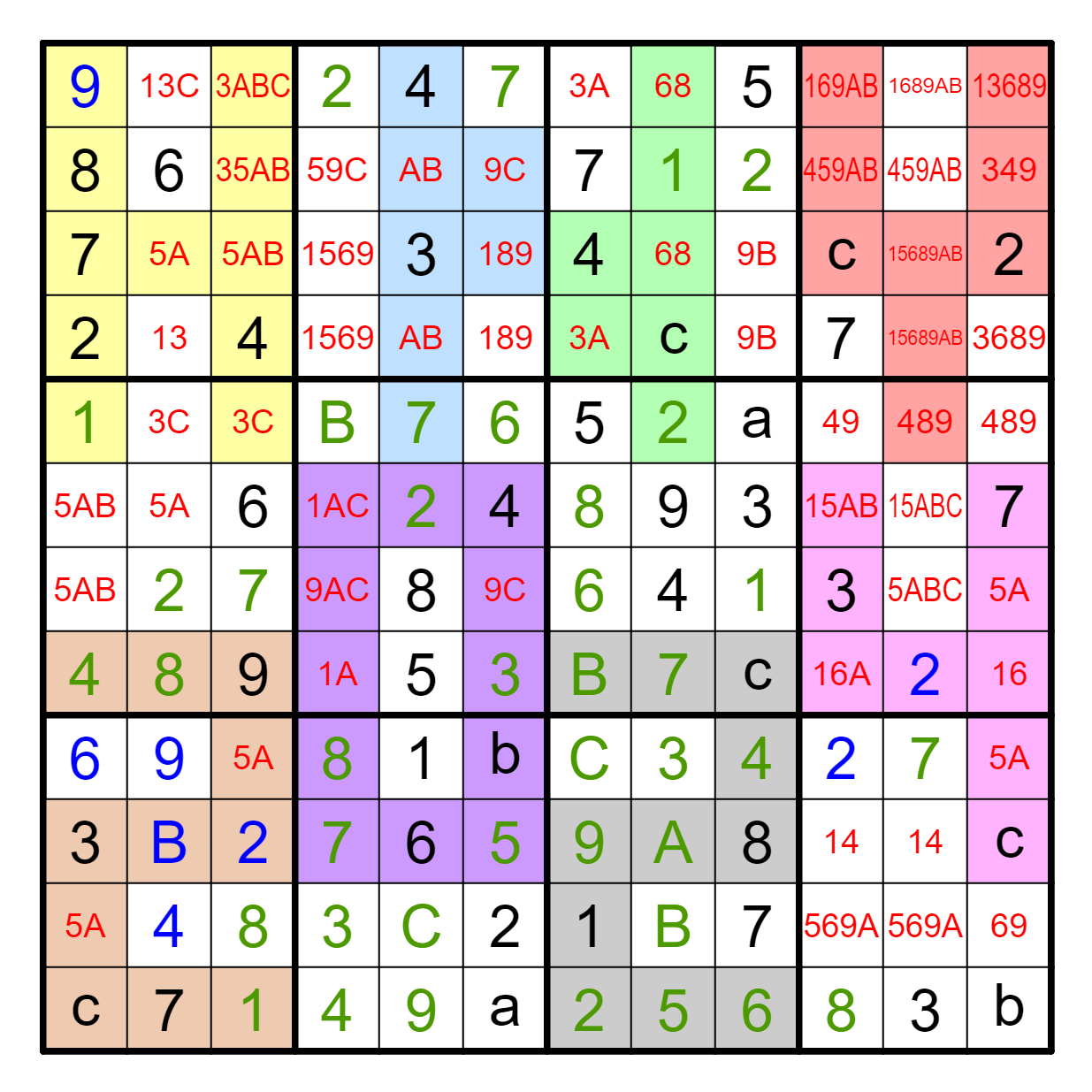
The following puzzle is a typical Sudoku 12 x 12 (1-9, a, b, c), but with an added twist. In addition to the standard rules, each colored box must also contain unique digits; no repetition is allowed within each colored section. Here's to a prosperous and joyful 2024! Happy New Year!
Happy New Year!!
This was very tricky! Took just over 4 hours in total, but what better way to start the year :P
Step by step:
1:
Starting off, ignoring the colours and solving like a normal sudoku, a few cells can be filled in. Column 5 has a few cells with only one possibility, and a couple of 7s can be placed as well as a C.
2:
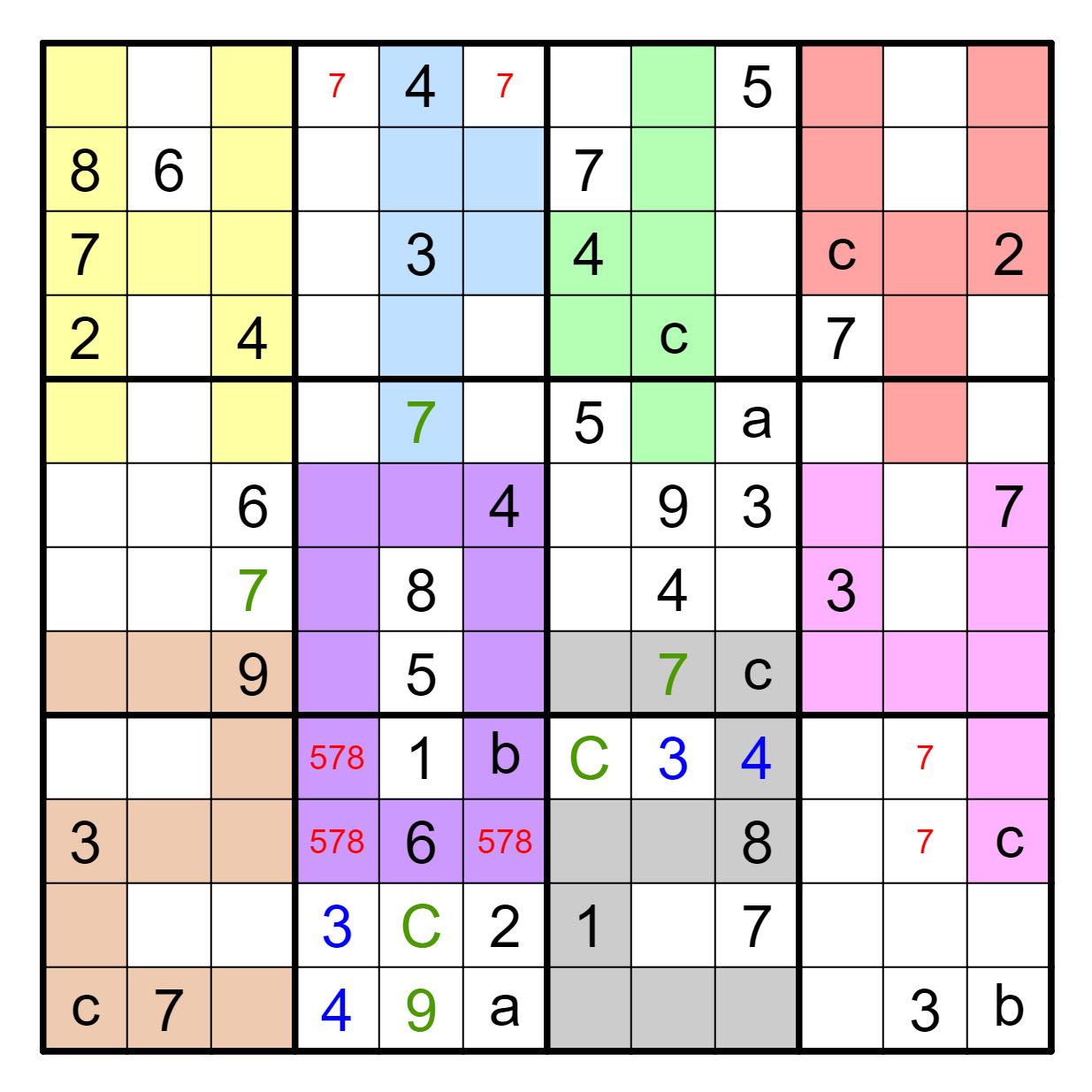
Now the colours come in to play. There seems to be the most information around the middle bottom so lets start there. The '0' will be of particular use as it contains 12 colours cells, meaning every digit must be included. The 0 does not currently include a 5, 7 or 8, but all these are in box 6. So the 5, 7 and 8 must be in the purple cells of box 10, leaving a 4 and a 3 that can be placed in that box. A 3 and 4 can then be placed in box 11.
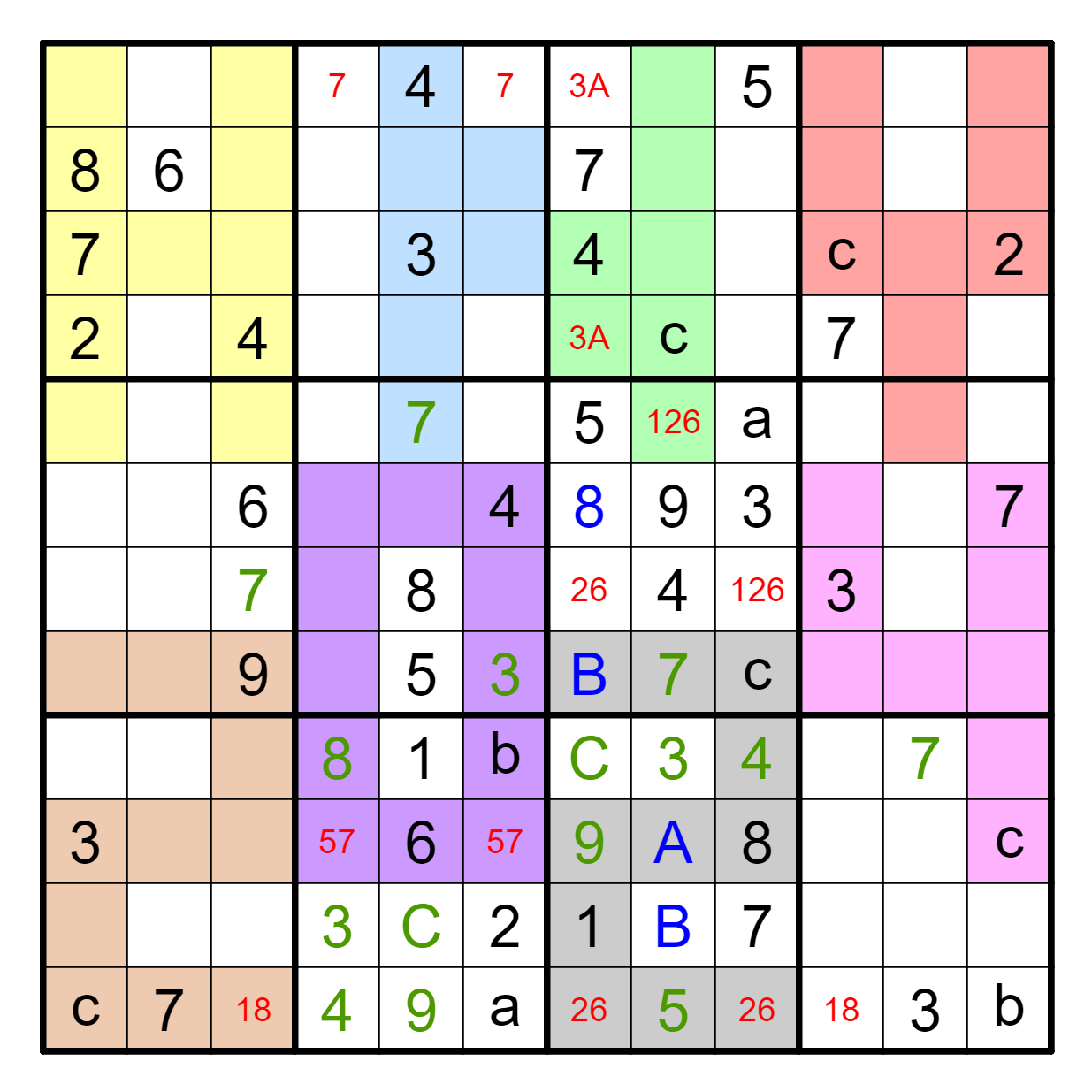
3:
The 8 in the 578 triple only has one place it can go, and this leaves a 7 in that row ruling out a candidate on the right, placing a 7 in box 12. A 9 can be placed in box 11, as well as 5 due to a 2/6 pair in the same box.
Going back to the '0', it currently doesn't include a 3, and there is only place a 3 can go.
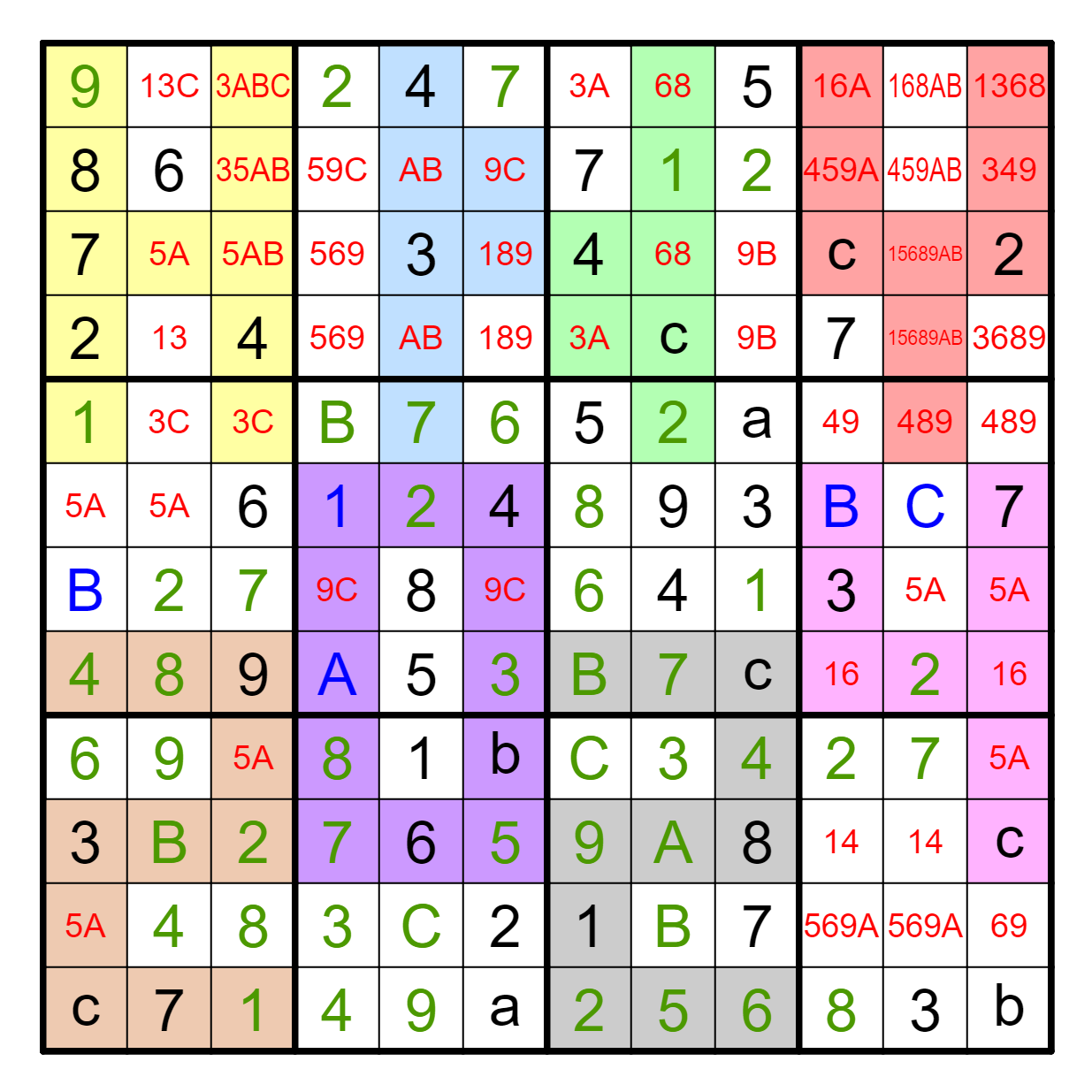
4:
Now looking at the second '2' in '2024', it contains 11 cells, and so 11 digits. But which of the 12 available won't be included? The digit not included has to be the 3, as it is in boxes 7 and 11, meaning it can't be placed in any of the coloured cells anyway. So we know the '2' must include every digit except 3. (The same logic can be applied to the 'H' which won't contain a 6). So that means the second 2 is currently missing 2, 6, A, and B.
The 2, 6 form a pair on the bottom row, so the A and then the B can be placed. This leaves a B in box 11, and there is a 2/6 pair in column 7 placing an 8.
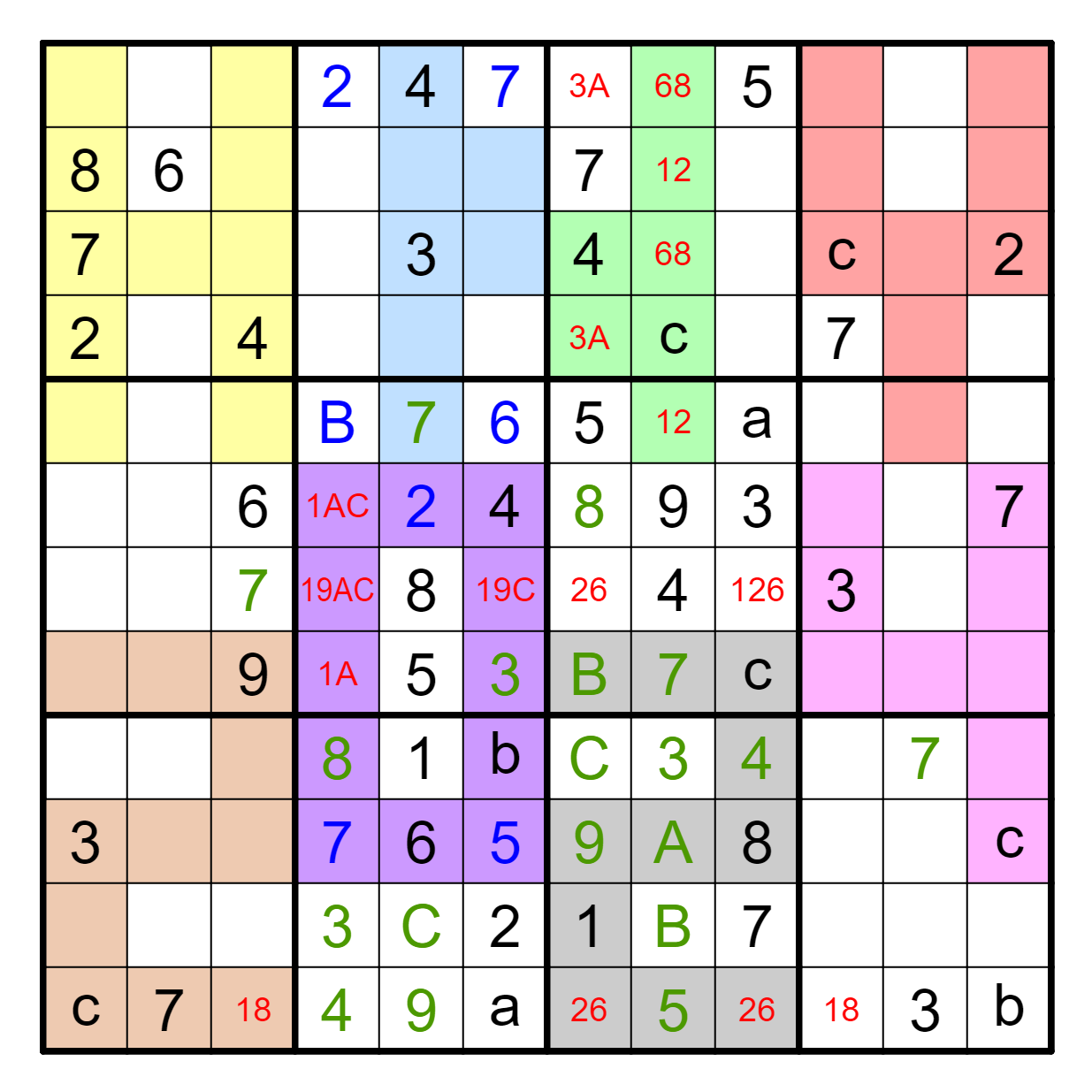
5:
The '0' is missing 1, 2, 9, A and C, so a 6 and a B can be placed in box 6. Looking at column 8 now, there is 1, 2, 6 and 8 remaining. There is a 1/2 pair, and hence a 6/8 pair. This means there is only one place for a 2 on the top row, and this places the final two 7s, and a 5. The 2 in the '0' can also be placed.
6:
Looking at the 'H' top left, the only digit that can be placed at the bottom left cell is a 1. This solves the 1/2 pair from earlier, as well as box 7 and the 2/6 pairs. A 2 can also be placed in box 3.
A 4/8/9 triple on the right leaves a 3/C pair on the left, and then there is only one place for an 8 in box 5. That then leaves one place for a 2 in the same box, and the 4 in the triple means there must be a 4 on the left of the 8th row.
Now the 8 in the first '2' of '2024' solves the 1/8 pair on the bottom row, and leaves only one place for an 8 in the 9th box.
7:
The 4 in the first '2' of '2024' leaves the 4/B pair as just a B, which gives a 2 and a 4 in the same box. There's only one place for a 2 in box 12, and that gives a 2 in the box above as well.
There are few enough remaining cells where all candidates can be filled to help solve. There must be a '6' in the 4 in row 8, meaning there is only one place for a 6 in row 9. There is a 5/A pair remaining in box 9, placing a 9. There is then another 9 in the topmost left cell.
8:
There is a 5/A pair in the '4' of '2024', leaving a 1/6 pair and a B can be placed. This leaves just one place for a B in the first column.
The 1/6 pair in the 8th box gives a C in the box and an A in the row. The C also gives a 1.
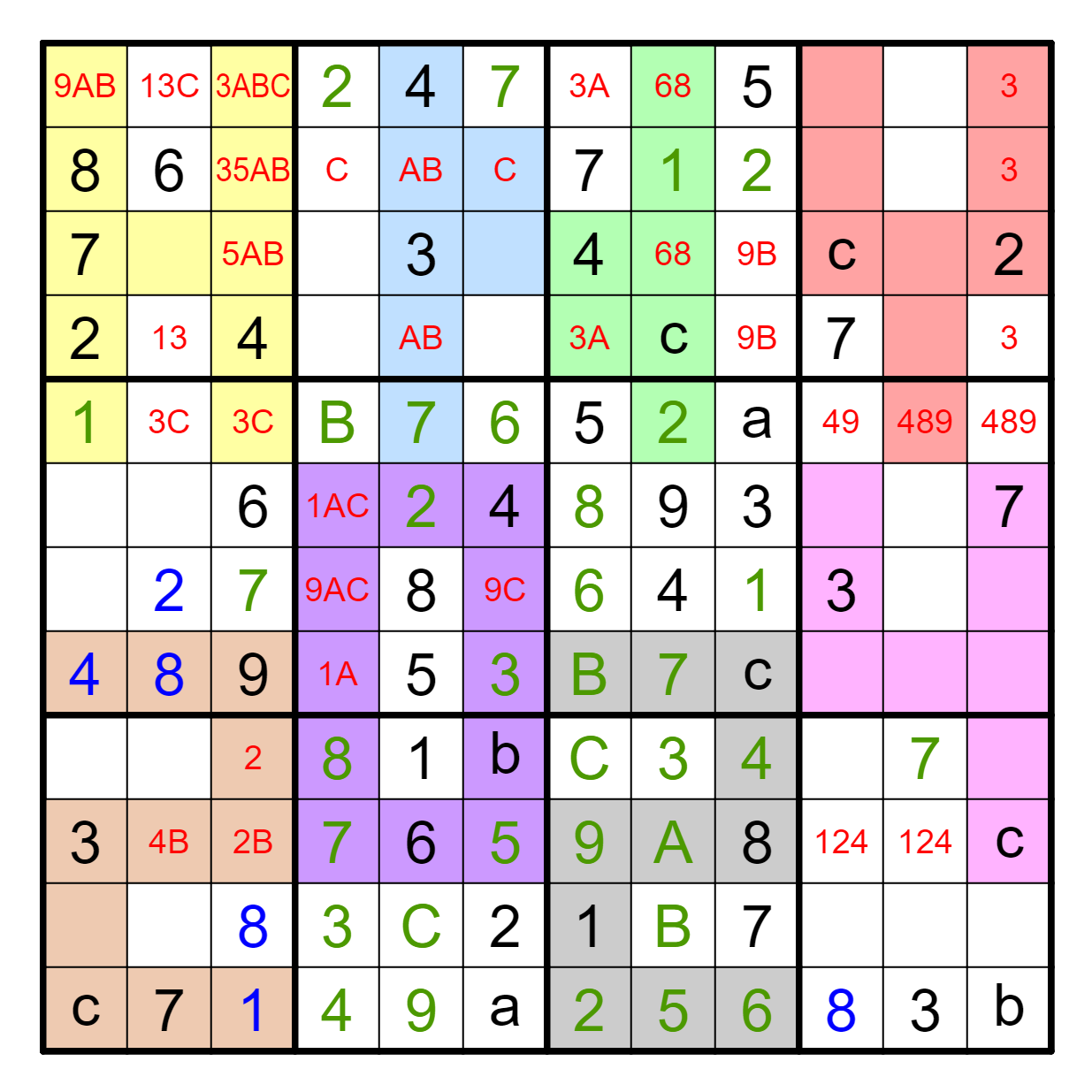
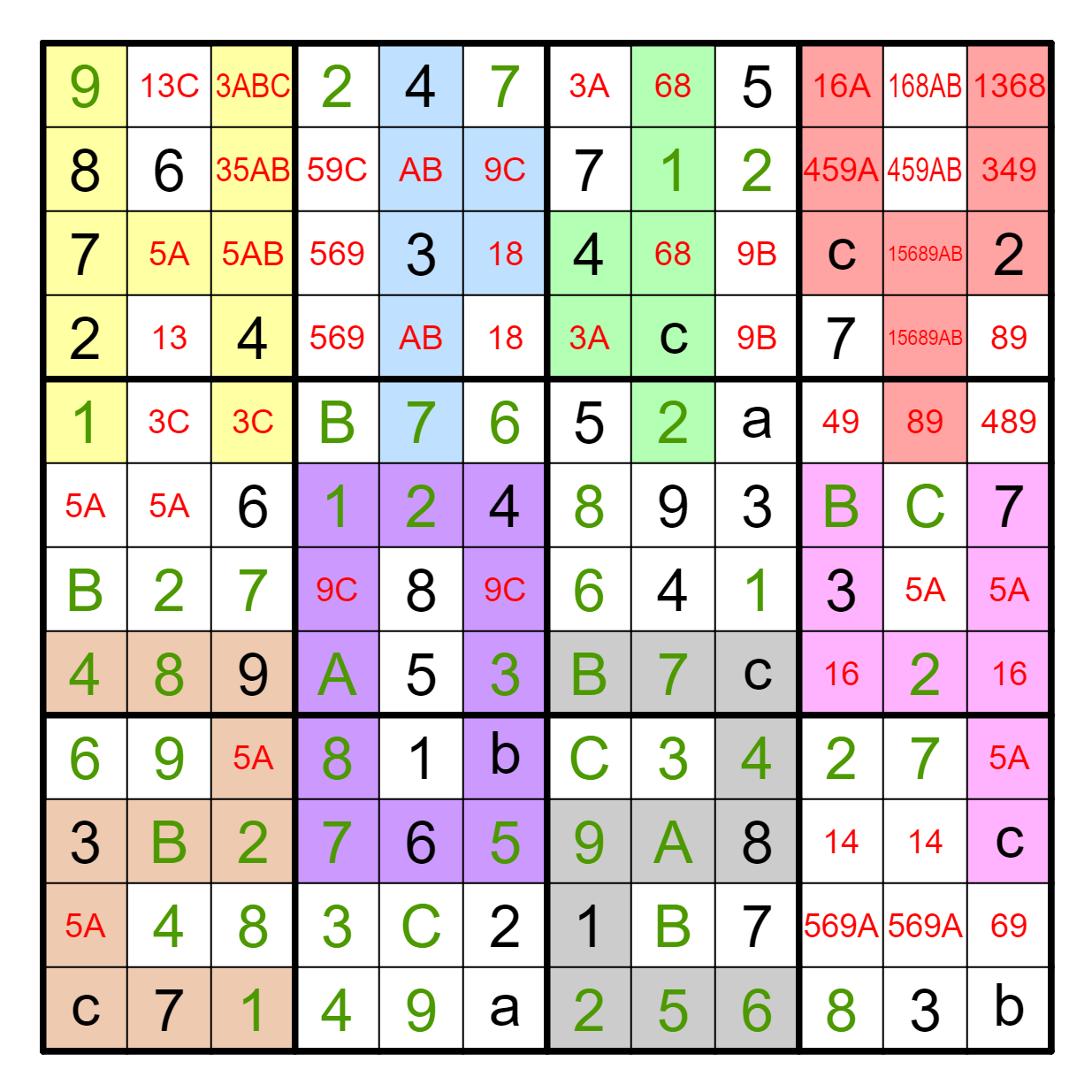
9:
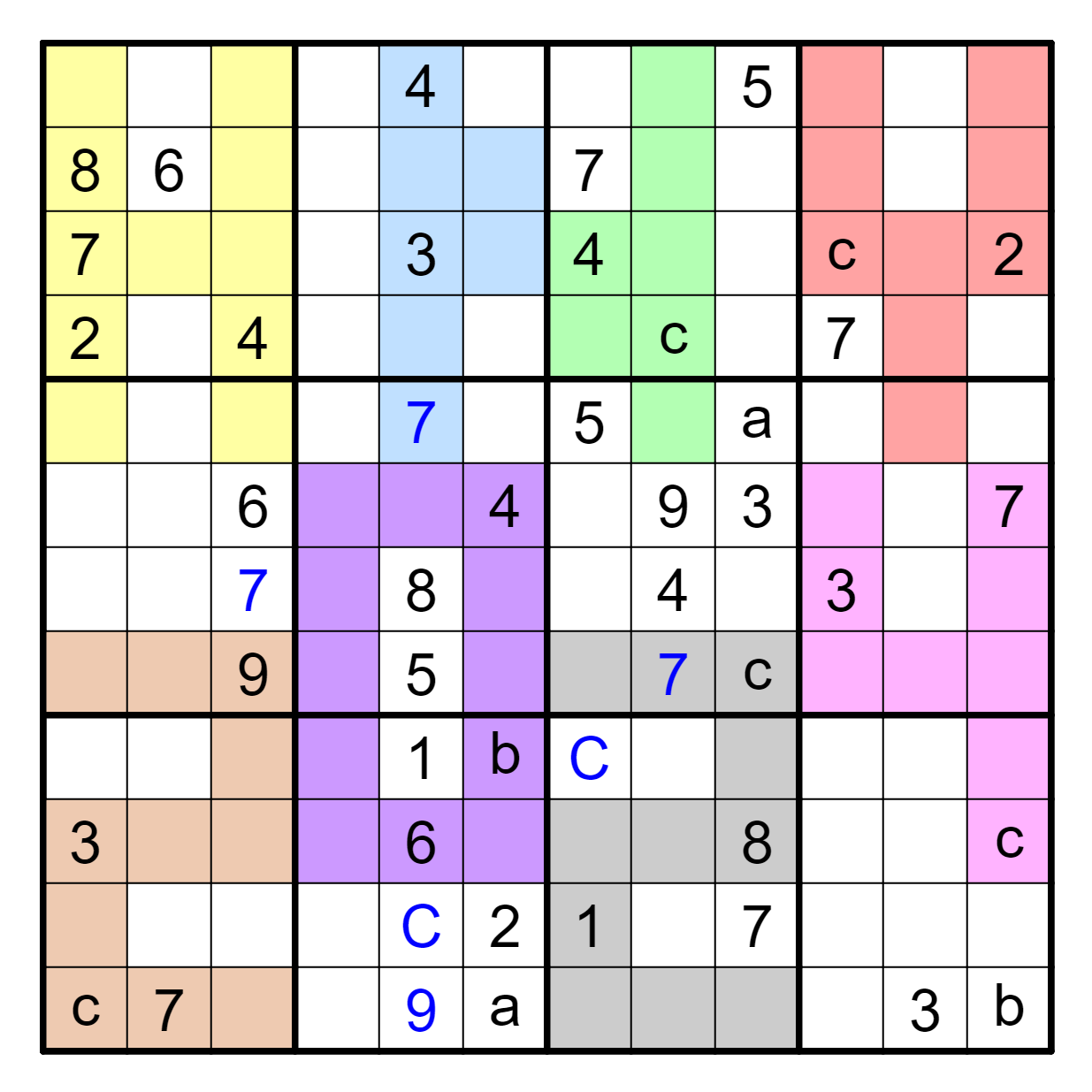
Consider the 'Y'. At the bottom of the Y, we know there is a 4, 8 or 9. If it is a 4, there will be no place for a 4 in box 4, so it must be an 8 or 9. The cell in row 4 column 12 has to be identical to the cell at the bottom of the Y, as that is the only cell in the box that is not 'seen' by the bottom of the 'Y'.
This gives this grid:
Now I've looked for a while but haven't found a stand-out piece of logic, but if the cell is a 9 it quickly transpires it doesn't work due to the A and Bs in the 3rd row. If anyone else can spot some logic here that would be great.
So based on this, the cell has to be an 8. This leaves one place for an 8 in box 4, and then there is a domino of simple deductions from pairs. The rest all falls into place with simple sudoku logic!
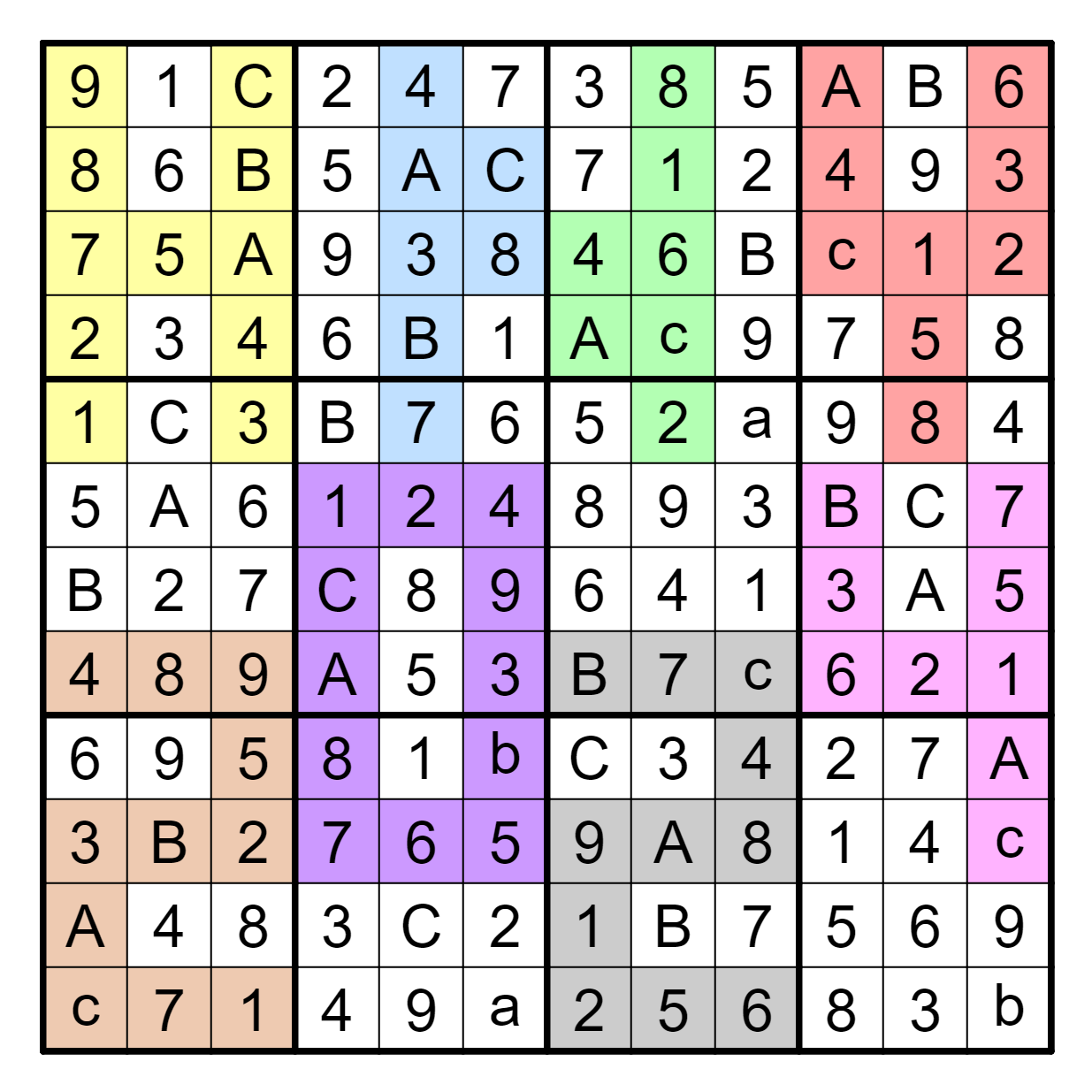
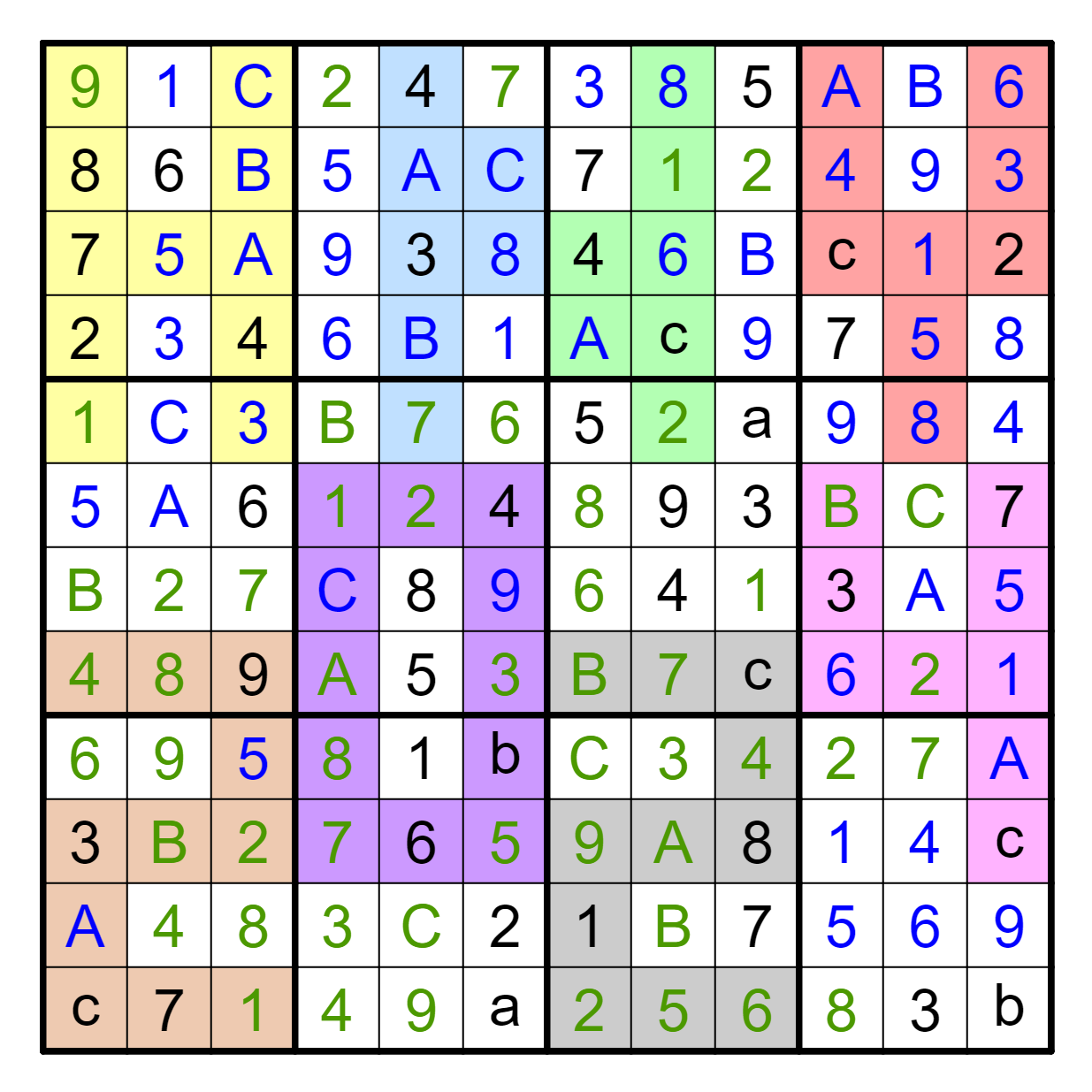
Leaving the final solution:
