0
$\begingroup$

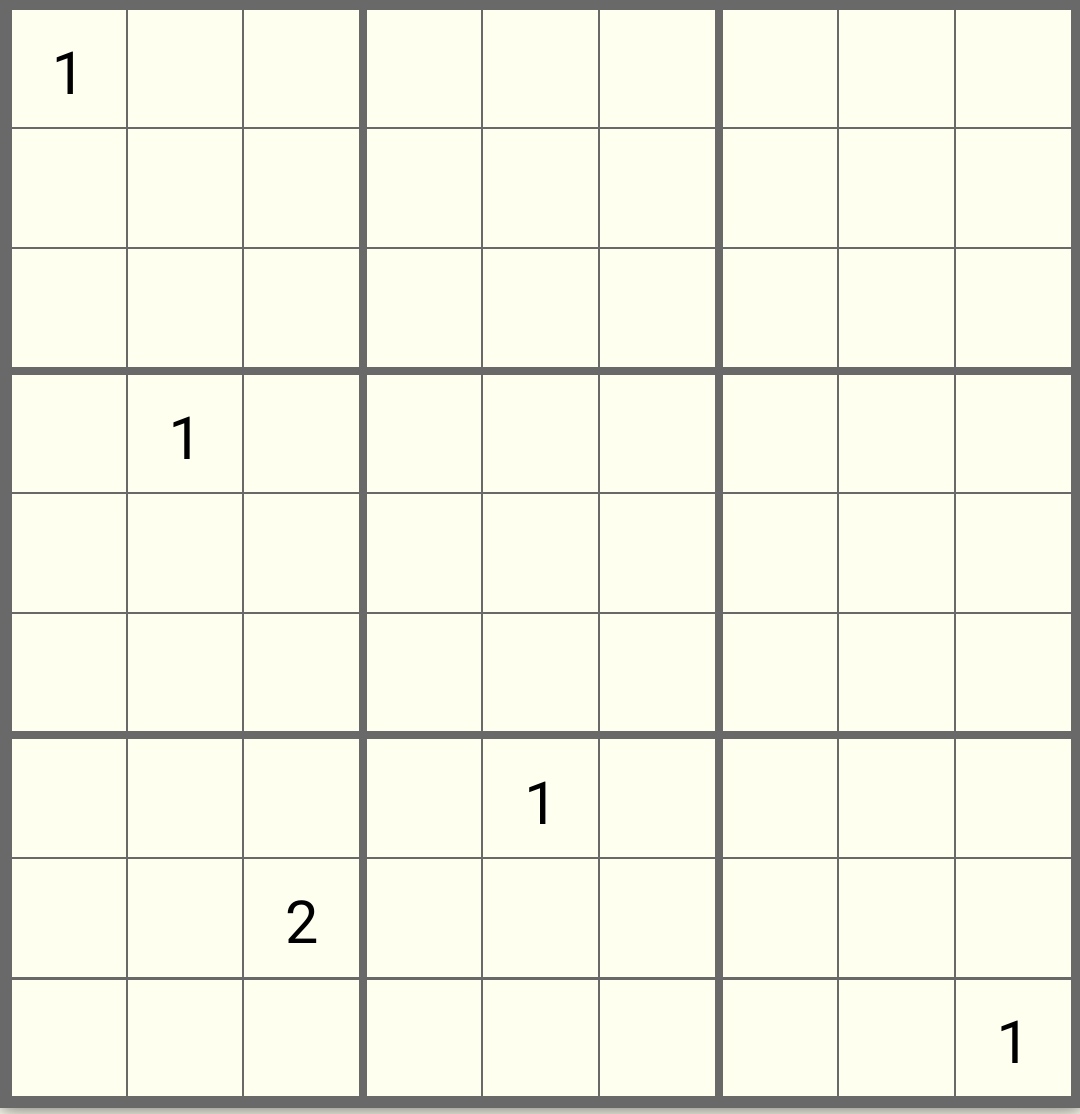
Does every validly posed Sudoku (doesn't break any Sudoku rules so only no duplicate 1 to 9 in rows, columns or square) have at least one solution, and if not, what is the minimum number of givens for it to be unsolvable?

According to Wikipedia:

The puzzle setter provides a partially completed grid, which for a well-posed puzzle has a single solution.

Many others sources including Sudopedia do not state that a Sudoku-like grid with given 1 to 9 numbers has to have one solution to be a Sudoku.

Does have given numbers mean it is solvable, and assuming no, what is the least numbers in a validly posed grid needed to be for it be unsolvable?

Improve this question
$\endgroup$
2
  • 2
    $\begingroup$ "Unsolvable" in the sense of having no completions? $\endgroup$
    – Parcly Taxel
    Commented Sep 22, 2021 at 12:58
  • $\begingroup$ A "validly posed grid" as in "you must make at least one deduction to know there can be no solution"? $\endgroup$
    – Bass
    Commented Sep 22, 2021 at 16:25

1 Answer 1

Reset to default
3
$\begingroup$

5 numbers are certainly enough to make it unsolvable. This is a simple example : grid created with sudoku9x9.com

You can never put one in the bottom left corner. Maybe someone has an optimization?

Improve this answer
$\endgroup$

Not the answer you're looking for? Browse other questions tagged or ask your own question.