5
$\begingroup$

A Jigsaw sudoku is like a regular sudoku, but with uneven shapes instead of 9 squares.

However, they still have the normal rules for rows and columns.

Would it be possible to have a jigsaw sudoku where there are 3 layers of irregular shapes, and no rules for rows/columns? Does any example of such a puzzle exist, or can you make one?

Improve this question
$\endgroup$
1
  • $\begingroup$ Based on Glorfindel's answer below, an additional desired restriction is that there are no sets of 2 or more squares that are in the same 3 shapes - as per regular sudoku. $\endgroup$
    – xorsyst
    Commented Jun 20, 2018 at 8:50

2 Answers 2

Reset to default
7
$\begingroup$

Sure, why not? The reason that they're not widespread becomes apparent when you try to make 2 layers visible:

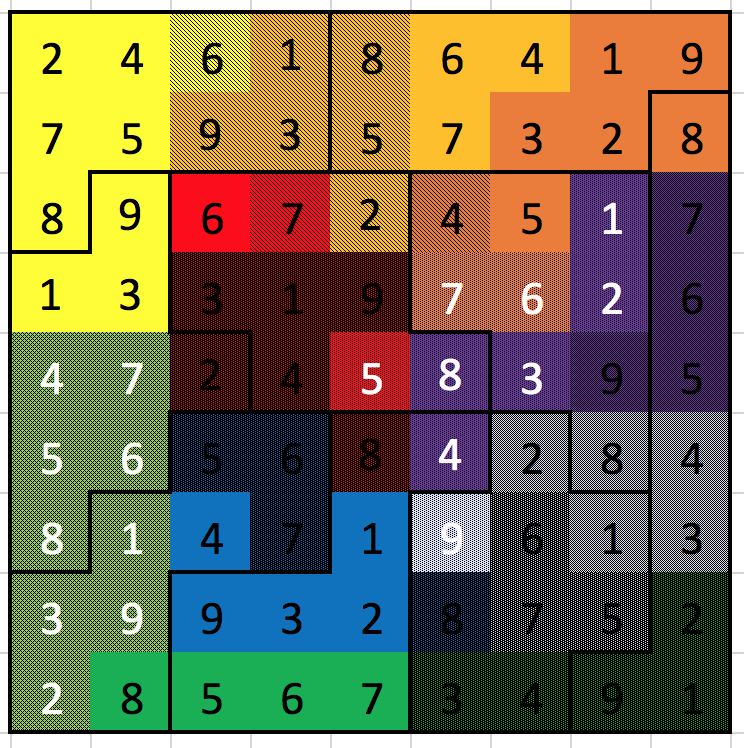
enter image description here

I'm having a hard time adding a third layer which is both easily distinguishable and still allows (black) numbers to be added. It might work if you have a different border style which is visible on enough places to make it clear; e.g. the top three 3x3 squares might work.

It is possible to construct 3 irregular layers which do not allow a solution, but I suspect most of them do. Here is an example; the third layer is visualized by background patterns and/or white numbers instead of black.

enter image description here

Improve this answer
$\endgroup$
8
  • $\begingroup$ How about outlines of shapes (diamond, circle, square, star, etc...) on each grid space? $\endgroup$
    – humfuzz
    Commented Jun 13, 2018 at 17:13
  • 1
    $\begingroup$ That might work as well, but I fear that especially when lots of numbers have been filled in it'll get unclear as well. $\endgroup$
    – Glorfindel
    Commented Jun 13, 2018 at 17:18
  • $\begingroup$ The question is though: Does at least one solution exist for such a puzzle? I guess there are even less possibilities for three overlapping jigsaws $\endgroup$
    – Narusan
    Commented Jun 13, 2018 at 18:32
  • $\begingroup$ Without the row/column constraints I don't see why not. $\endgroup$
    – phenomist
    Commented Jun 14, 2018 at 0:35
  • 1
    $\begingroup$ If you made a phone app (for example), you could have a switch to change the outlines shown instead of having all of the mess on screen at once. That way you wouldn't have to make sure to mark the same numbers in two spots, at least. $\endgroup$
    – Ian MacDonald
    Commented Jun 14, 2018 at 20:30
1
$\begingroup$

I agree with Glorfindel, it's definitely possible to create such puzzles.

However, there's at least one particular kind of grouping pattern that will immediately cause the resulting to grid to be un-sudoku-able:

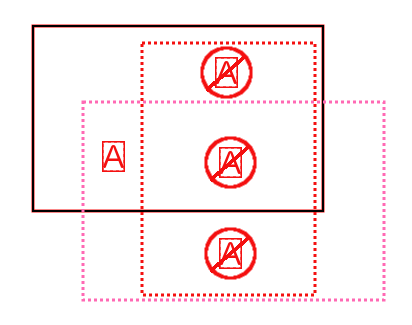
enter image description here

In the diagram there's a region (the one with the small red dots) that's completely contained within other regions that all overlap somewhere outside the contained region. Such overlap can be seen in the small rectangular area marked with $\color{red}{\boxed{\textbf{A}}}$.

In the picture, there are two such areas doing the containing and overlapping (the pink one and the solid black one), but there could, in theory, be more than two such areas involved.

The red region consists solely of those parts of the pink and black regions that are not $\color{red}{\boxed{\textbf{A}}}$. The red region therefore cannot contain anything that's in $\color{red}{\boxed{\textbf{A}}}$ either, so it's impossible to fit any kind of sudoku onto this pattern.

There are other, more (or less) subtle ways to mess up the groupings so that they cannot be sudoku-ed, but this seemed to be the simplest interesting one.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.