14
$\begingroup$

This is a Palisade (or 6 Cells) puzzle. The rules are:

  • Thicken some of the pentagons' sides so the thick borders outline regions of six pentagons apiece.
  • Any pentagon with a number in it indicates how many of its sides are thickened. Note that if the pentagon is along the border of the entire diagram, then that side counts as one of the thickened sides.
  • Thickened sides can be used only as parts of regions' outlines: no thickened side of a pentagon can have both of its sides in the same region.
  • Besides the outer border of the diagram, two sides are thickened to get you started.

Improve this question
$\endgroup$
1
  • $\begingroup$ This was nice. I solved it using similar logic to Deusovi, a few minutes at a time over a few sessions. Thanks! $\endgroup$
    – Harfatum
    Commented May 27, 2021 at 23:29

1 Answer 1

Reset to default
14
$\begingroup$

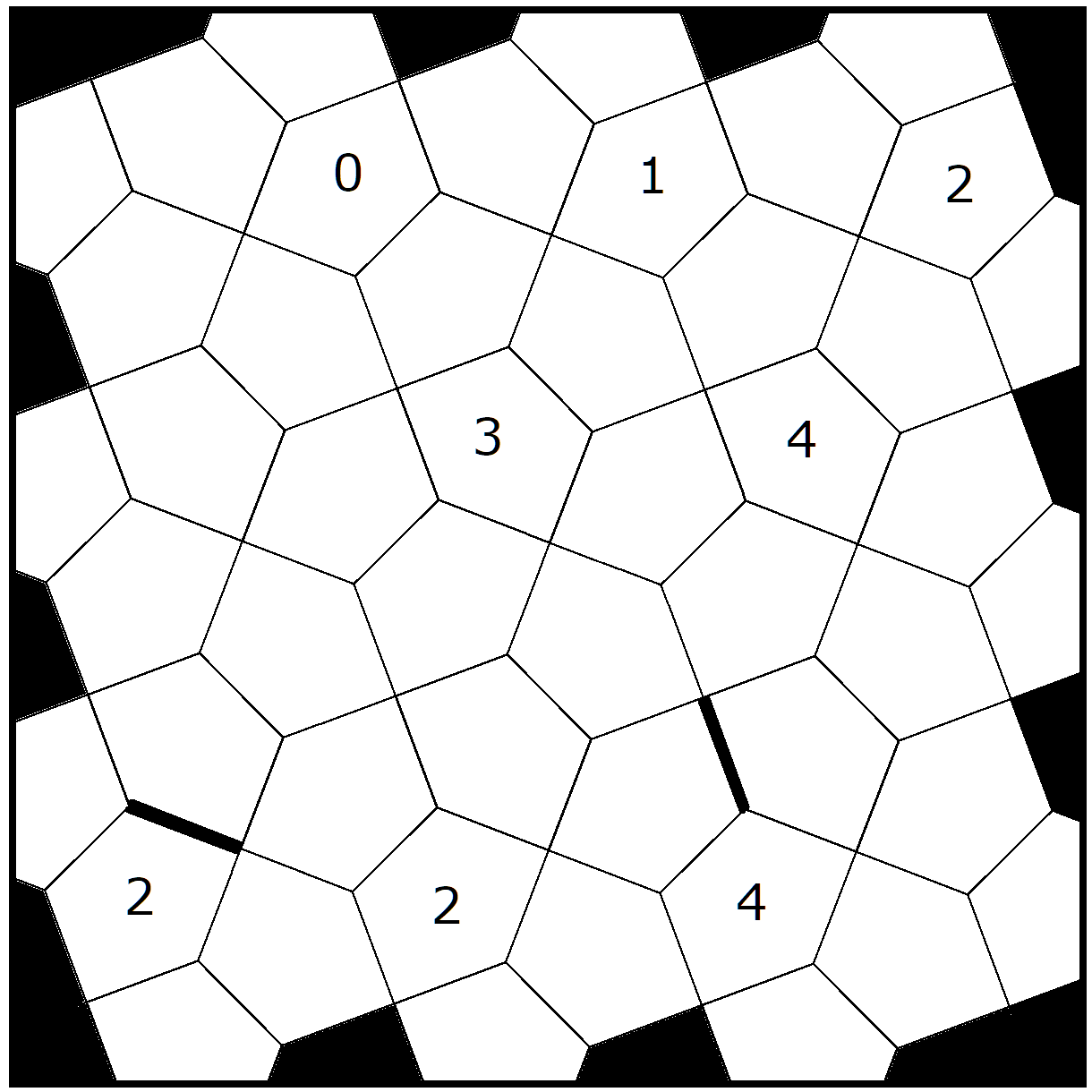
To start:

The 0 makes a 6-cell region by itself. This creates a wall by the nearby 1.
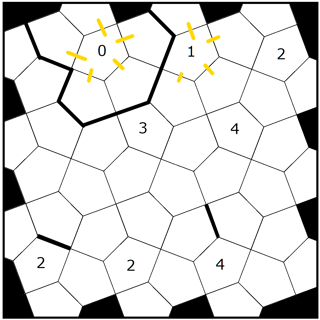
enter image description here

Then, make sure not to block off any cells where they can't be part of 6-cell regions:
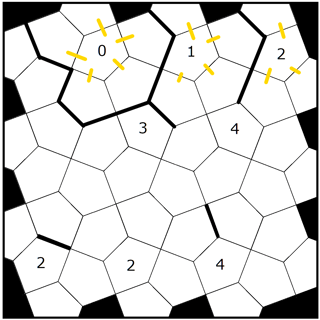
enter image description here

Next,

the 4 clue does some work - if it went up to join the 2, it would stop the 2 from making a region.
enter image description here
And we can do something similar with the 4 clue down below. (I also notice that the 2 in the bottom left is already satisfied.)
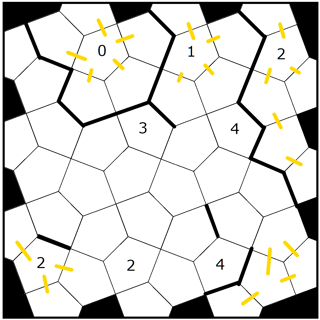
enter image description here

We can complete the bottom-right corner:

The 4 clue going up and right would block off the region under it. And if that region doesn't take both cells next to it, it blocks off the one it doesn't take.
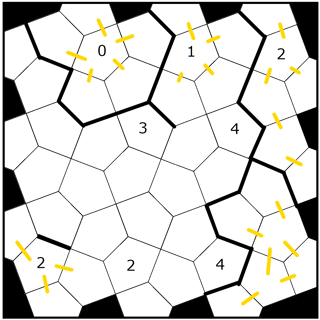
enter image description here
The 2 near the bottom middle cannot join with the other 2, because the region would be too large.
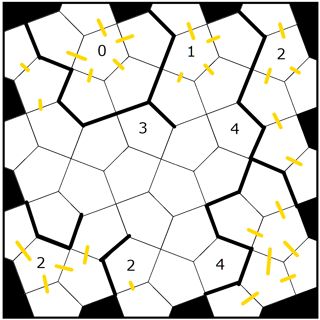
enter image description here

Now we've finished off a clue:

We've both of that 2's walls, so it claims the other three cells next to it; this makes the bottom-left region complete.
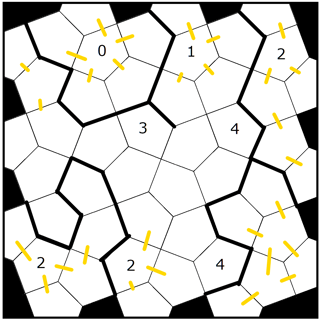
enter image description here
The chokepoint on the left blocks off six cells.
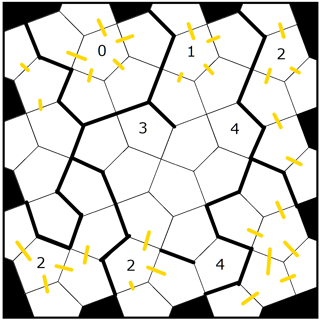
enter image description here
Putting a border on the left side of the 3 would cause it to gain a fourth wall. Putting a border on the right side of the 3 would either block the 2 region or cause it to merge with the 3 and be too big. So the 3's remaining border must be on the bottom.

This completes the 1 region, and the rest of the puzzle falls into place. The solved puzzle:

enter image description here

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ Good explanation. I'd appreciate your feedback on the puzzle. $\endgroup$
    – msh210
    Commented May 24, 2021 at 7:25
  • 1
    $\begingroup$ @msh210 It was pretty simple overall, but I had fun getting used to the logic of the genre. I liked how the ending required more complicated logic, too - overall it felt like there was a nice gradual increase in difficulty. $\endgroup$
    – Deusovi ♦
    Commented May 24, 2021 at 19:37

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.