Haisu: Schezo's Birthday

Question

Rules of Haisu:

1. Draw a path from S to G passing through all white cells exactly once by connecting the centres of adjacent cells.
2. When the path passes over a number N, it is on its N-th entry to the region which contains it.

---

The standard Haisu rules apply. However, all question marks should be replaced by the same number. This number must be deducted by the solver.

This puzzle celebrates the birthday of my favorite game character, Schezo Wegey, on March 16! :)

Answer 1

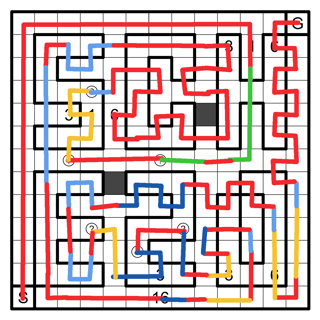
I think I got it:

Sorry about covering up some of the clues, they are pretty easy to figure out though, because they follow the 3/16 theme.

EDIT: Full write-up below, with different colours this time, since hindsight allows for more efficient deductions :-)

---

There are couple of easy deductions we can often make from the connectivity:

1. never close off an area
2. never almost close off an area; if there's only one entrance/exit, entering will create a dead end
3. corners have only two neighbours so the path through them is fixed.

With these in mind, let's start the puzzle. First, we fill in the easy bits, starting with the ones above. Then:

• There's only one path from S to the 1 in the big area.
• Question marks inside the same area must be connected.
• In the O, as well as the big area, the path through the 1 is forced by the neighbouring 3 and 6.

To reach the 3 in the big area we must pass through the H and the S, forcing us to travel through the question marks in the big area (which we now know must equal 2). We are left with a cul-de-sac at the top (the green line is of course only for illustration purposes), which we must immediately enter. There are a lot of digits, so the path is forced.

At the bottom, we have to have 4 entrances to the O between the 1 and the 6, so the path from 1 cannot go to the corner; the 6 would be left in a dead end.

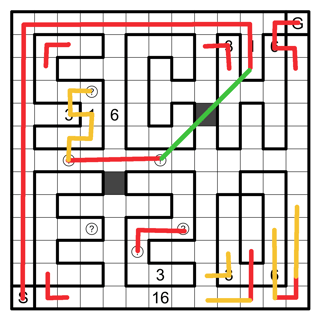
To turn the green "shortcut" into an actual path, we can now see that we need at least two entrances to the H on its left side, and three on the right side. This leaves only one possible path (with an optional tiny detour in the middle.)

Filling that path in, and again marking corners and unavoidable path extensions due to connectivity (the right hand side basically solves itself), we get to the most difficult bit:

To get ahead, we concentrate on the square marked A in the next picture. It constricts the path between the big area's 3 and 6.

In particular, if that path uses the square C and not the square B, then A becomes a fork in the road: whichever way we go, the other route will become a dead end.

Since the path cannot reach B before the big area's 6, the only option is that the path cannot use C either:

Connecting the rest on the top side is simple, so we have now reached the E. Counting the visits to the big area, we notice we are in a hurry: we must reach the 16 with the next visit.

Since the 16 is at the border, the path up to there is forced.

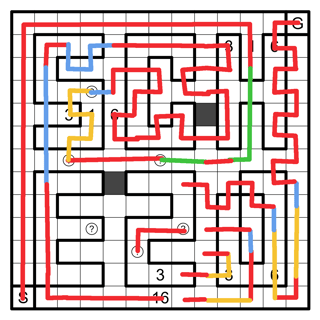
The digits in the Z block all other routes, so we get the Z done easily

and finally, filling in the corners of the E (light blue), and noticing that we must enter the middle prong first (orange), we get the final solution: