HAISU is a portmanteau of three Japanese words - 'hairu', to enter, 'su', number, and 'hausu', an English borrow word meaning house, of course.
Together, we get a meaning of 'enter number house', which I have roughly translated to English as 'Room Count'.
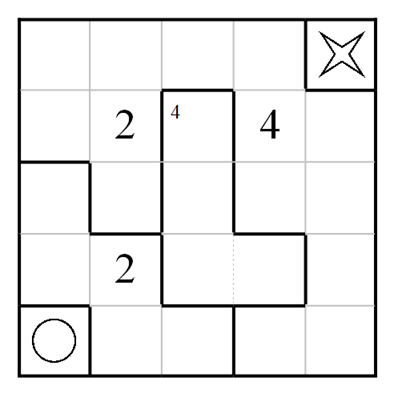
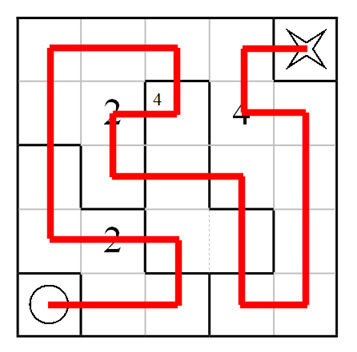
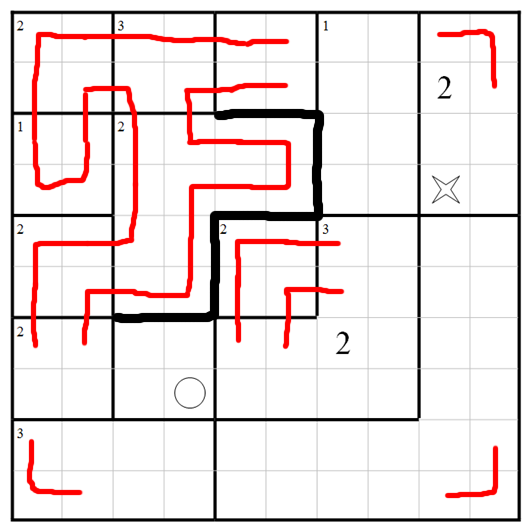
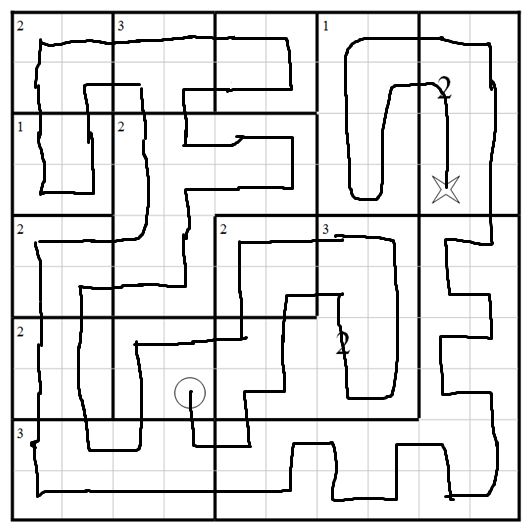
The rules are simple - draw a path from the O to the X, passing through every cell in the grid exactly once. The grid is divided into several rooms. When your path passes over a cell with the big number N, it must be the Nth time you have entered the room. If a room has a small number m in the top left corner, you must enter that room a total of m times. An example Haisu puzzle and its unique solution are shown below.
Hopefully this example puzzle clarifies the rules.
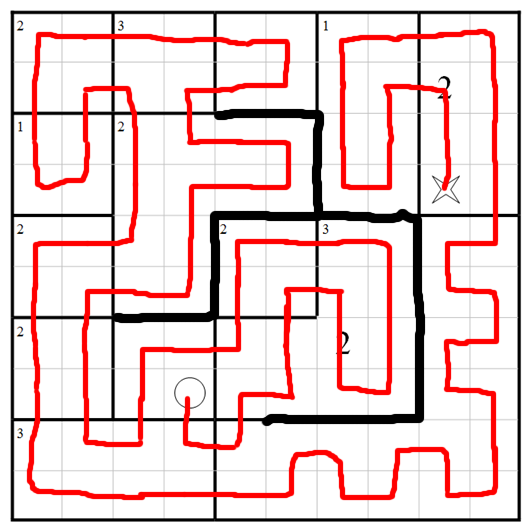
My previous Haisus have been over-complicated and/or difficult, so here is a puzzle which is MUCH more moderate in difficulty!