Tetrikabe: Parallelogram

Question

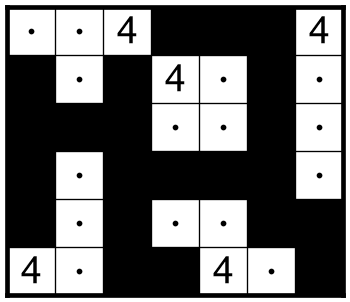
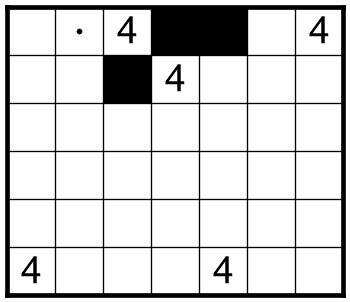
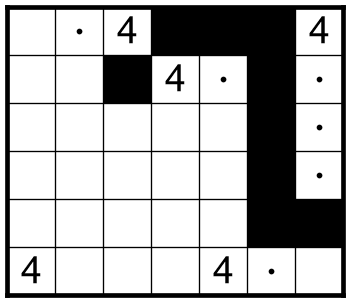
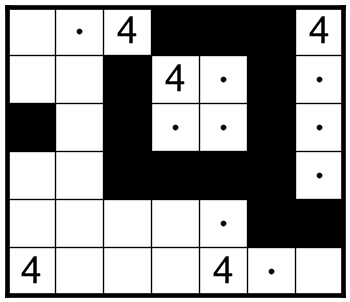
Rules: (Nurikabe section shamelessly stolen from an earlier puzzle by @jafe)

• Numbered cells are unshaded.
• Unshaded cells are divided into regions, all of which contain exactly one number. The number indicates how many unshaded cells there are in that region.
• SPECIAL RULE: the regions will form a tetromino set, with rotation and reflection allowed.
• Regions of unshaded cells cannot be (orthogonally) adjacent to one another, but they may touch at a corner.
• All shaded cells must be connected.
• There are no groups of shaded cells that form a 2 × 2 square anywhere in the grid.

I've included all available tetrominoes as a reference.

A playable version of this puzzle can be found here. The link leads to a puzz.link editor. Note that this editor won't force you to use the tetromino rule, and it has a timer.

The first answer with a fully-explained logical solution path will get the checkmark. I welcome multiple answers, if later ones can show a better-explained, more elegant, or significantly different path.

Tabular transcription good for copying into a spreadsheet (ignore the header row; the first row with a number is the first row of the puzzle):

		4				4
			4
4				4

Answer 1

Completed Grid

Reasoning

After the trivial initial shadings, we are left with:

Notice that this configuration leaves very few locations for the unshaded I tetromino; upper left is clearly blocked, and using either middle 4 cuts the grid into two disconnected halves. Finally, placing it on the 4 in the lower left would force R2C1 to be shaded and disconnected. This forces the I to use the top right 4. With some additional easy deductions, we obtain:

Now let's place the O:

The only possible locations for the O are in R2-3C4-5 and the bottom left corner. But if the O is placed in the bottom left corner, the upper left 4 must be an L to have an unshaded square in R3-4C1-2. This forces R3C3 to be shaded, which leaves no possibility for the 4 in R2C4, since it cannot be either S or T. With some additional follow-on trivial deductions based on reachability, this leaves us with:

Finishing up:

The L cannot go in the bottom right, since if it did, the bottom left would have to cover the R5-6C3-4 2x2, meaning it could not cover the R3-4C1-2 2x2, forcing the upper left 4 to be an L as well. Similar logic shows it cannot be T either: if it were, the lower left 4 would have to be an L to cover a square of R4-5C3-4, which would again again force the upper left 4 to be an L. So the lower right shape must be an S. Finally we must cover either R3C2 with a top left L, or R4C2 with a bottom left L, but the former case forces the bottom left to be a T which would block the shaded squares in the upper left corner from connecting. So we must have the answer above.