1
$\begingroup$

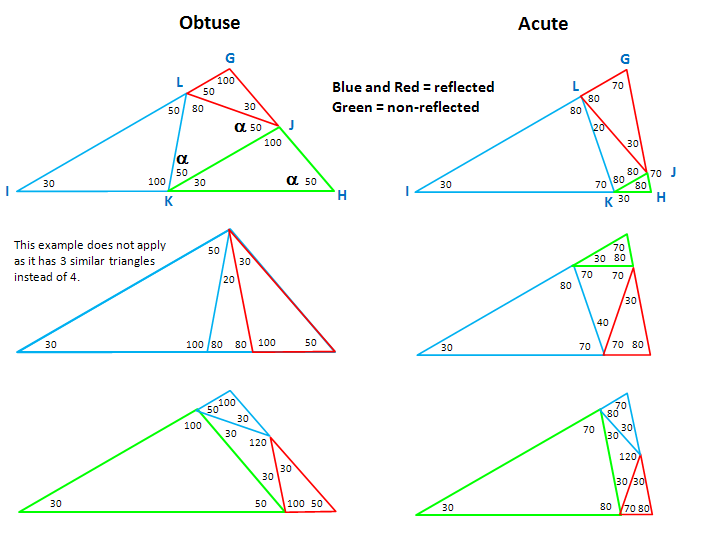
The challenge as described hereafter is to create a total of 4 similar triangles by drawing 4 triangle in a scalene, acute triangle - out of the 5 resulting triangles (4 that make the original one)

If D, E, and F are the middle of the sides of the triangle ABC than the 4 triangles created are equivalent and with the original triangle we get 5 similar triangles.

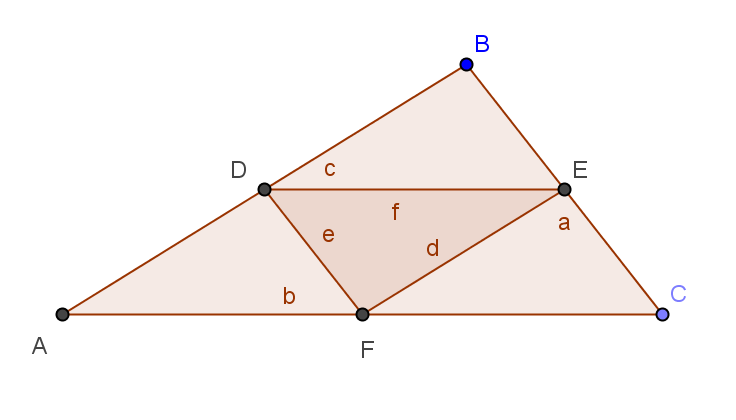
enter image description here

The challenge: In a triangle create four internal triangles as described in the following diagram. The requirement is that with the original triangle you get exactly FOUR similar triangles.

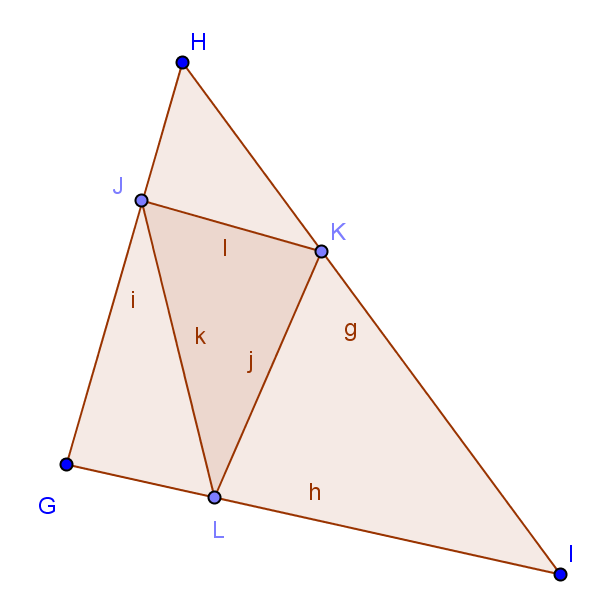
enter image description here

What is unique about the internal triangle KJL? What is unique about the points on the sides, K, J, and L?

Improve this question
$\endgroup$
8
  • $\begingroup$ How are there 4 similar triangles in the bottom picture? I don't even see 2 similar triangles $\endgroup$
    – Ivo
    Commented Feb 18, 2015 at 10:47
  • $\begingroup$ KL||HG, jk||GI, JL||HI $\endgroup$
    – Himanshu
    Commented Feb 18, 2015 at 12:18
  • $\begingroup$ @Himanshu that's not true. that is only the case if youmake the triangles like the top picture. $\endgroup$
    – Ivo
    Commented Feb 18, 2015 at 13:15
  • 1
    $\begingroup$ Please note that the questions are only for the second case. The first case is trivial and well investigate. $\endgroup$
    – Moti
    Commented Feb 19, 2015 at 3:39
  • $\begingroup$ Points K, J, L lie on the angle bisectors of the triangle GIH. $\endgroup$
    – user70926
    Commented Feb 28, 2015 at 18:53

1 Answer 1

Reset to default
2
$\begingroup$

In order to create four similar triangles instead of five, it is necessary to:

1. reflect two of the internal triangles (refer to examples below)

2. and place the third, non-reflected, internal triangle inside any vertex - provided this vertex has an angle less than $90^\circ$. Call this internal angle $\alpha$.

3. then form the base of an $isosceles$ triangle with the internal side of the non-reflected triangle

4. then use two equal lengths of the two reflected triangles and two angles equal to $\alpha$ to complete the internal, $isosceles$ triangle

The internal triangle KJL is unique because it is:

an isosceles triangle. Points J, K, L are unique as determined by the requirement to form an $isosceles$ triangle with 2 angles equal to $\alpha$.

Examples of obtuse and acute triangles with several placements of the internal triangles:


Triangles

Improve this answer
$\endgroup$
9
  • $\begingroup$ The claims are not correct. This is not a solution. I tried your claim using Geogebra and found easily cases when this is not working. You need to try in another way. The right solution will work for any scalene and acute triangle. $\endgroup$
    – Moti
    Commented Feb 28, 2015 at 16:47
  • $\begingroup$ @Moti - OK, I think this is a better answer. $\endgroup$
    – Len
    Commented Mar 1, 2015 at 2:12
  • $\begingroup$ Let me look at this in details. It could be correct! $\endgroup$
    – Moti
    Commented Mar 6, 2015 at 8:04
  • $\begingroup$ It has to be "scalene, acute triangle". So the $100^0$ is not a good example to start with. $\endgroup$
    – Moti
    Commented Mar 7, 2015 at 3:02
  • $\begingroup$ How exactly the reflection is carried out? Trial and error? $\endgroup$
    – Moti
    Commented Mar 7, 2015 at 3:04

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.