Given a triangle ABC with sides a=|BC|,b=|CA|,c=|AB| a diamond is circumscribed around the triangle's incircle. The diamond and the triangle share the corner C along with (part of) sides a and b.
Derive an expression for the diamond's side length d in terms of a,b and c.
Prettiest solution wins. (Elegant, minimal algebra derivation exists.)
Attribution: This puzzle was conceived by me.
As Bubbler already noted in a comment, the final formula is
$$d=\frac{2ab}{a+b+c}$$
To derive that, I will use the following facts and properties.
If you shorten or lengthen one side of a triangle by moving one vertex directly towards or away from another, then the area of the triangle is changed by the same factor as the side length. This is obvious if you consider that side as the base, and the fact that the area is proportional to the base when the height is kept constant.
The radius $r$ of the in-circle of a triangle with area $A$ and perimeter $p$ is given by $r=\frac{2A}{p}$. This is because if you dissect the triangle by cutting from vertices to the circle centre then you get triangles of equal height $r$. This is the basis for many cake cutting puzzles similar to Piece of Cake for King Solomon.
A rhombus can be split along its diagonal into two equal triangles. The area of a rhombus is equal to the height times its base.
Now let's put all this together:
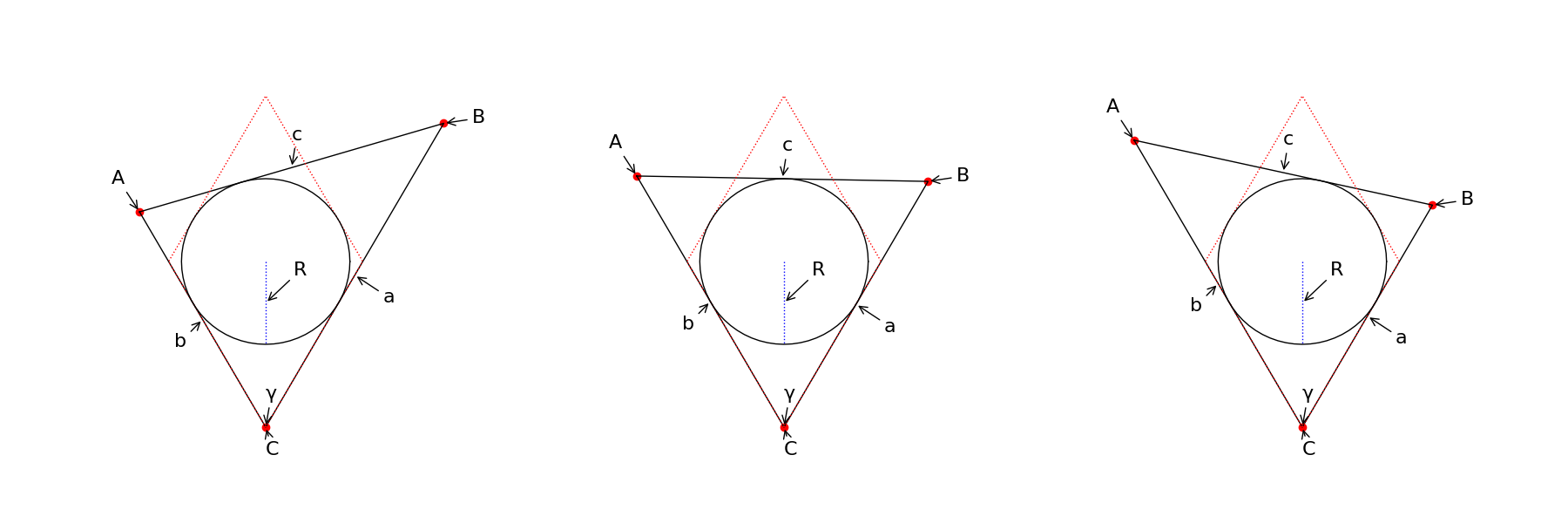
Start with the rhombus. It's area is $2r\cdot d$ because the incircle touches the top and the base.
Halve it along the diagonal not involving vertex $C$. The half that is the triangle with vertex $C$ then has area $rd$.
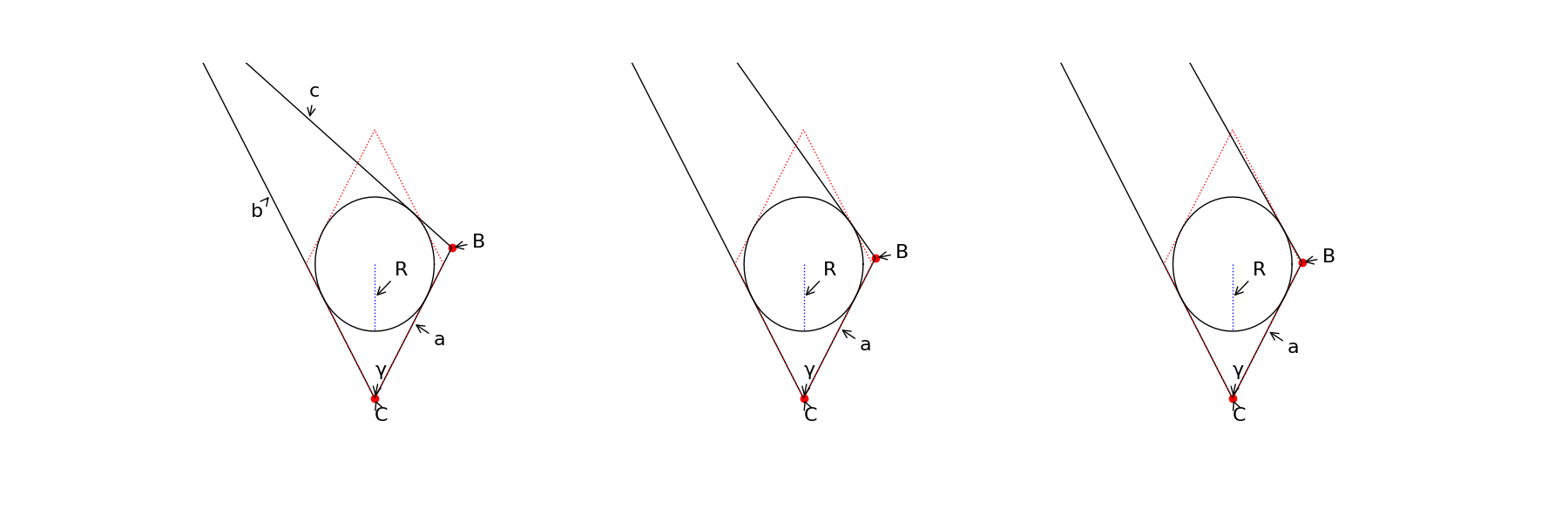
Lengthen one side from length $d$ to length $a$, and the other side from length $d$ to length $b$. You now get the original triangle, and from fact #1 we know its area is $rd\cdot \frac{a}{d}\cdot \frac{b}{d} = \frac{rab}{d}$.
Substituting this area into the formula #2 for the inradius $r=\frac{2A}{p}$, we get $r=\frac{2rab}{dp}$ which simplifies to $d=\frac{2ab}{p}$.
First, note that the diamond is fully determined by C and the incircle or, more precisely, by the angle γ at C and the incircle's radius R. Therefore the desired expression must evaluate to the same value for any triangle which gives rise to the same γ and R. Let us call M the set of all such triangles.
The M family of triangles
Observe that if we move one corner, for example A, farther and farther out then the adjacent sides b and c become arbitrarily long while their quotient converges to 1 (because by the triangle inequality their difference is bounded by a and a monotonically gets shorter in the process). They also become more and more parallel; consequently, the opposite side, a, converges to d, the side of the diamond.
Therefore, if we can find any expression in a,b,c that takes a constant value on M and we can solve for a when b and c are very large this will give us the needed expression for d.
To obtain an expression that is constant on M recall that M is by definition where R and γ are constant. We can link these to the side lengths a,b,c using two well known expression for the surface area S of ABC:
½ ab sin(γ) = S = ½ R(a+b+c)
Moving all occurrences of a,b,c to one side we obtain
z := R / sin(γ) = ab / (a+b+c)
which is indeed constant on M. Solving for b -> ∞, c -> ∞, b/c -> 1, a -> d as described above yields simply d = 2 z. The complete formula is therefore
d = 2 ab / (a+b+c)
sqrt((a+b+c)(a+b-c)(a-b+c)(-a+b+c)), or even better,sin C. $\endgroup$/in the middle. $\endgroup$