5
$\begingroup$

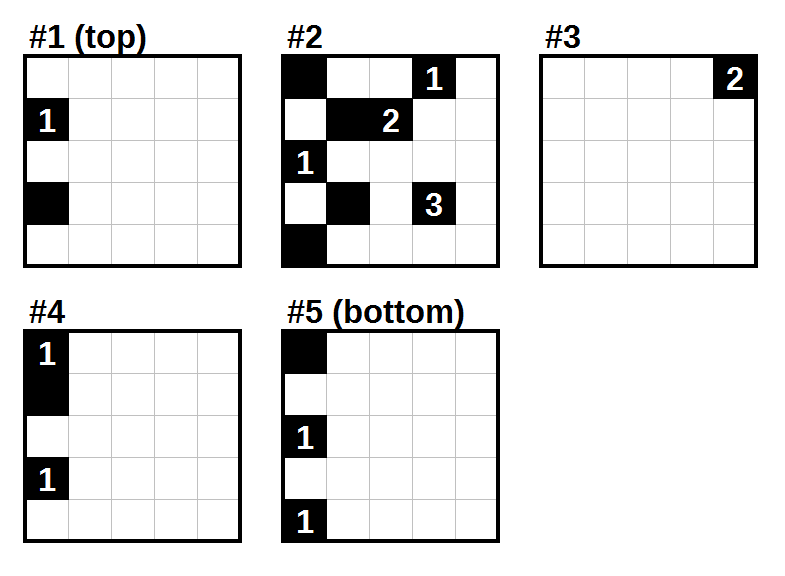
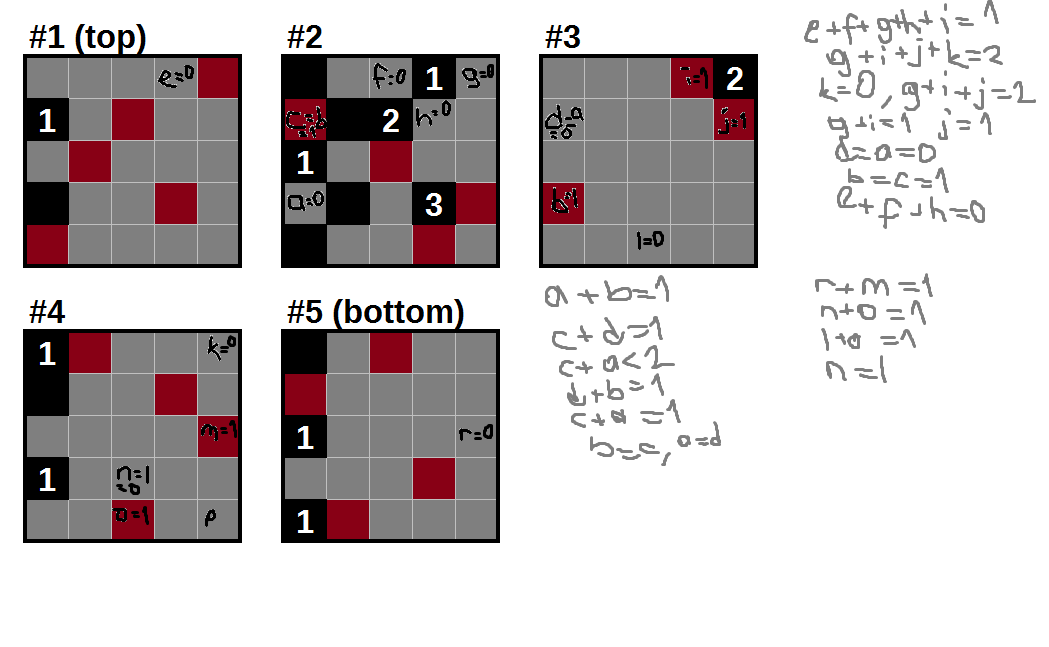
This is a three-dimensional Akari puzzle (also known as Light Up). The five squares represent the layers of a $5\times5\times5$ cube, top to bottom. The objective is to add light bulbs into any number of cells so that the resulting grid satifies the following rules:1

  • Black cells are walls and cannot contain light bulbs.
  • Numbers in black cells indicate how many light bulbs are directly adjacent to that cell (vertically, horizontally or along the Z-axis).
  • A light bulb illuminates its own cell as well as every cell visible from it in all six directions (up/down, right/left, and both ways along the Z-axis), continuing until a wall comes in the way.
  • Every white square must be illuminated by at least one light bulb.
  • No light bulb may be illuminated by another light bulb.

Note: The solution is unique and solvable by logic alone. No guesswork or trial-and-error is necessary here.

enter image description here

1 Paraphrased from the original rules on Nikoli

Improve this question
$\endgroup$

1 Answer 1

Reset to default
6
$\begingroup$

Took some notes on the picture:

enter image description here

Improve this answer
$\endgroup$
3
  • $\begingroup$ This is correct. Nice work! $\endgroup$
    – Jafe
    Commented Jul 20, 2019 at 16:51
  • $\begingroup$ #3 column 2 row 3 - what bulb lights that cell? $\endgroup$
    – Jasen
    Commented Jul 20, 2019 at 21:01
  • $\begingroup$ There's an unblocked bulb in the top layer, on c2, r3. $\endgroup$
    – Nautilus
    Commented Jul 20, 2019 at 22:32

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.