The image below shows one large rectangle, with smaller rectangles inside it. Your task is simply to calculate the area of the red rectangle, marked with an x.
Note that I have deliberately made the height and width of the rectangles incorrect, to avoid solutions using measuring tools.
It looks like
$x$ = 18.
Explanation:
I originally thought the derivation must be on similarity of the rectangles, e.g. the blue 48 and green 40 must be in the same proportion as the one I marked 30 vs. the yellow 25.
However, that is not enough to uniquely determine the solution, we need to use either the given height (18) or width (16) as well.
Assuming integer lengths, the 25 must be 5x5, so the 30 is 6x5 and $x$ has a height of 6. The left column has a width of 5, the right column 48/6 = 8, so there's 3 left of the width of $x$.
So $x$ = 6x3 = 18.
Glorfindel has found the answer; here is a uniqueness proof.
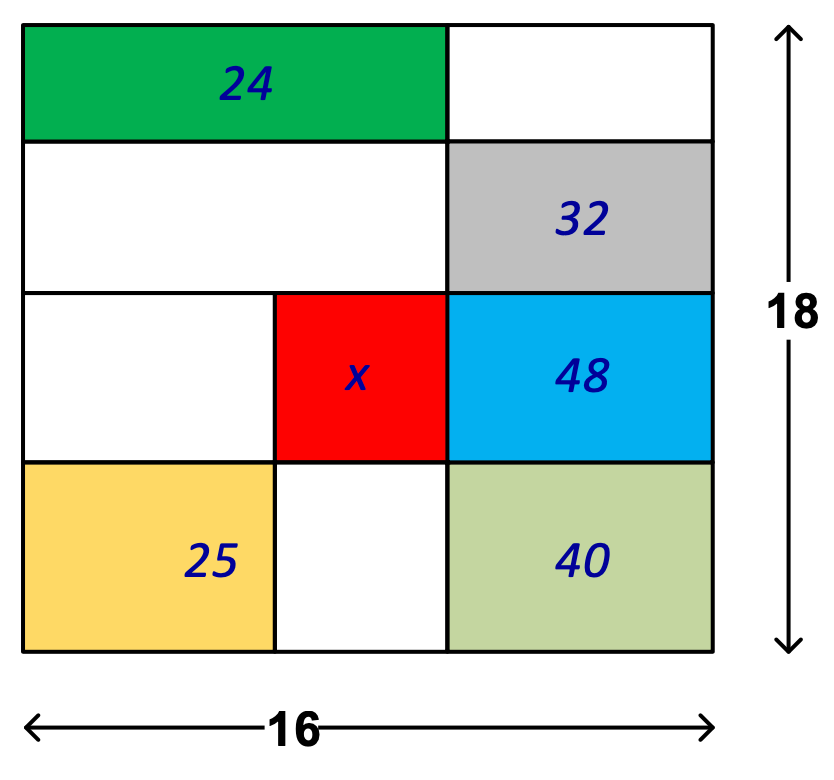
Write $a,b$ for the column widths; $c,d$ for the heights of the first row and the remainder of the rows together. Comparing the $24$-cell at top left and the $25$-cell at bottom right we can see that $c<d$.
Now
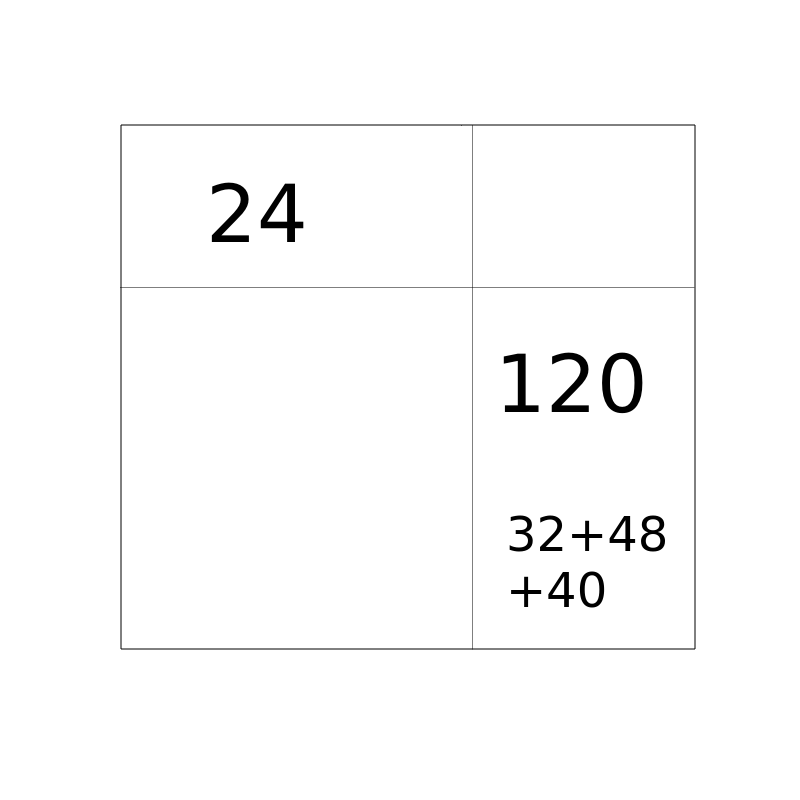
we have $a+b=16$, $c+d=18$, $ac=24$, $bd=120$. Using the first two to replace $b$ with $16-a$ and $d$ with $18-c$ we get $ac=24$, $(16-a)(24-c)=120$; simplifying the latter (and using the former to get rid of its $ac$ term) we find $18a+16c=192$ or $9a+8c=96$. Writing $\alpha=9a,\gamma=8c$ we now have the sum and product of $\alpha,\gamma$, so they satisfy the quadratic equation $x^2-96x+1728=0$ which we can solve either by inspection or by plugging into the quadratic formula: $x=24,72$. We can assign these to $\alpha,\gamma$ either way around. One way yields $a=8,c=3$; the other way yields $a=8/3,c=9$. The second solution is inconsistent with the relation $c<d$ we found before, so the first must be correct.
And now
the rest is routine.
Here is the answer drawn to scale.
Analysis:
There are 4 widths and 3 heights, for a total of seven unknowns. You can write this as a system of seven equations (5 for the colored rectangle areas, and two for the sum of the sides). As others have shown, the system has two solutions, only one of which makes sense (unless you allow rectangles to overlap). You can reasonably arrive at the solution by guessing that the bottom left rectangle has dimensions 5 by 5 and the rectangles on the right have a width of 8. That's what I would do in a time crunch.
Another answer, slightly simpler
Let's consider this part first.
Assume that the right part of the rectangle is $A$ times larger than the left one, so the area of the lower-left rectangle is $\frac{120}A$, and the upper-right one is $24A$. The total area of the all 4 rectangles is $16\times18=288$, so we get $$24+24A+\frac{120}A+120=288, \mathrm{or}\ 24A+\frac{120}A=144.$$ Multiplying by $\frac{A}{24}$, we get $$A^2+5=6A.$$ The only solutions to this are $A=1$ and $A=5$. But the latter is not suitable for the problem, since the area of the lower-left rectangle must be $\geqslant25$, but we get $\frac{120}5=24$. So, $A=1$, and we get the width and heights of the given rectangles:
(First, all the areas written in black are now obvious. Second, we notice that the 25 rectangle has a height of 5, so it's width must be also 5. Now, areas written in red are obvious too.)
Final answer: $x=18$.
Let the top-right empty rectangle have dimensions $w$ and $h$. Then we have: $$wh+(16-w)(18-h)=144$$ by equating the known areas.
Expand and simplify: $$144-18w-16h+2wh=0$$ $$72-9w-8h+wh=0$$ which factors:$$(8-w)(9-h)=0$$
So either $w=8$ (and $x=18$ from the 48-box has height $6$ and the $40$-box has height $5$) or $h=9$.
If $h=9$, as the remaining boxes in the column have ratio $4:6:5$ of heights, they have heights $\frac{12}{5}, \frac{18}{5}, \frac{15}{5}(=3)$. Therefore $x$ has width $16-\frac{65}{3}=\frac{-17}{3}$, for a total area of $\frac{18\times-17}{5\times3}=\frac{-102}{5}$ which is impossible.
