A cryptic tricolour

Question

_{This puzzle is part 8 of Gladys' journey across the globe. Each part can be solved independently. Nevertheless, if you are new to the series, feel free to start at the beginning: Introducing Gladys.}

---

Dear Puzzling,

As I'm writing to you, I'm looking at an extremely impressive statue. This marks a big milestone for my trip. I certainly could have stayed in my previous destination for a bit, but the way I have planned the trip means I'm going to spend more time in certain parts of the world than others. I'll write you again soon. Take care!

Wish you were here!
Love, Gladys.

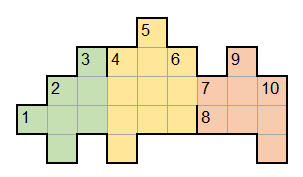
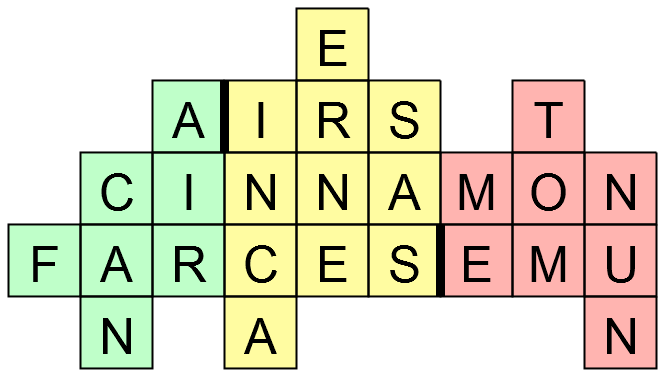
Across
1. Comedies face having romantic lead discuss ending (6)
2. Disguised con man carrying fashionable spice (8)
4. Tax organization fires leading financier, leaving no evidence behind (3)
8. Reject rights to consume bird (3)

Down
2. Create digital copy of unopened container (3)
3. Leading automobile innovator's radio broadcast (3)
4. Native American's piece of raisin cake (4)
5. Bird central to western epic (4)
6. Airline buried in disasters (3)
7. Formerly central Windows version (2)
9. Cat takes on mouse initially (3)
10. Woman with habit to turn right before finishing run (3)

---

_{Gladys will return in "Gladys visits a campus".}

Answer 1

The filled grid:

Brief clue explanations:

* is anagram, < is reversal, _TEXT_ is hidden word, A_ or _A is first/last letter extraction.

1A: FA(R_)CE + S
2A: C(IN)NAMON*
4A: (-f_)IR(-_e)S: parsed as "tax organization = FIRES, "leading financier" leaving, no "evidence behind" "
8A: (EMU_)<

2D: (-s)CAN
3D: AIR (initials)
4D: _IN CA_
5D: _ERN E_
6D: _SAS_
7D: _ME_
9D: TOM (initials)
10D: NUN (some letter change with "run"?)

The location is

found by anagramming the three regions independently: each makes a word, giving the location AFRICAN RENAISSANCE MONUMENT. (This is in Senegal, as shown by the green-yellow-red background representing its flag.)