A 3 x 1 rectangle has a perimeter of length 8. Two of these rectangles can each be bend in a U-shape and stitched together to yield a cube of unit volume.
Surely we can do better than that!
What is the minimum stitch length required to create a unit volume out of two identical (bendable but not deformable) planar shapes?
Note: any stitching required to 'seal' the volume (including stitching required between parts of the same shape) counts towards the total.
Edit: I found a better way to use this technique, and the answer becomes "how small do you want it?"
Consider the following simple paper template:
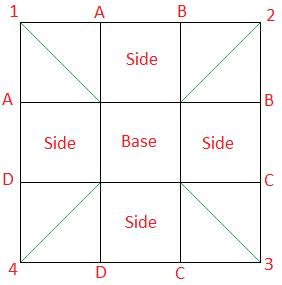
Now fold this along the black lines so it forms an open-topped cube. The two points marked A must meet, as do B, C and D. This leaves four flaps - fold them along the green lines, and then along the sides of the cube so that 1 meets B, 2 meets C, 3 meets D, and 4 meets A. If the cube faces all have side $\frac{1}{2^{1/3}}$, then the cube has volume $\frac{1}{2}$. Sew two of these together, and you enclose a unit volume.
Now the stitching must go through three layers of figure, but the overall length of stitching is only $\frac{4}{2^{1/3}} = 3.1748$
That's pretty small, but you can improve it by making your two boxes taller because the stitching length only depends on the width. So if we make the height of the boxes a multiple n of the width, then we have $nw^3 = \frac{1}{2}$, so $w = \frac{1}{(2n)^{1/3}}$ and the stitching length 4w approaches zero as n increases without bound.
The price you pay for all of this is sewing multiple thicknesses of material. You will need to sew through 2n + 1 layers of material.
Old answer:
I'm going to bend the rules a little, since there's nothing to say every part of the shapes has to be part of the volume boundary.
You can make a tetra-brik ( http://en.wikipedia.org/wiki/Tetra_Brik ) with height h and square cross-section of side w, out of a single shape which is 4w wide, and h + w high. It has a stitching length of 5w + h (one stitch length up the side to make a tube, and 2w stitch length at each of top and bottom to seal it).
Once you've done that, you can either use a double thickness (both shapes) to make your brik, since the same stitching will attach both; or you cut an infinitesimal hole in the brik and patch it with the second planar shape.
The resulting stitch length:
The volume of the brik is $w^2h$, which must be 1. So $h = \frac{1}{w^2}$.
Stitch length $s = 5w + \frac{1}{w^2}$
$\frac{ds}{dw} = 5 - \frac{2}{w^3}$, so the minimum is at $w^3 = \frac{2}{5}$.
This gives you a square cross section area of $(0.736806299728077)^2 = 0.54288352331898$, and a height of 1.8420157493202, for a total stitch length of 5.5260472479606
I really wanted to make a volume that genuinely used parts of both shapes - say a cone that simply had the other shape stitched flat across the open end - but I couldn't get one that was actually better than two cones. I promise an upvote to the first person who can do that.
I believe the minimum is about $5.88$, and is achieved by a Mylar balloon shape.
Let me introduce you to the magic of paper pleating:
The Nash-Kuiper embedding theorem $-$ well, a specific case of it, translated into English $-$ says that we can pleat paper into any shape, wasting as little paper as we want to. The only condition says that the paper can't get bigger. That is, if you imagine the paper as a fully stretched sheet of rubber, then the rubber can't stretch any more, it can only shrink. I recommend image searching "pleated paper"; it looks cool.
With that in mind, we have a hard math problem on our hands, from the calculus of variations. The Mylar balloon solves this problem (thanks Florian F), and looks like this (diagram from Wikipedia):
(To get the Mylar balloon, rotate this image around the vertical axis.)
To create your very own Mylar balloon, get a circle of paper of radius $a+\epsilon$, where $a$ is the arc length from the diagram. Pleat it into an approximation of the top half of the Mylar balloon. Slice off a plane very close to the equator, so that the boundary of the remaining squashed-hemisphere of paper lies on a plane. Reflect to get the bottom half, and stitch along the boundary. Voila, a (very good approximation of a) Mylar balloon of volume 1.
Using the formulas in the Wikipedia page, we find that for a Mylar balloon of volume 1, each of the papers is a circle of radius $a \approx 0.936$. Therefore, the length of stitching required is $2\pi a\approx 5.88$.
Two of the answers given so far use cones. To optimize this idea, suppose we take two identical circular sectors, form them into right circular cones of radius $r$ and height $h$, and attach them along their bases. The total volume will be $\frac{2}{3}\pi r^2 h$ (twice the volume of a cone of radius $r$ and height $h$), and the length of stitching is $2\sqrt{r^2+h^2}+2\pi r$ (each cone requires $2\sqrt{r^2+h^2}$ stitching to assemble, and it requires $2\pi r$ stitching to attach them). We should try to minimize the quantity $2\sqrt{r^2+h^2}+2\pi r$, subject to the contraint $\frac{2}{3}\pi r^2 h=1$.
The minimum can be computed using the differential calculus. It's a big mess, but the result is: $$ r=\sqrt[6]{\frac{-9-\frac{36}{\pi^2}+9\sqrt{1+\frac{24}{\pi^2}}}{8(\pi^2-1)}}\approx 0.619857783, $$ $$ h=3\frac{\sqrt[3]{\frac{8(\pi^2-1)}{-9-\frac{36}{\pi^2}+9\sqrt{1+\frac{24}{\pi^2}}}}}{2\pi}\approx1.242674164. $$ This gives a total stitch length of about $6.672063354$.
Edit:
Aha, I was looking into cones, and I think I've figured out the ratio. Make a cone where $h=\frac{2{\pi} r}{3}$ That works out to $r^3=\frac{9}{4\pi^2}$ which is aprox 0.611. With a circumference of 3.839 and a lateral length of 1.418 * 2, it gives a total stitching of aprox $6.675$
Old:
An octahedron of volume 1 would have an edge length of 1.285. The planar shape you would need two of would be 4 equilateral triangles in a U shape. There would be 6 stiches. 1 on each piece to turn the U shape into a bottomless pyramid (2x), then 4 to put the two pyramids together.
Total stitching: 7.71
I was gonna try a dodecahedron and an icosahedron, but each of those has way too many stitches (20 and 12).
