9
$\begingroup$

Given the following shape - an hexagon ABCDEF of which a parallelogram CDGH is cut out. With a single cut divide the shape into two equal area shapes by means of an unmarked .

You may draw lines and points to find the cut. It is not too hard but interesting feature to exercise.

enter image description here

Improve this question
$\endgroup$

2 Answers 2

Reset to default
14
$\begingroup$

Here is a conceptually very simple solution:

Observe that both a regular hexagon and a parallelogram will be cut in half by any line passing through their center of mass.

Therefore all we need to do is draw a line through the centers of mass. The centers of mass can be easily found by intersecting diagonals.
enter image description here

Improve this answer
$\endgroup$
3
  • $\begingroup$ Looks far more convincing than my answer (deleting it again then :-) $\endgroup$
    – xhienne
    Jan 22, 2021 at 22:39
  • 1
    $\begingroup$ It would be nice to have an image of this, but yeah, I think you've got it. $\endgroup$
    – Joel Rondeau
    Jan 22, 2021 at 22:52
  • $\begingroup$ Add a drawing and I approve the response. $\endgroup$
    – Moti
    Jan 23, 2021 at 2:30
-3
$\begingroup$

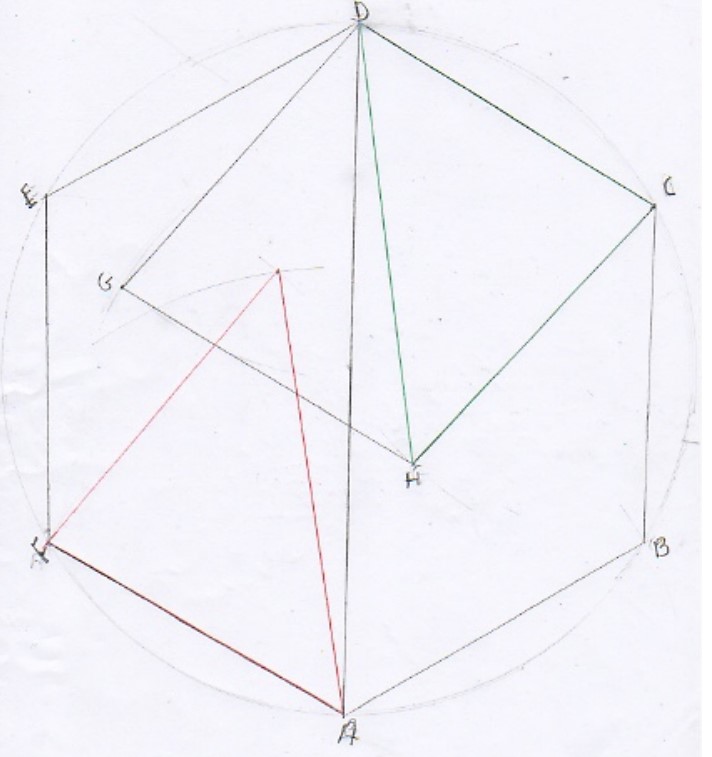
The question allows drawing of lines and points. In addition, the parallelogram is already cut out. If we draw the diagonal DH, the parallelogram is cut into two equal triangles, because in parallelograms the two opposite sides are always equal and both have the same third side DH. All vertices of a regular hexagon lie on the circumference. If we draw the straight line AD without doing any cutting, this line divides the hexagon into two equal areas because point D is opposite point A. We put the two triangles DHC and DHG as shown in the drawing. The areas of the two half hexagons which are not covered by the triangles are equal.

jan24

Improve this answer
$\endgroup$
3
  • 1
    $\begingroup$ From the question, the parallelogram is already cut out of the shape, so you can't cut it or move part of it. (In all fairness, this isn't made the most clear from the image provided.) Also, your solution requires two cuts (the parallelogram and the hexagon), but the question allows for one $\endgroup$
    – samm82
    Jan 24, 2021 at 6:08
  • $\begingroup$ I edited my answer to make everything clear. $\endgroup$
    – Vassilis Parassidis
    Jan 24, 2021 at 19:21
  • 1
    $\begingroup$ The task is to divide the octagon ABCHGDEF into two equal areas using a single straight line. This answer is incorrect because you modified the given octagon. (For drawing geometrical shapes, you can use something like GeoGebra.) $\endgroup$
    – Bubbler
    Jan 25, 2021 at 8:05

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge that you have read and understand our privacy policy and code of conduct.

Not the answer you're looking for? Browse other questions tagged or ask your own question.