I believe the minimum is about $\frac 12\sqrt[3]{6}\pi^{5/3}+\epsilon$$5.88$, whichand is about 6.12261393519866achieved by a Mylar balloon shape.
Let me introduce you to the magic of paper pleating:

The Nash-Kuiper embedding theorem $-$ well, a specific case of it, translated into English $-$ says that we can pleat paper into any shape, wasting as little paper as we want to. The only condition says that the paper can't get bigger. That is, if you imagine the paper as a fully stretched sheet of rubber, then the rubber can't stretch any more, it can only shrink. I recommend image searching "pleated paper"; it looks cool.
With that in mind, we have a hard math problem on our hands, from the calculus of variations. The Mylar balloon solves this problem (thanks Florian F), and looks like this (diagram from Wikipedia):
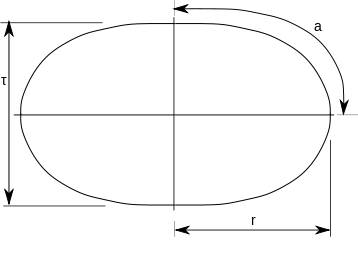
(To get the Mylar balloon, rotate this image around the vertical axis.)
To create your very own Mylar balloon, get a circle of paper of circumferenceradius $\frac 12\sqrt[3]{6}\pi^{5/3}+\epsilon$$a+\epsilon$, and pleatwhere $a$ is the arc length from the diagram. Pleat it into an approximation of a hemispherethe top half of circumference $\sqrt[3]{6}\pi^{2/3}+\epsilon$the Mylar balloon. Slice off a plane very close to the equator, so that the boundary of the remaining almostsquashed-hemisphere of paper lies on a plane. Reflect to get the other hemispherebottom half, and stitch along the boundary. Voila, a (very good approximation of a) sphereMylar balloon of volume 1.
Using the formulas in the Wikipedia page, we find that for a Mylar balloon of volume 1, each of the papers is a circle of radius $a \approx 0.936$. Therefore, the length of stitching required is $2\pi a\approx 5.88$.
