Hexagonal Kurokuron

Question

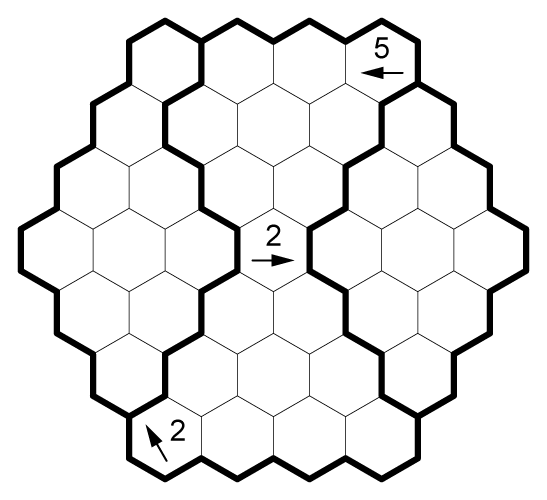
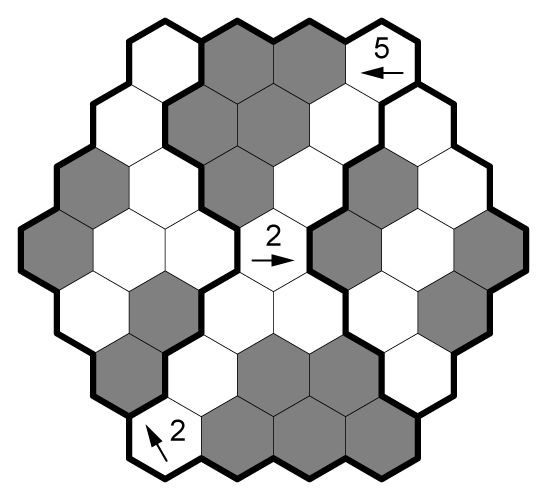
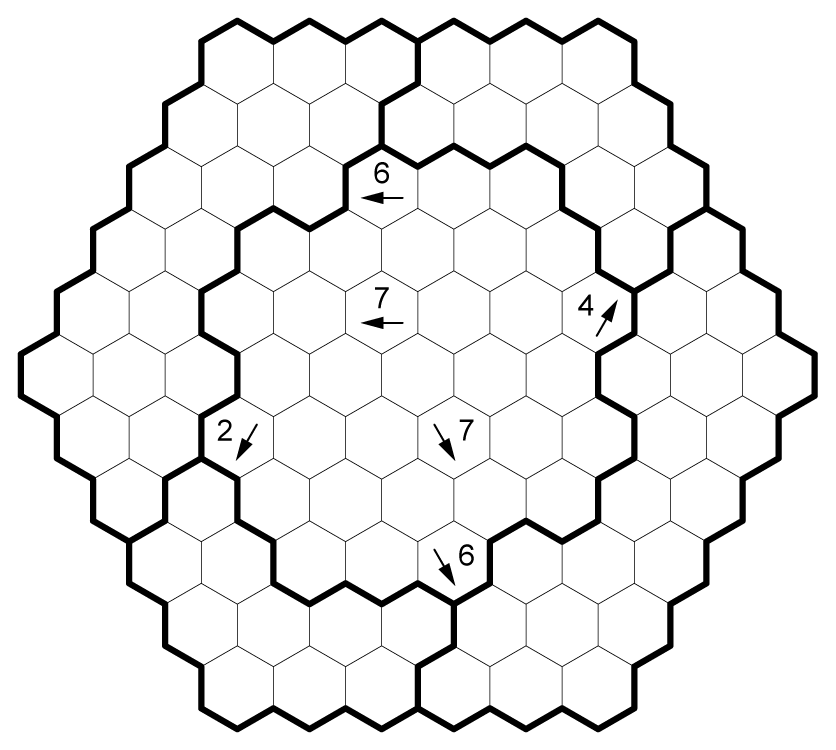
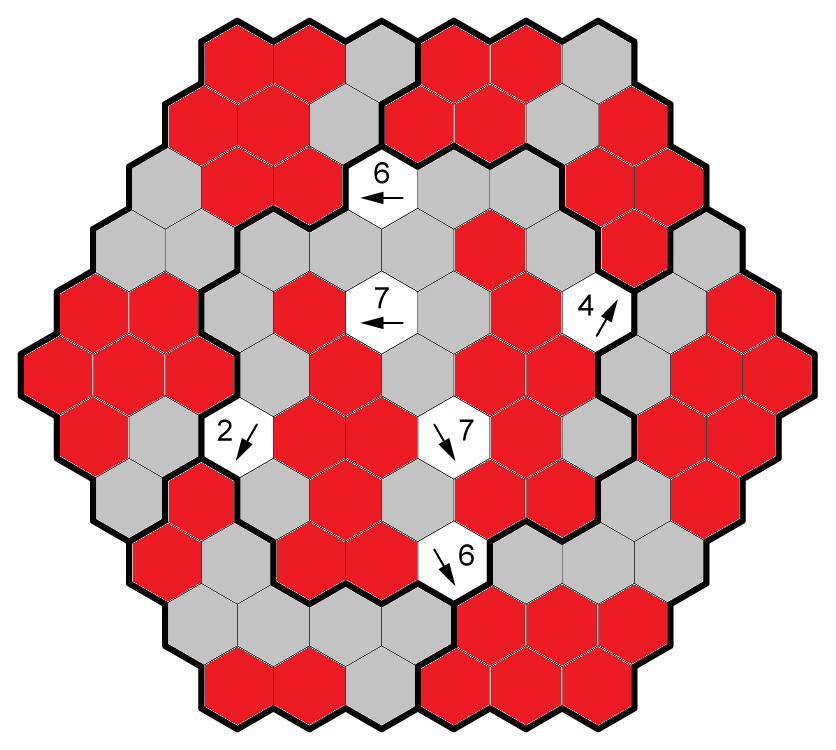
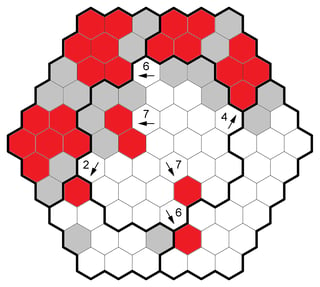
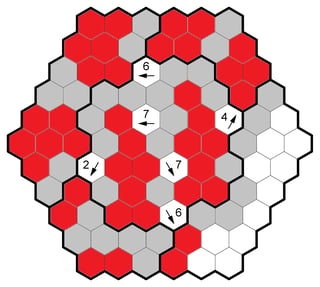
Kurokuron (クロクロ一ン: "black clone"), is a shading puzzle that first appeared in Puzzle Communication Nikoli issues 153-155. Identical rules apply to a hexagonal grid.

Rules

Shade some cells such that:

1. Bold-outlined regions contain exactly two shapes, made up of contiguous groups of 1 or more shaded cells. Within a region, the two shapes must be congruent, allowing reflection and rotation.
2. A shape cannot share an edge with another shape.
3. Cells with arrows, which cannot be shaded, point to a neighboring cell, which must be shaded and part of a shape consisting of the given number of cells.

Example

Puzzle

Answer 1

Final grid

(red is shaded, gray is not shaded)

Explanation (Potential spoilers!)

Note that there really is one possibility to fit two regions into the 4 region (all other ways to put two 4-shapes cause them to touch). The same applies to the 6 region in the upper left corner:

From here, I figured out that if the puzzle were to have a unique solution, then the two shapes in the 2 region had to not touch other regions as much as possible, leading to this:

From here there is only one way to fill the central 7 region, and the 6 region follows easily.