24
$\begingroup$

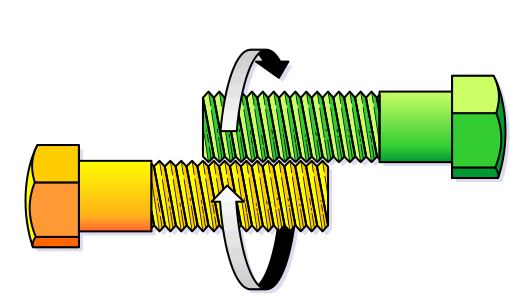
This is a puzzle of Martin Gardner, taken verbatim from My Best Mathematical and Logic Puzzles:

Two identical bolts are placed together so their grooves intermesh. If you move the bolts around each other as you would twiddle your thumbs, holding each bolt firmly by the head so it does not rotate and twiddling them in the direction shown below, will the heads
(a) move inward,
(b) move outward, or
(c) remain the same distance from each other? enter image description here

Image source: https://www.theguardian.com/science/alexs-adventures-in-numberland/2014/oct/27/solutions-to-martin-gardners-best-mathematical-puzzles.

I got the correct answer, though I couldn't really intuitively grasp why it was correct. The book offers the following answer and explanation (mouse over for spoilers):

The answer is (c): the situation is comparable to a person walking up an escalator at the same rate it is moving down.

The top google results for this puzzle pretty much quote this solution without further comment. Perhaps I'm just dense, Gardner's answer doesn't really feel like an explanation. Therefore, I've posted this problem for two reasons:

  1. To give people who haven't seen this puzzle a chance to enjoy it, and

  2. To get some satisfying explanations which make me say, "Oh, of course that's why that answer correct!".

Improve this question
$\endgroup$
4
  • $\begingroup$ The double-start thread threw me off. $\endgroup$
    – Bergi
    Commented Jun 7, 2017 at 13:06
  • $\begingroup$ I don't think those grooves can intermesh from two identical bolts. You'd need a left-handed and a right-handed thread. $\endgroup$
    – Bergi
    Commented Jun 7, 2017 at 13:08
  • $\begingroup$ @Bergi - two identical bolts can indeed intermesh in the way shown. The good thing about this puzzle is that you can actually try it for yourself. Grab a couple of bolts and give it a go. $\endgroup$
    – Tim
    Commented Jun 8, 2017 at 2:16
  • $\begingroup$ I actually did this and filmed it: Youtube $\endgroup$
    – user2633613
    Commented Jun 12, 2018 at 8:37

4 Answers 4

Reset to default
19
$\begingroup$

The bolts are identical - they both follow the "righty tighty lefty loosy" rule.

Now look at the twiddling movement, first from one side, then the other. From one side the movement is clockwise, but viewed from the opposite side it must then be anti-clockwise. Therefore, as one bolt tightens, the other loosens, resulting in no effect.

To push the point home, looking from the opposite side you will see the same as if everything were going in reverse. So if they were moving towards each other, then merely looking at it from the opposite side would make them go in reverse and then must make them move apart. This is obviously impossible, so the bolts can't be moving towards each other.

Improve this answer
$\endgroup$
2
  • 5
    $\begingroup$ when i'm next using a screwdriver, i'll remember the 'righty tighty lefty loosy' rule - it should save me some time! $\endgroup$
    – JMP
    Commented Jun 7, 2017 at 6:08
  • 1
    $\begingroup$ I think looking from the other side is the most satisfying explanation so far. $\endgroup$
    – Neil
    Commented Jun 7, 2017 at 8:54
7
$\begingroup$

Deep chiral explanation:

There are 4 possible rotations, one moves the bolts together, another moves them apart, two have no action. Owing to the symmetry of the rotations given, no movement,

Improve this answer
$\endgroup$
6
$\begingroup$

The top screw is being

Unscrewed by the movement of the bottom one around it (its external environment is moving clockwise around it from its point of view).

The bottom screw is being

Screwed in by the movement of the top one around it (it's external environment is moving anti-/counter-clockwise around it from its point of view).

So

the top one is trying to move away from the bottom one at the same rate as the bottom one is trying to move towards the top one.

By symmetry

neither can "win", so they cancel each other out and stay the same distance apart.

Improve this answer
$\endgroup$
3
$\begingroup$

The top one will be screwed, which will move it from one distance x to the other.
As for the other, the movement will cause it to be unscrewed, which will cause it to retreat by the same distance x from the first.
There will therefore be a general translation to the left without any real change of distance between the two.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.