19
$\begingroup$

Inspired by Fitting the 9th piece into the pizza box

2 pizzas with radius r are each cut into 8 identical slices. 6 pieces were eaten so there are 10 pieces left. They need to be put in a square box without cutting or overlapping pieces. What is the minimal side length of the box, expressed in r?

Improve this question
$\endgroup$
3
  • 2
    $\begingroup$ The $m=4,n=5$ entry here yields a packing with side length $2.081r$: erich-friedman.github.io/mathmagic/0112.html $\endgroup$
    – RobPratt
    Commented Aug 23 at 3:25
  • 1
    $\begingroup$ @RobPratt some cool packings on that page. $\endgroup$
    – Dmitry Kamenetsky
    Commented Aug 23 at 5:06
  • $\begingroup$ 2r, because sane people will put the extras in a zip-top bag. 😉 (Or feed them to the dog, as in the preceding question.) $\endgroup$
    – Matthew
    Commented Aug 25 at 4:57

3 Answers 3

Reset to default
21
$\begingroup$

Thanks to 2012rcampion for crunching the numbers to find a minimum side length of

$r\times 2.064\,821\,224\,090\,113\,066\,623\,307\,255\,407\ldots$

For this arrangement of slices:

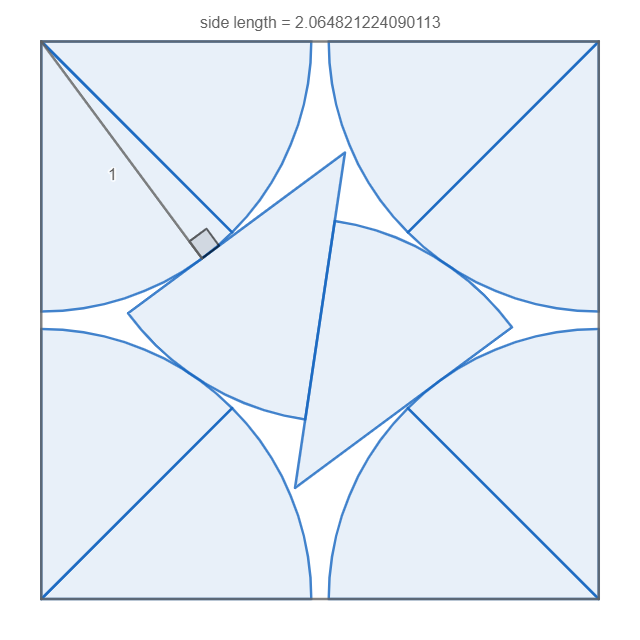
Two in each corner, two in the center

Improve this answer
$\endgroup$
2
  • $\begingroup$ @2012rcampion I believe you are correct. I have no idea how to derive that, but it certainly works. Thank you. $\endgroup$
    – Daniel Mathias
    Commented Aug 23 at 1:06
  • $\begingroup$ I don't know the actual answer, but since yours is the lowest of all answers I accepted it. $\endgroup$
    – Ivo
    Commented Aug 23 at 6:02
18
$\begingroup$

Here is a simple but rather effective packing:

symmetric packing

The sides of the square box are

$\frac {1+\sqrt 2 + \sqrt 3} 2 r \approx 2.073 r$

Explanation:

Let $x$ be the distance between the bottom left corner and the tip $T$ of the lone slice on the left. The distance of $T$ to the top left corner is $\sqrt 3$ because these two points together with the centre of the upper half-pizza form a 30-60-90 triangle. The side of the square is therefore $s = x+\sqrt3$.

Now extend the diagonal side of the lone slice on the left and the base of the square up to their intersection. The extended base has length $1+\sqrt 2$, the extension itself has length $x$. Therefore $s = 1+\sqrt 2-x$. The formula can now be obtained by adding the two expressions for the side length.

Improve this answer
$\endgroup$
6
  • 4
    $\begingroup$ I think you should put a square root on the 1 in your formula. It's totally unnecessary but it would definitely look cooler. $\endgroup$
    – Michael Seifert
    Commented Aug 22 at 19:31
  • 7
    $\begingroup$ @MichaelSeifert Why stop there? How would you like $(\sqrt 1 + \sqrt 2 +\sqrt 3) / \sqrt 4$? $\endgroup$
    – Albert.Lang
    Commented Aug 23 at 3:02
  • 1
    $\begingroup$ @Albert.Lang I like the way you think $\endgroup$
    – Michael Seifert
    Commented Aug 23 at 11:30
  • $\begingroup$ Understanding the proof is a puzzle in its own right, haha. But I got it now. Needed some time to see which triangles you used to derive the 1+sqrt(2) $\endgroup$
    – justhalf
    Commented Aug 23 at 21:46
  • $\begingroup$ I don't understand how you come to the assumption of a 30-60-90 triangle $\endgroup$
    – kopaka
    Commented Aug 27 at 12:28
7
$\begingroup$

Here's the best setup I could come up with, using unit slices:

Pizza packing optimized

Achieving a length of

$s = 2.137580020435572137..$

Here, the highlighted slice is shifted by $b \approx 0.01626$ in both directions, and the rest of the pieces fit.

This value of $b$ is optimal for this configuration, and the resulting side length turns out to be algebraic :

Let $p_{\pm} = 284+49\sqrt2 + 13\sqrt[3]{164662-2705\sqrt{2} \pm 225 \sqrt{3 \left(62135-39834 \sqrt{2}\right)}}$. Then $${s=\hspace{3px}} \frac{1}{390} \left(474+153 \sqrt{2}+\sqrt{6 (p_{-}+p_{+})}+\sqrt{6 \left(1704+294 \sqrt{2}-p_{-}-p_{+} -8700 \sqrt{\frac{3}{p_{-}+p_{+}}}+9000 \sqrt{\frac{6}{p_{-}+p_{+}}}\right)}\right)$$ and $$b = s - \frac{3 \sqrt{2}}{2}$$

Improve this answer
$\endgroup$
4
  • 2
    $\begingroup$ This is excellent work! I have come to the conclusion that pizza makers should make slightly bigger pizza boxes so we can fit in more pizza slices :) $\endgroup$
    – Dmitry Kamenetsky
    Commented Aug 21 at 12:46
  • 1
    $\begingroup$ @DmitryKamenetsky ledopizza.com/why-is-ledo-pizza-square "round pans have become the rage as pizza operators are able to cut costs by giving the appearance of more, while actually offering less." $\endgroup$
    – RobPratt
    Commented Aug 21 at 16:08
  • 1
    $\begingroup$ I don't think square pizzas will ever become preferred Italian standard, only if not because classically throwing them turning around to get correct proportions won't result in a square. :-) $\endgroup$
    – FirstName LastName
    Commented Aug 22 at 0:51
  • 3
    $\begingroup$ Square pizzas used to be the standard at a pizza place up on Basingstoke Road in Reading opposite Morrisons some few decades ago. I can't remember its name. Best pizzas ever and they were very popular. For the size of box you got (obviously) far more pizza than a circular one. Don't know why they stopped trading because they were the absolute best. $\endgroup$
    – Prime Mover
    Commented Aug 22 at 9:55

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.